この記事では、三角関数の角度の求め方や変換公式(\(90^\circ − \theta\) など)についてわかりやすく解説していきます。
三角方程式・三角不等式の計算問題もていねいに説明していくので、この記事を通してぜひマスターしてくださいね!
三角関数の角度の求め方
例題を通して、三角関数の角度の求め方を説明します。
\(\displaystyle \sin \theta = \frac{\sqrt{3}}{2}\) のとき \(\theta\) を求めなさい。(\(0 \leq \theta \leq 2\pi\))
このように、三角関数を含む方程式を「三角方程式」と呼びます。
三角方程式を解いて角度を求める際は、単位円を用います。
まず、求める \(\theta\) の範囲を確認します。
今回は \(0 \leq \theta \leq 2\pi\) と設定されているので、単位円 \(1\) 周分を考えます(→ 補足① 単位円と三角比の関係)。
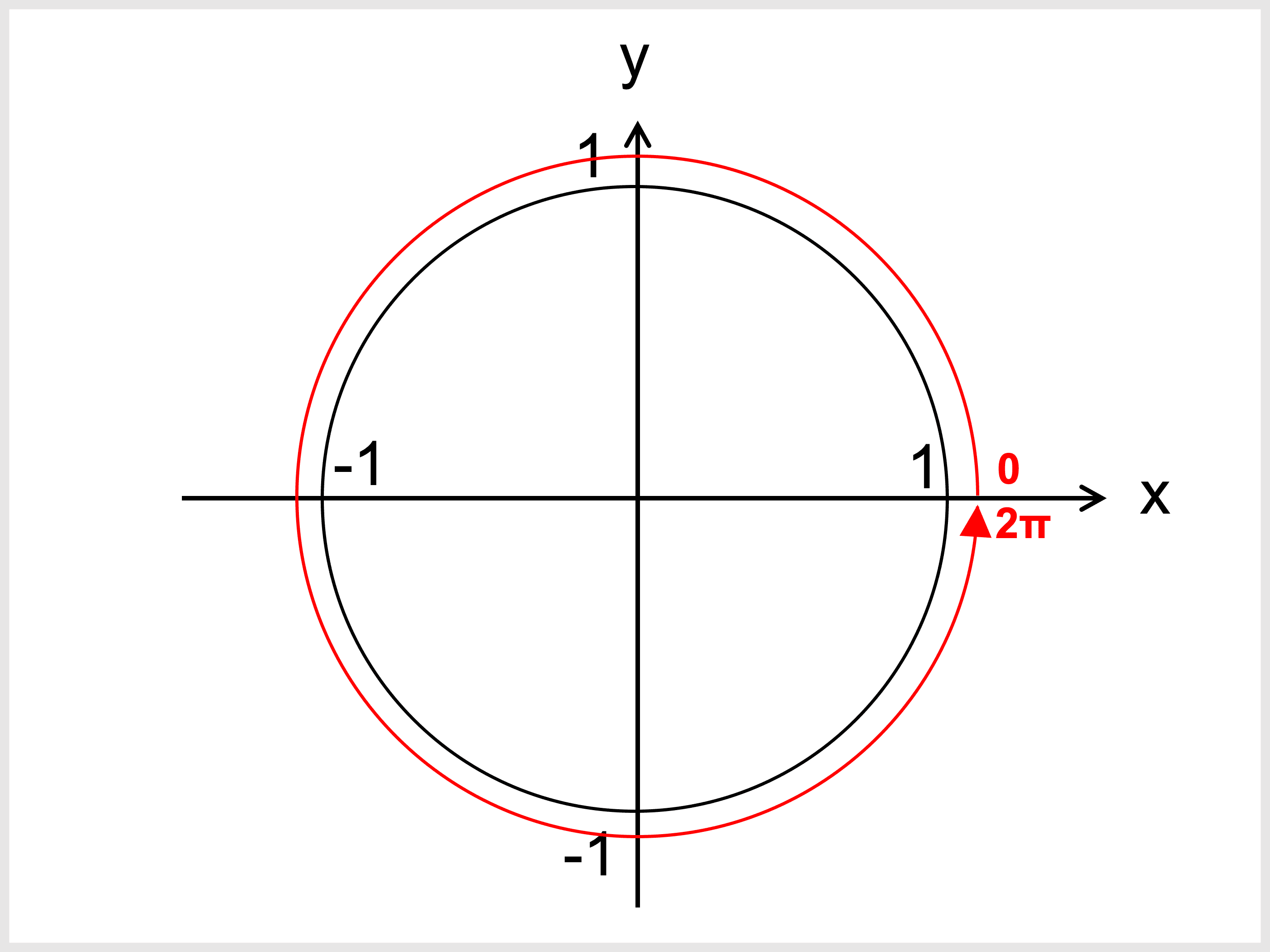
与えられた条件を単位円に記入しましょう。
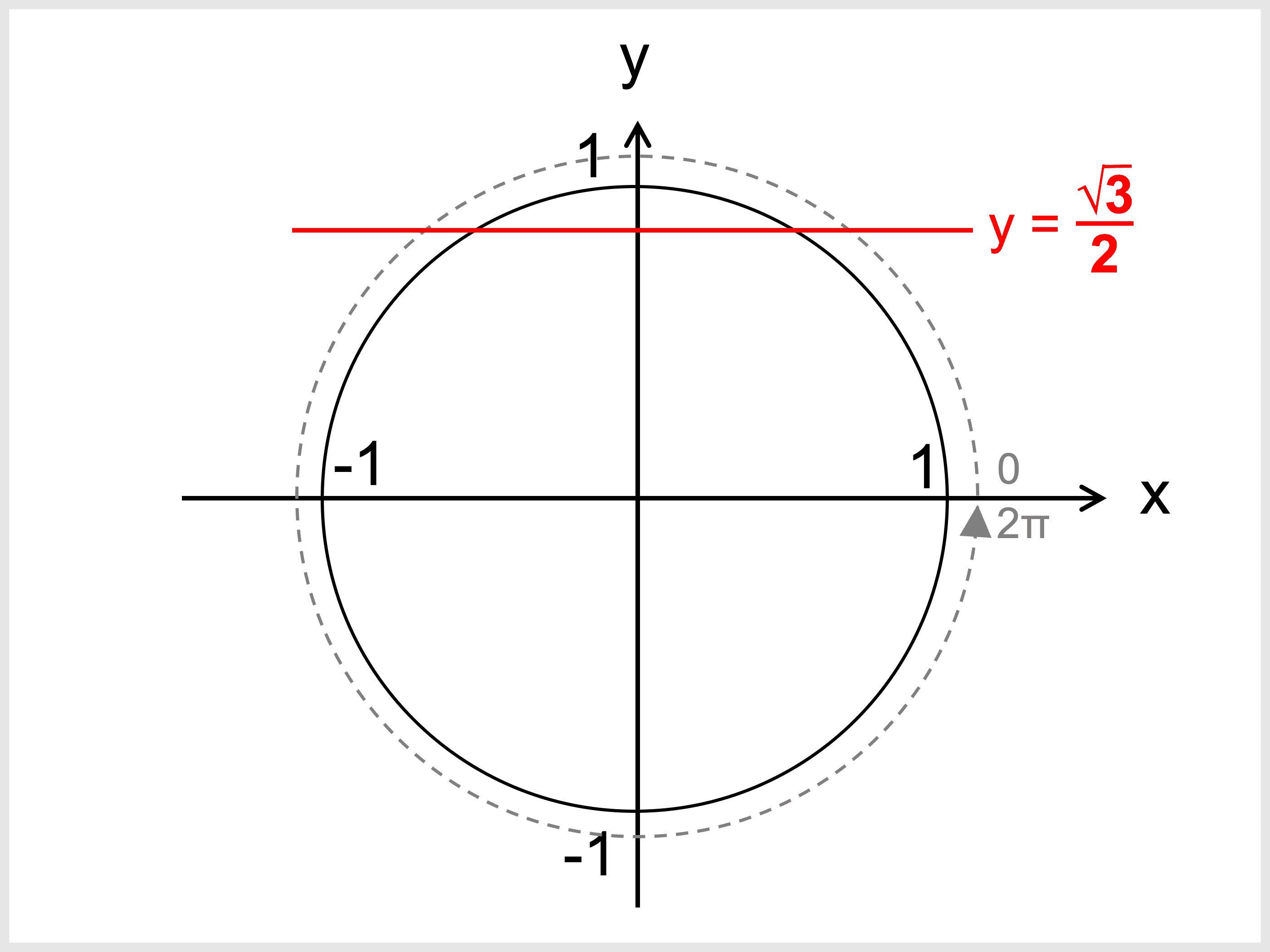
今回は \(\displaystyle \sin \theta = \frac{\sqrt{3}}{2}\) なので、\(\displaystyle y = \frac{\sqrt{3}}{2}\) の直線を引きます。
\(\displaystyle \frac{\sqrt{3}}{2}\), \(\displaystyle \frac{1}{2}\), \(\displaystyle \frac{1}{\sqrt{2}}\) の長さの感覚は、暗記した直角三角形とともに身につけておきましょう(→ 補足② 暗記すべき直角三角形)。
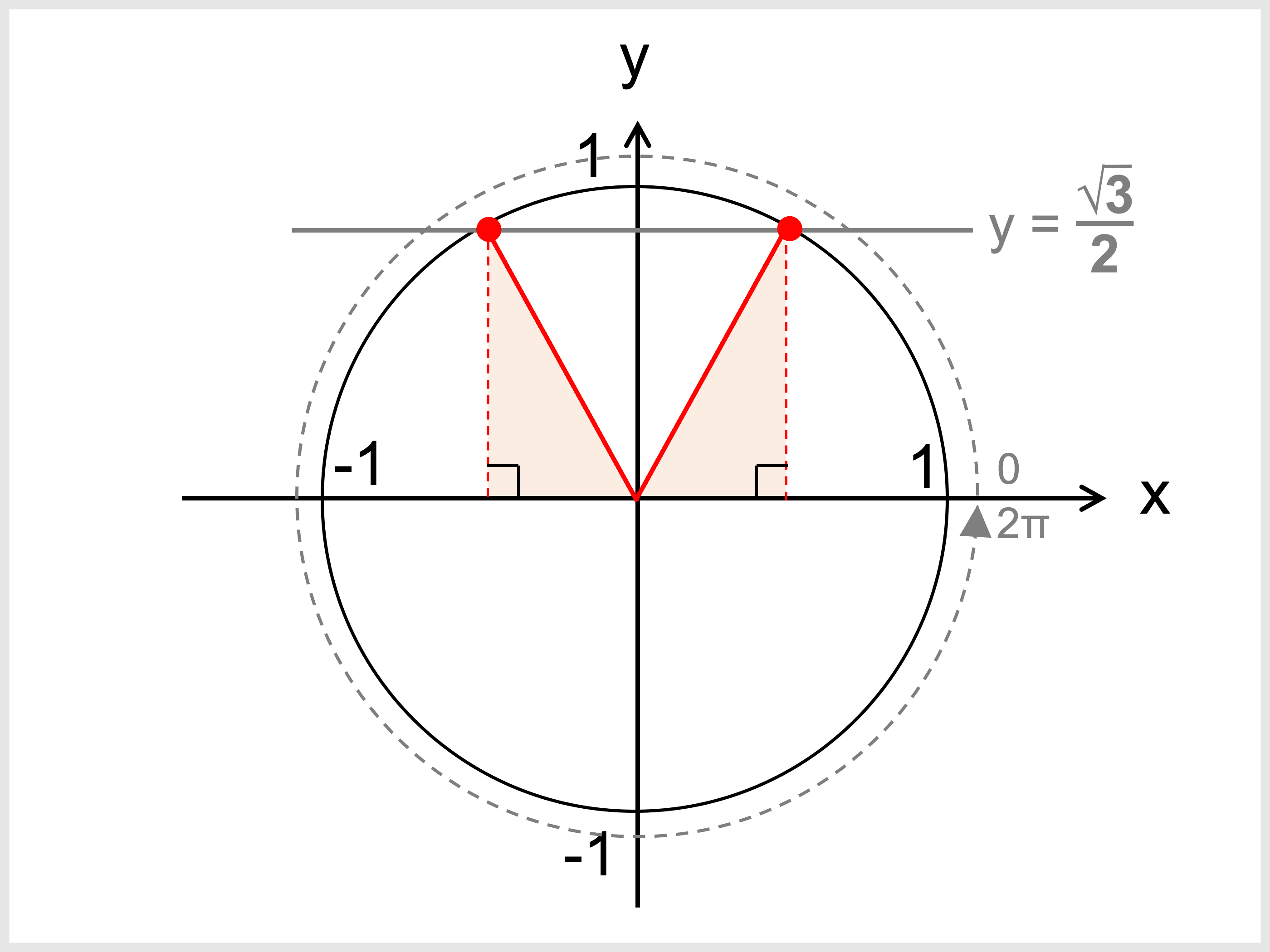
先ほどの直線と単位円の交点を原点と結び、動径を得ます。
また、その交点から \(x\) 軸に垂線を下ろして直角三角形を作ります。
今回の直角三角形は、辺の比が \(\displaystyle \frac{1}{2} : 1 : \frac{\sqrt{3}}{2}\) のものですね。
よって、\(x\) 軸となす角が \(\displaystyle \frac{\pi}{3}\) \((= 60^\circ)\) の直角三角形とわかります。
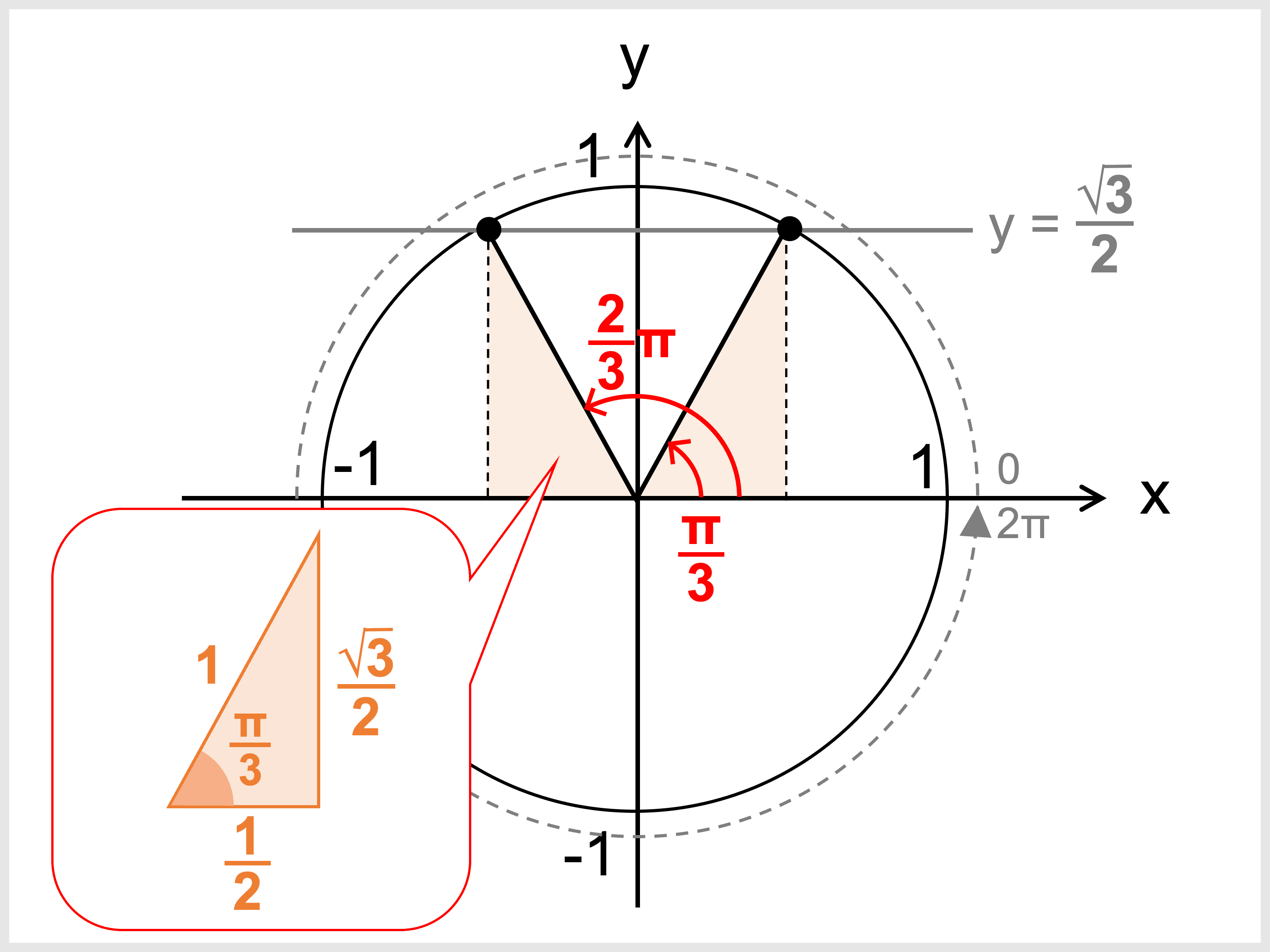
始線からの動径の角度は、
- \(\displaystyle \frac{\pi}{3}\)
- \(\displaystyle \pi − \frac{\pi}{3} = \frac{2}{3} \pi\)
ですね。
よって答えは \(\color{red}{\displaystyle \theta = \frac{\pi}{3}, \frac{2}{3} \pi}\) です。
このように、三角関数の角度は単位円に条件を書き込んでいくだけで求められます。
角度の範囲や値の条件を見落とさないように注意しましょう!
補足① 単位円と三角比の関係
単位円とは半径 \(1\) の円のことで、\(1\) 周分の角度は \(\color{red}{360^\circ}\)(度数法)または \(\color{red}{2\pi}\) (弧度法)です。
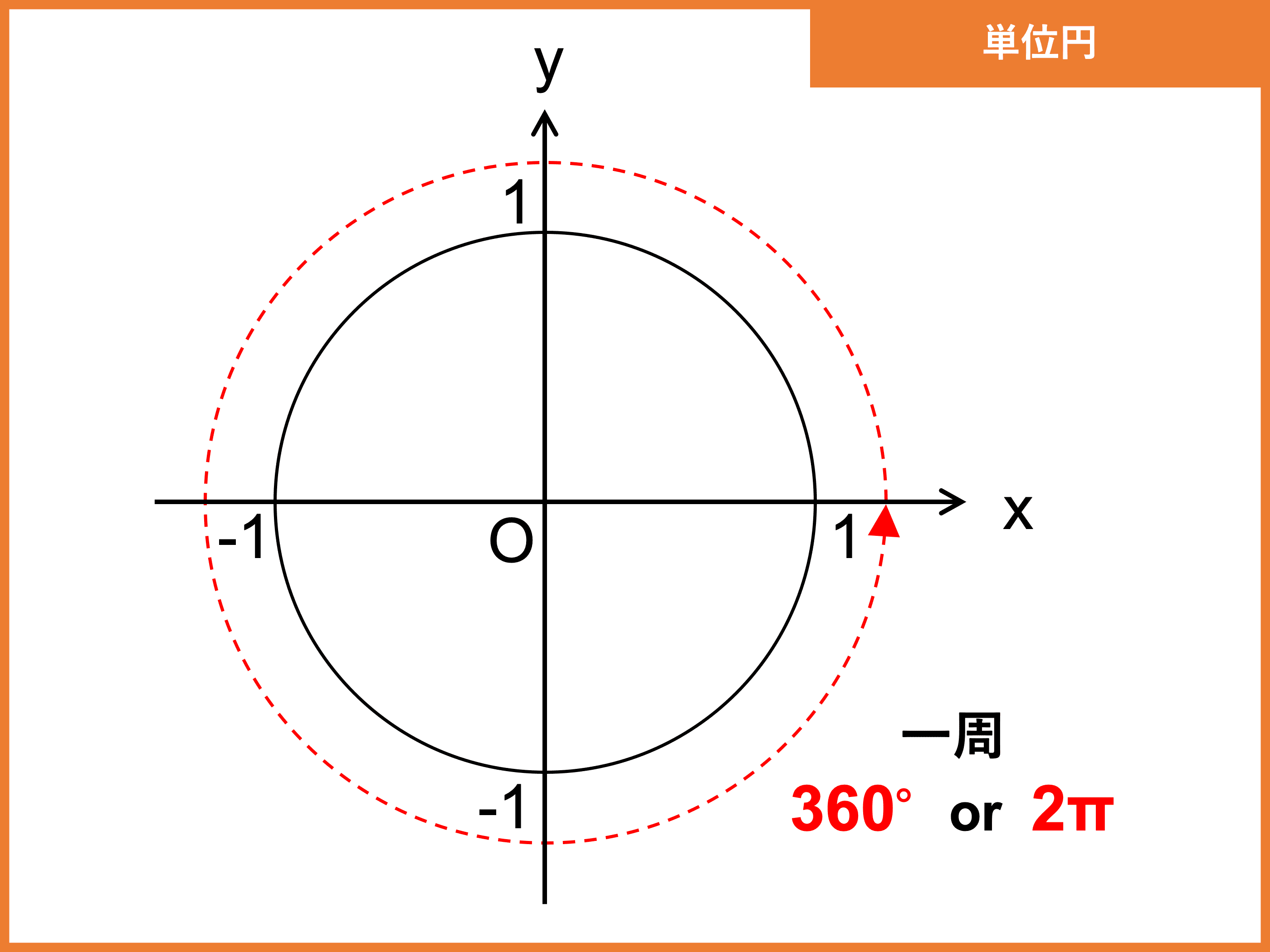
単位円上に点 \(\mathrm{P}(x, y)\) をとり、動径 \(\mathrm{OP}\) が \(x\) 軸となす角を \(\theta\) とすると、三角比の値は次のようになります。
- \(\cos \theta = x\) → 「\(x\) 座標の値」
- \(\sin \theta = y\) → 「\(y\) 座標の値」
- \(\displaystyle \tan \theta = \frac{y}{x}\) → 「動径の傾きの値」(\(x = 1\) のときの \(y\) の値)
「\(\cos\) は横軸、\(\sin\) は縦軸、\(\tan\) は傾き」を見ると覚えておきましょう。

補足② 暗記すべき直角三角形
主要な角度の三角比をスムーズに求めるために、次の \(2\) つの直角三角形を暗記しておきましょう。

辺の長さは斜辺を \(1\) として、角度は度数法・弧度法の両方で覚えておくのがポイントです。
これだけ覚えておけば、あとは単位円の中に適切な直角三角形を配置するだけで、主要な角度の三角比を求めることができます。
三角関数の変換公式
三角関数の角度の変換公式(\(90^\circ \pm \theta\), \(180^\circ \pm \theta\), \(−\theta\) の変換公式)を示します。
これらの公式は丸暗記する必要はなく、単位円を使って自分で確認できればOKです!
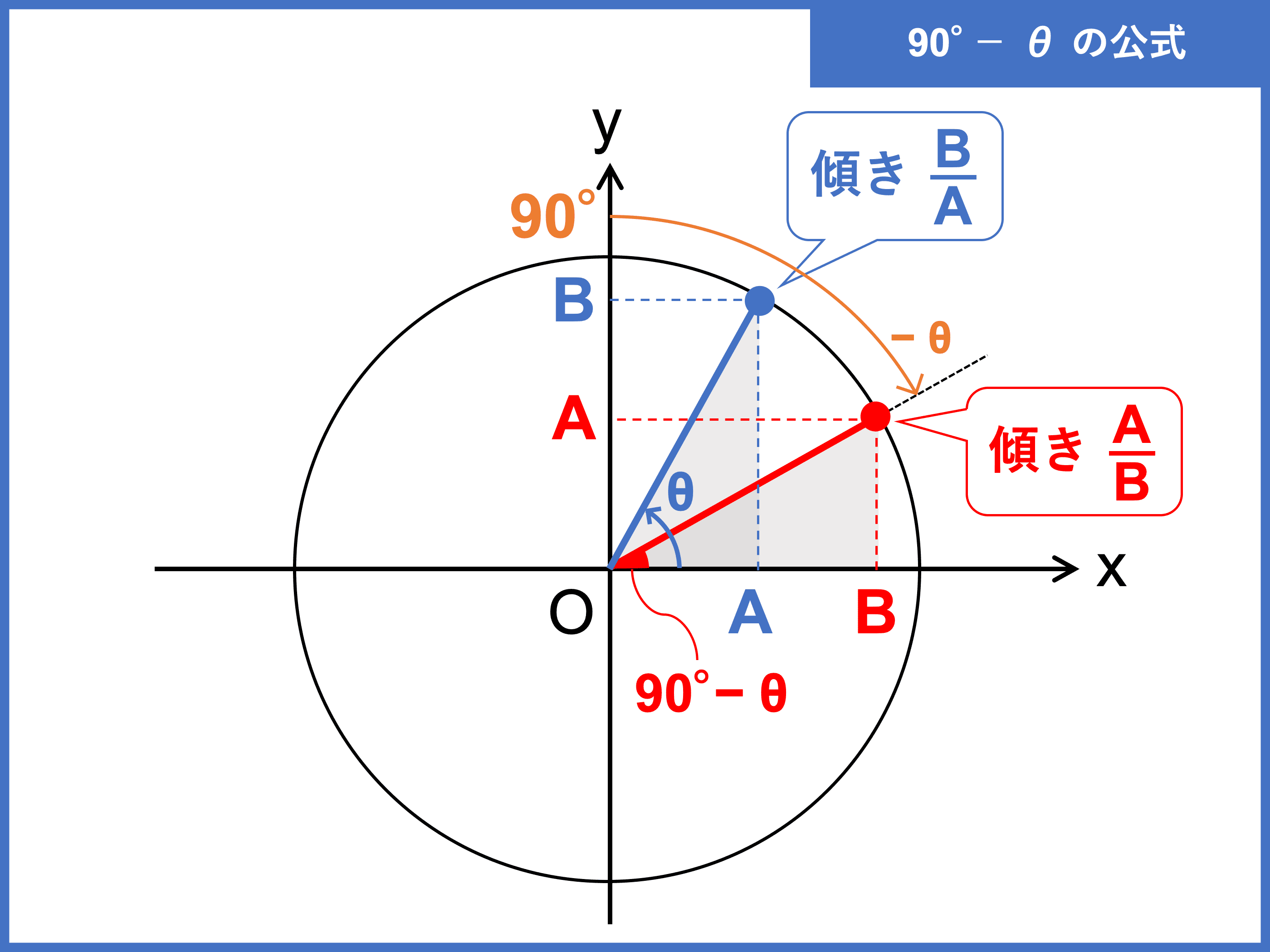
\(90^\circ − \theta \left(\displaystyle \frac{\pi}{2} − \theta \right)\) の変換公式
【度数】
- \(\color{red}{\sin (90^\circ − \theta) = \cos \theta}\)
- \(\color{red}{\cos (90^\circ − \theta) = \sin \theta}\)
- \(\color{red}{\tan (90^\circ − \theta) = \displaystyle \frac{1}{\tan \theta}}\)
【弧度 (ラジアン)】
- \(\color{red}{\displaystyle \sin \left( \frac{\pi}{2} − \theta \right) = \cos \theta}\)
- \(\color{red}{\displaystyle \cos \left( \frac{\pi}{2} − \theta \right) = \sin \theta}\)
- \(\color{red}{\displaystyle \tan \left( \frac{\pi}{2} − \theta \right) = \frac{1}{\tan \theta}}\)
\(90^\circ + \theta \left(\displaystyle \frac{\pi}{2} + \theta \right)\) の変換公式
【度数】
- \(\color{red}{\sin (90^\circ + \theta) = \cos \theta}\)
- \(\color{red}{\cos (90^\circ + \theta) = −\sin \theta}\)
- \(\color{red}{\tan (90^\circ + \theta) = −\displaystyle \frac{1}{\tan \theta}}\)
【弧度 (ラジアン)】
- \(\color{red}{\displaystyle \sin \left( \frac{\pi}{2} + \theta \right) = \cos \theta}\)
- \(\color{red}{\displaystyle \cos \left( \frac{\pi}{2} + \theta \right) = −\sin \theta}\)
- \(\color{red}{\displaystyle \tan \left( \frac{\pi}{2} + \theta \right) = −\frac{1}{\tan \theta}}\)

\(180^\circ − \theta \left(\pi − \theta \right)\) の変換公式
【度数】
- \(\color{red}{\sin (180^\circ − \theta) = \sin \theta}\)
- \(\color{red}{\cos (180^\circ − \theta) = −\cos \theta}\)
- \(\color{red}{\tan (180^\circ − \theta) = −\tan \theta}\)
【弧度 (ラジアン)】
- \(\color{red}{\sin (\pi − \theta) = \sin \theta}\)
- \(\color{red}{\cos (\pi − \theta) = −\cos \theta}\)
- \(\color{red}{\tan (\pi − \theta) = −\tan \theta}\)

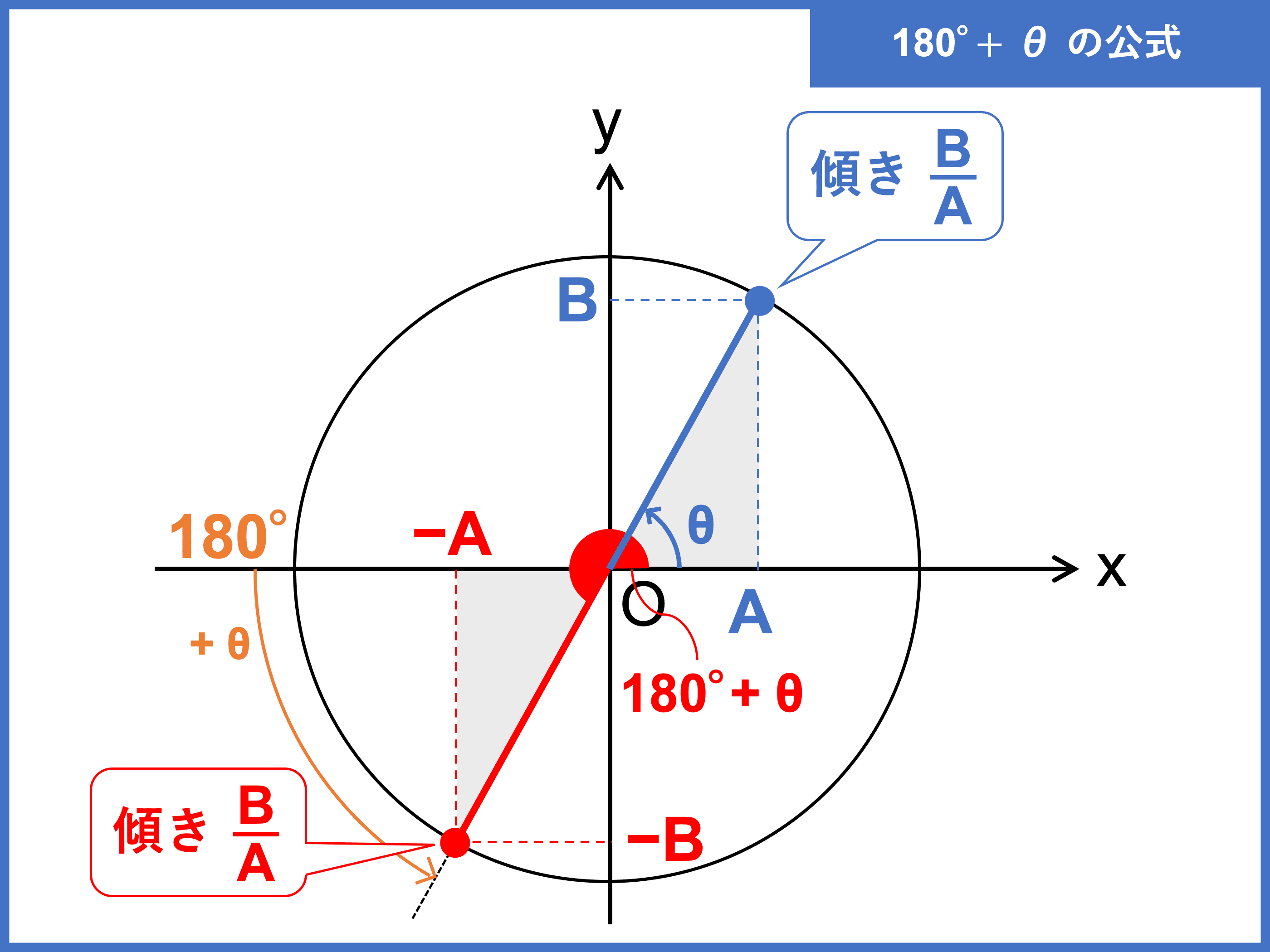
\(180^\circ + \theta \left(\pi + \theta \right)\) の変換公式
【度数】
- \(\color{red}{\sin (180^\circ + \theta) = −\sin \theta}\)
- \(\color{red}{\cos (180^\circ + \theta) = −\cos \theta}\)
- \(\color{red}{\tan (180^\circ + \theta) = \tan \theta}\)
【弧度 (ラジアン)】
- \(\color{red}{\sin (\pi + \theta) = −\sin \theta}\)
- \(\color{red}{\cos (\pi + \theta) = −\cos \theta}\)
- \(\color{red}{\tan (\pi + \theta) = \tan \theta}\)
\(− \theta\) の変換公式
【度数】
- \(\color{red}{\sin (−\theta) = −\sin \theta}\)
- \(\color{red}{\cos (−\theta) = \cos \theta}\)
- \(\color{red}{\tan (−\theta) = −\tan \theta}\)
【弧度 (ラジアン)】
- \(\color{red}{\sin (−\theta) = −\sin \theta}\)
- \(\color{red}{\cos (−\theta) = \cos \theta}\)
- \(\color{red}{\tan (−\theta) = −\tan \theta}\)
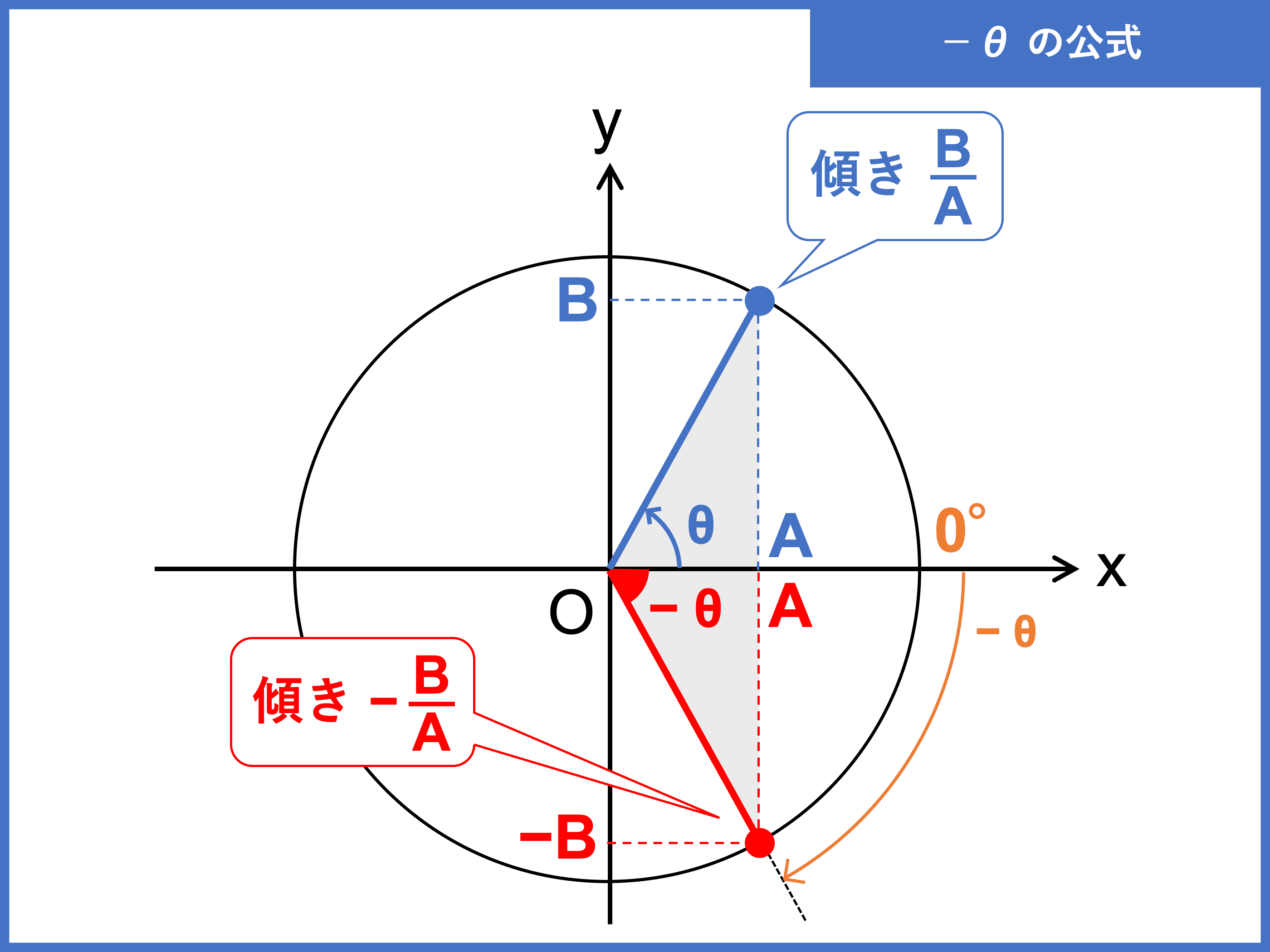
単位円で見ると、どれも同じ形の直角三角形を違う場所に配置しただけというのがわかりますね。
このように、変換公式を使うときは実際に単位円を書いて値や符号の関係を確かめるのが一番確実です。
ちなみに、どの変換公式も「加法定理」を使えば証明できます。
 加法定理とは?覚え方や証明、応用問題をわかりやすく解説
加法定理とは?覚え方や証明、応用問題をわかりやすく解説
三角関数の角度の計算問題
それでは、三角関数の角度に関する計算問題を解いてみましょう!
計算問題①「三角方程式 \(\displaystyle 2\cos \theta + 1 = 0\)」
\(\displaystyle 2\cos \theta + 1 = 0\) のとき \(\theta\) を求めなさい。ただし、\(0 \leq \theta \leq 3\pi\) とする。

まずは、三角方程式が「\((\text{三角比}) = (\text{数値})\)」となるように式変形します。
単位円上に動径を図示するときは、\(\theta\) のとりうる範囲に注意しましょう。
\(\displaystyle 2\cos \theta + 1 = 0\) より
\(\displaystyle 2\cos \theta = −1\)
\(\displaystyle \cos \theta = −\frac{1}{2}\)
\(0 \leq \theta \leq 3\pi\) において、\(\displaystyle \cos \theta = −\frac{1}{2}\) を満たす動径は
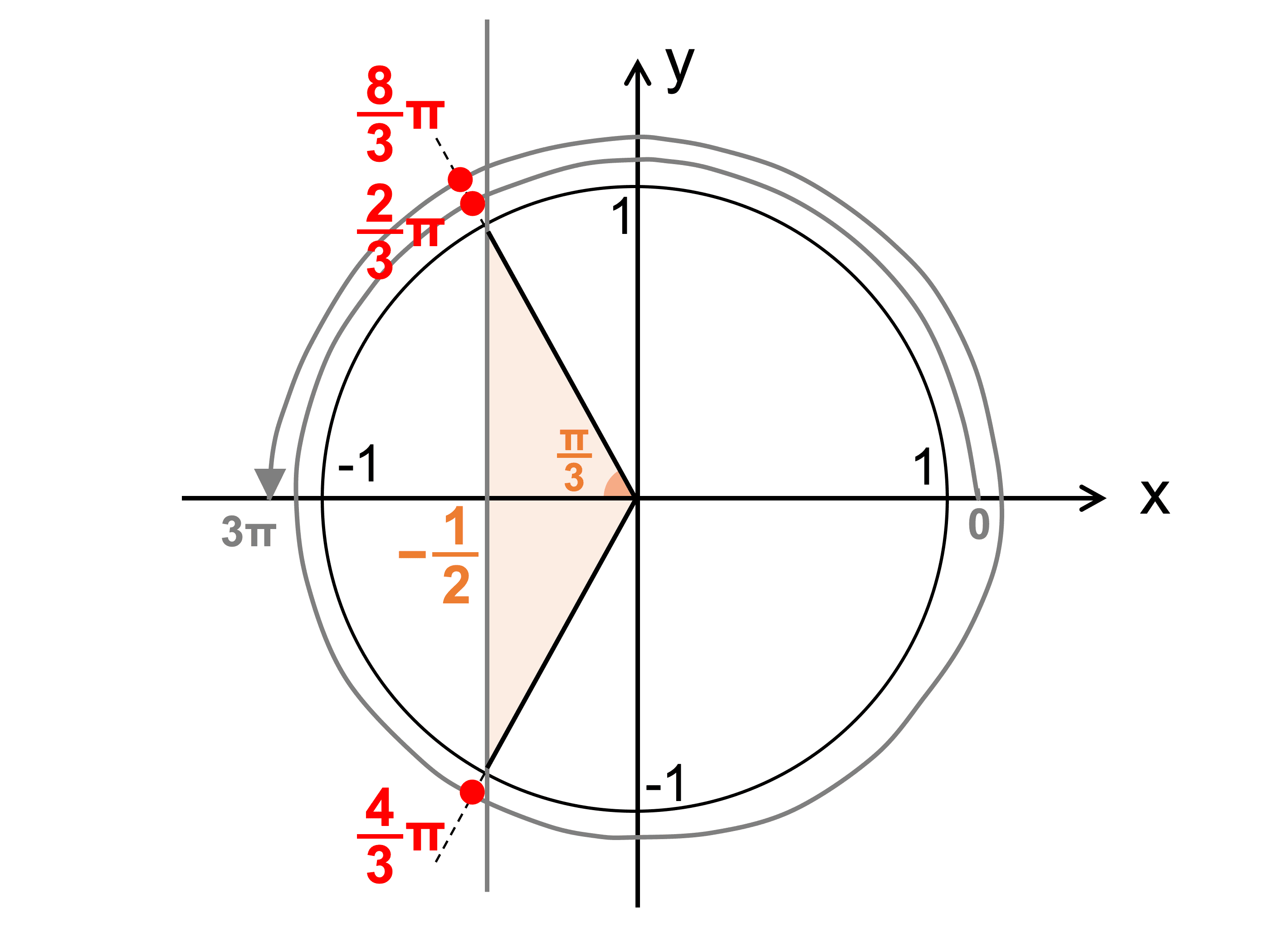
よって、\(\displaystyle \theta = \frac{2}{3}\pi, \frac{4}{3}\pi, \frac{8}{3}\pi\)
答え: \(\color{red}{\displaystyle \theta = \frac{2}{3} \pi, \frac{4}{3} \pi, \frac{8}{3}\pi}\)
計算問題②「三角方程式 \(\displaystyle \tan \theta = −1\)」
\(\displaystyle \tan \theta = −1\) のとき \(\theta\) を求めなさい。ただし、\(\displaystyle \frac{\pi}{2} \leq \theta \leq \frac{7}{2} \pi\) とする。

\(\displaystyle \tan \theta\) なので、傾きを考えます。この問題も \(\theta\) の範囲に注意です!
\(\displaystyle \frac{\pi}{2} \leq \theta \leq \frac{7}{2} \pi\) において、\(\displaystyle \tan \theta = −1\) を満たす動径は

よって、\(\displaystyle \theta = \frac{3}{4}\pi, \frac{7}{4}\pi, \frac{11}{4}\pi\)
答え: \(\color{red}{\displaystyle \theta = \frac{3}{4}\pi, \frac{7}{4}\pi, \frac{11}{4}\pi}\)
計算問題③「三角不等式 \(\displaystyle 2\cos^2 \theta − \sin \theta − 2 \geq 0\)」
\(0 \leq \theta \leq 2\pi\) のとき、不等式 \(\displaystyle 2\cos^2 \theta − \sin \theta − 2 \geq 0\) を解け。

三角関数を含む不等式を「三角不等式」といいます。
式変形して三角比を \(1\) 種類に絞り、単位円でとりうる値の範囲を図示して \(\theta\) の範囲を求めます。
\(\displaystyle 2\cos^2 \theta − \sin \theta − 2 \geq 0\) において、三角比の相互関係より
\(\displaystyle 2(1 − \sin^2 \theta) − \sin \theta − 2 \geq 0\)
\(\displaystyle 2 − 2\sin^2 \theta − \sin \theta − 2 \geq 0\)
\(\displaystyle − 2\sin^2 \theta − \sin \theta \geq 0\)
\(\displaystyle 2\sin^2 \theta + \sin \theta \leq 0\)
\(\displaystyle 2\sin \theta \left(\sin \theta + \frac{1}{2}\right) \leq 0\)
よって \(−\displaystyle \frac{1}{2} \leq \sin \theta \leq 0\)
\(0 \leq \theta \leq 2\pi\) において、\(−\displaystyle \frac{1}{2} \leq \sin \theta \leq 0\) を満たす動径の範囲は
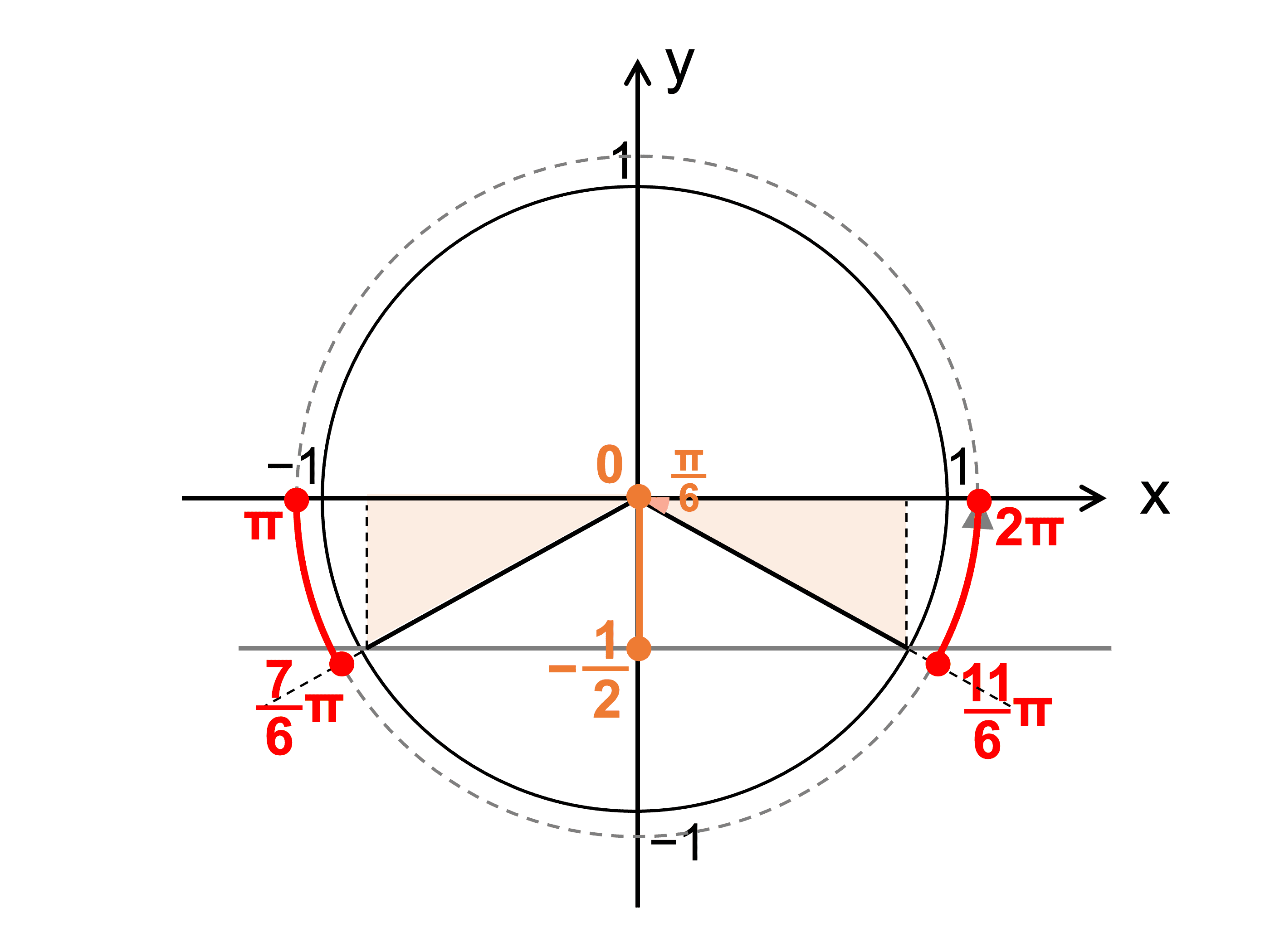
よって、求める \(\theta\) の範囲は
\(\displaystyle \pi \leq \theta \leq \frac{7}{6}\pi, \frac{11}{6}\pi \leq \theta \leq 2\pi\)
答え: \(\color{red}{\displaystyle \pi \leq \theta \leq \frac{7}{6}\pi, \frac{11}{6}\pi \leq \theta \leq 2\pi}\)
計算問題④「sin, tan の角度を変換する」
\(0 \leq \theta \leq 90^\circ\)、\(\displaystyle \sin \theta = \frac{3}{5}\) のとき、次の値を求めよ。
(1) \(\sin(90^\circ − \theta)\)
(2) \(\tan(180^\circ − \theta)\)

角度を変換する問題です。
まずは \(\cos \theta\) の値を求めておき、変換公式を活用しましょう。
単位円を書いて確認するとより確実です。
\(0 \leq \theta \leq 90^\circ\) より \(0 \leq \cos \theta \leq 1\)
\(\sin^2\theta + \cos^2\theta = 1\) より、
\(\begin{align}\cos \theta &= +\sqrt{1 − \sin^2\theta} \\&= +\sqrt{1 − \left( \frac{3}{5} \right)^2} \\&= +\sqrt{1 − \frac{9}{25}} \\&= +\sqrt{\frac{16}{25}} \\&= \frac{4}{5}\end{align}\)
(1)

\(\sin(90^\circ − \theta) = \cos \theta = \displaystyle \frac{4}{5}\)
答え: \(\color{red}{\displaystyle \frac{4}{5}}\)
(2)

\(\begin{align}\tan(180^\circ − \theta) &= −\tan \theta\\&\displaystyle = −\frac{\sin \theta}{\cos \theta}\\&\displaystyle = −\frac{\frac{3}{5}}{\frac{4}{5}}\\&\displaystyle = −\frac{3}{4}\end{align}\)
答え: \(\color{red}{\displaystyle −\frac{3}{4}}\)
以上で計算問題も終わりです!
三角比・三角関数の問題では、単位円を使って角度を求める機会が非常に多いです。
できて当たり前というレベルにしておきましょうね!

