この記事では、「接弦定理」についてわかりやすく解説していきます。
接弦定理の逆や定理の証明、問題の解き方も説明していきますので、ぜひこの記事を通してマスターしてくださいね!
接弦定理とは?
接弦定理とは、三角形の外接円とその接線がなす角度に関して成り立つ定理です。
円に \(\triangle \mathrm{ABC}\) が内接し、接点 \(\mathrm{A}\) で円が直線 \(\mathrm{AT}\) と接するとき、
\begin{align}\color{red}{\angle \mathrm{TAB} = \angle \mathrm{ACB}}\end{align}
が成り立つ。
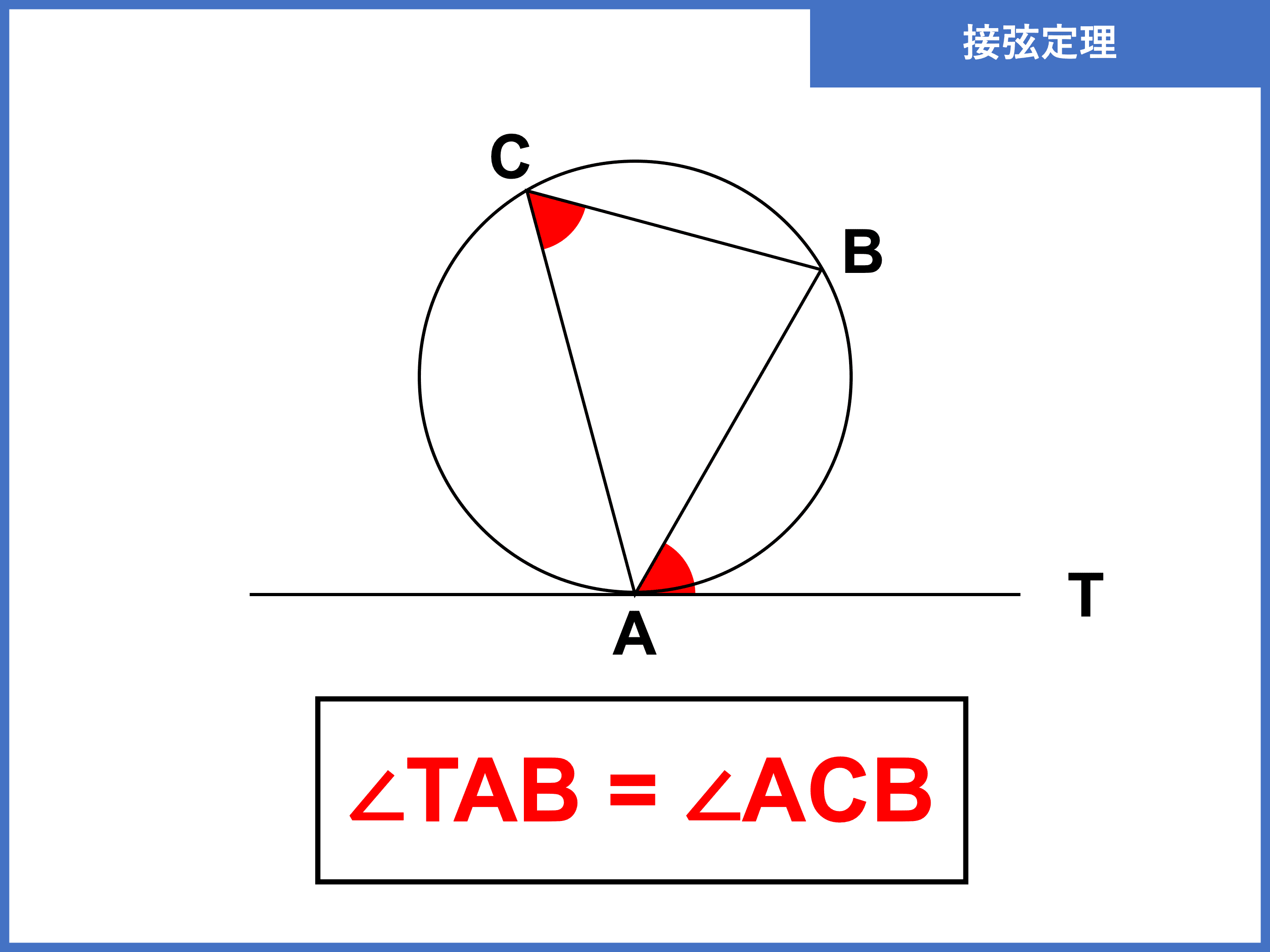
接線 \(\mathrm{AT}\) と弦 \(\mathrm{AB}\) がなす角度 \(\angle \mathrm{TAB}\) は、弧 \(\mathrm{AB}\) に対する円周角 \(\angle \mathrm{ACB}\) と等しくなります。
頂点の記号がいつも同じとは限らないので、記号で丸暗記するのではなく、図の位置関係で接弦定理を理解しておきましょう!
接弦定理の証明
ここでは、接弦定理がなぜ成り立つのかを証明します。
円に \(\triangle \mathrm{ABC}\) が内接し、接点 \(\mathrm{A}\) で円が直線 \(\mathrm{AT}\) と接するとき、接弦定理
\begin{align}\angle \mathrm{TAB} = \angle \mathrm{ACB}\end{align}
を証明せよ。
円周角の定理「\(1\) つの弧に対する円周角の大きさは等しい」より、円周角は自由な位置にとれるので、直角三角形をつくって直角をうまく利用するのがポイントです。
接線と弦が作る角が、(i) 鋭角、(ii) 直角、(iii) 鈍角になる場合の \(3\) 通りに分けてそれぞれ証明します。
(i) \(\angle \mathrm{TAB}\) が鋭角の場合 \((\angle \mathrm{TAB} < 90^\circ)\)
線分 \(\mathrm{AD}\) が円の直径となるように点 \(\mathrm{D}\) をとる。
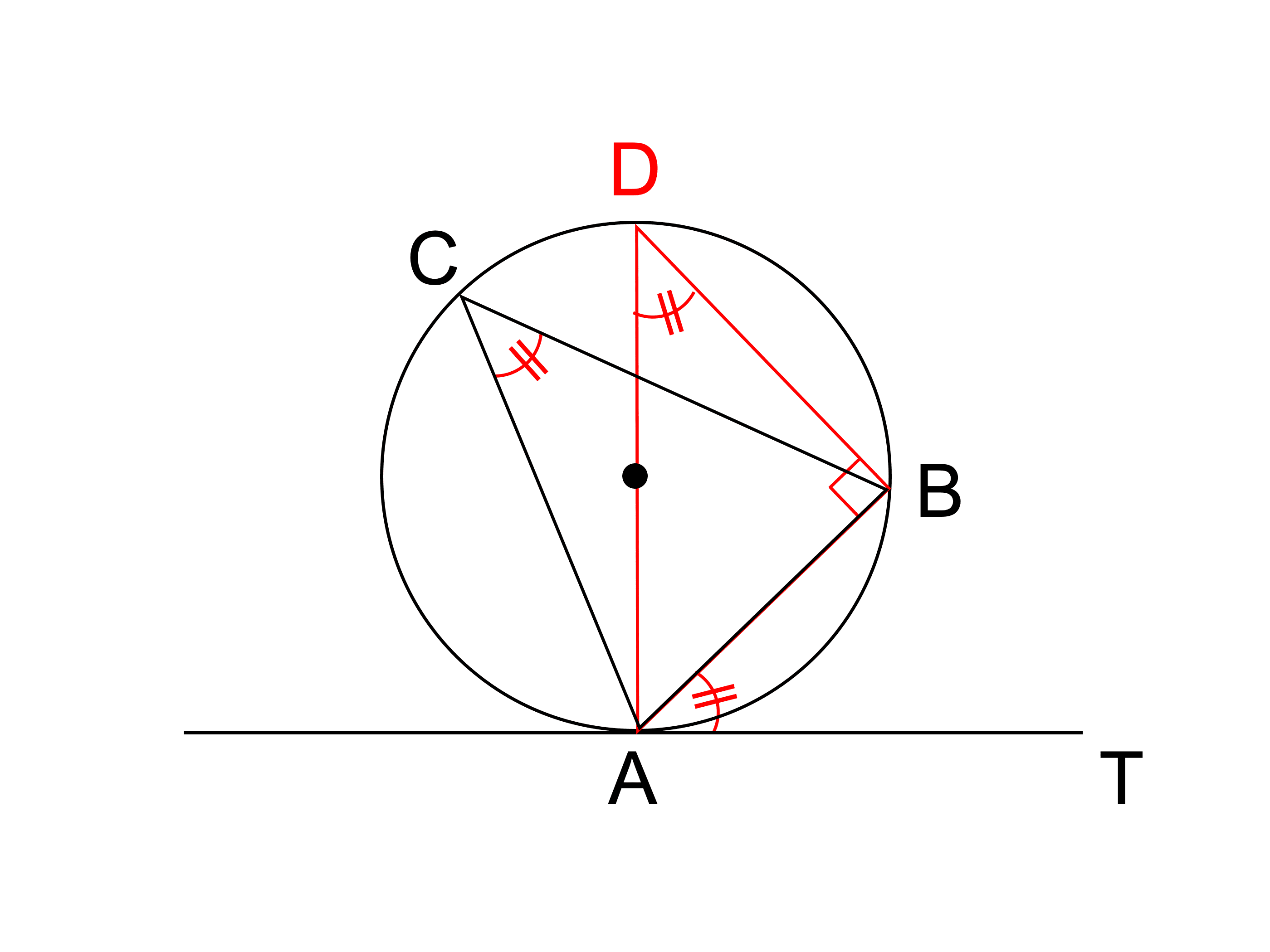
図において、円周角の定理より
\(\angle \mathrm{ACB} = \angle \mathrm{ADB}\) …①
直径に対する円周角であるから
\(\angle \mathrm{ABD} = 90^\circ\)
三角形の内角の和は \(180^\circ\) なので
\(\angle \mathrm{ADB} = 90^\circ − \angle \mathrm{BAD}\) …②
また、\(\mathrm{AT}\) は円の接線であるから
\(\mathrm{AD}\) と垂直に交わり、
\(\angle \mathrm{DAT} = 90^\circ\)
よって
\(\angle \mathrm{TAB} = 90^\circ − \angle \mathrm{BAD}\) …③
②、③より、
\(\angle \mathrm{ADB} = \angle \mathrm{TAB}\) …④
①、④より、
\(\angle \mathrm{TAB} = \angle \mathrm{ACB}\)
(ii) \(\angle \mathrm{TAB}\) が直角の場合 \((\angle \mathrm{TAB} = 90^\circ)\)
\(\angle \mathrm{TAB}\) が直角のとき、\(\mathrm{AB}\) は円の直径である。
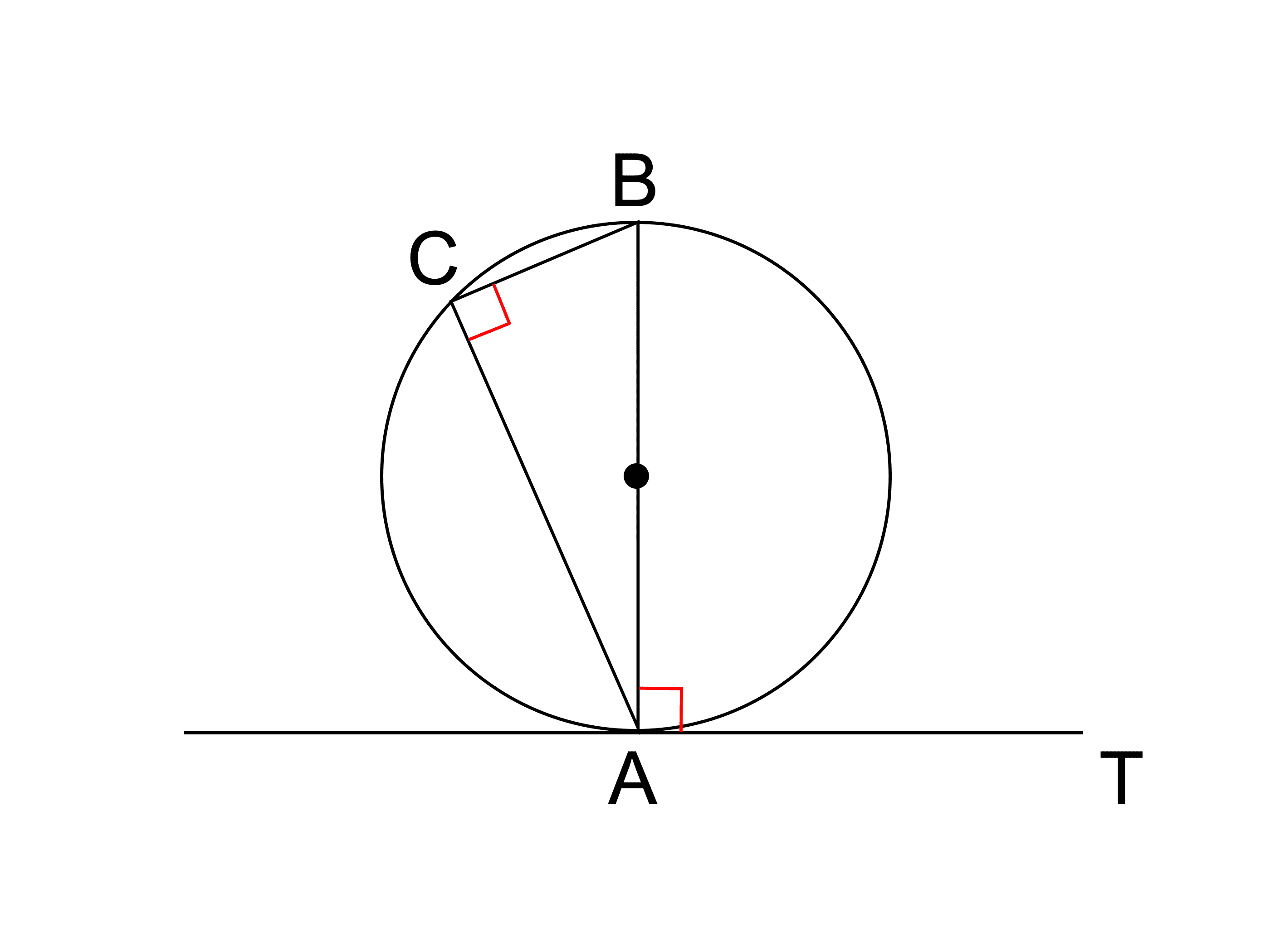
直径に対する円周角であるから
\(\angle \mathrm{ACB} = 90^\circ\) …①
また、\(\mathrm{AT}\) は円の接線であるから、\(\mathrm{AB}\) と垂直に交わり、
\(\angle \mathrm{TAB} = 90^\circ\)…②
①、②より、
\(\angle \mathrm{TAB} = \angle \mathrm{ACB}\)
(iii) \(\angle \mathrm{TAB}\) が鈍角の場合 \((\angle \mathrm{TAB} > 90^\circ)\)
接線 \(\mathrm{AT}\) の \(\mathrm{T}\) の反対側に \(\mathrm{T’}\) をとり、線分 \(\mathrm{AD}\) が円の直径となるように点 \(\mathrm{D}\) をとる。
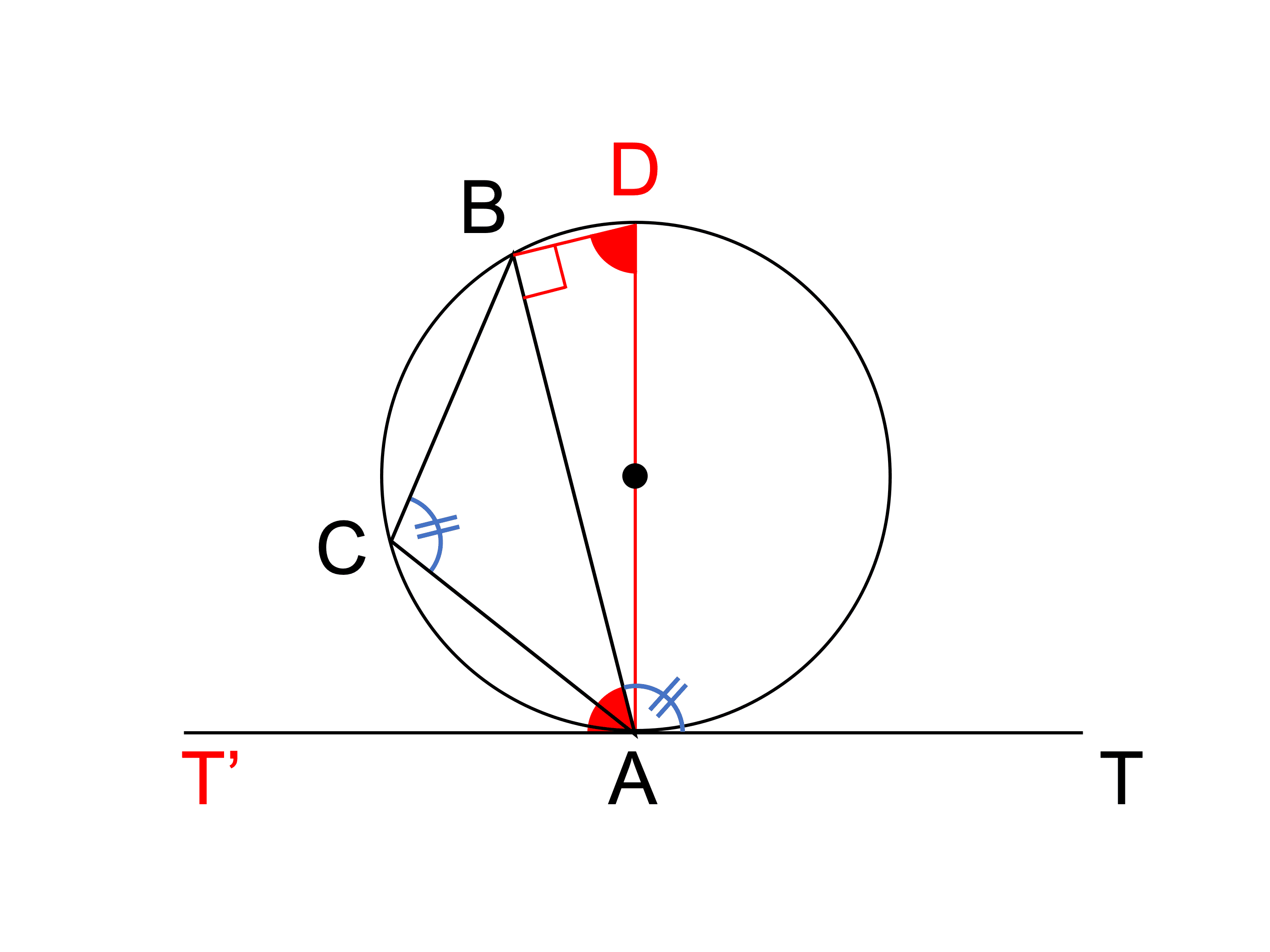
直径に対する円周角より、
\(\angle \mathrm{ABD} = 90^\circ\)
三角形の内角の和は \(180^\circ\) なので
\(\angle \mathrm{ADB} = 90^\circ − \angle \mathrm{BAD}\) …①
また、\(\mathrm{AT’}\) は円の接線であるから、
\(\angle \mathrm{DAT’} = 90^\circ\) なので、
\(\angle \mathrm{T’AB} = 90^\circ − \angle \mathrm{BAD}\) …②
①、②より、
\(\angle \mathrm{T’AB} = \angle \mathrm{ADB}\) …③
また、\(\angle \mathrm{TAB} = 180^\circ − \angle \mathrm{T’AB}\) …④
四角形 \(\mathrm{ABCD}\) は円に内接するので、
\(\angle \mathrm{ACB} = 180^\circ − \angle \mathrm{ADB}\) …⑤
③、④、⑤より、
\(\angle \mathrm{TAB} = \angle \mathrm{ACB}\)
(i) ~ (iii) より、\(\angle \mathrm{TAB}\) の大きさによらず、接弦定理は成り立つ。
(証明終わり)
接線と弦が作る角がどのような角度でも接弦定理が成り立つことが証明できましたね!
たまに証明自体が出題されることもあるので、証明の流れは押さえておきましょう。
接弦定理の逆
接弦定理は、その逆も成り立ちます。
点 \(\mathrm{C}\) と \(\mathrm{T}\) が直線 \(\mathrm{AB}\) に対して反対側にあり、\(\angle \mathrm{TAB} = \angle \mathrm{ACB}\) が成り立つならば、
直線 \(\mathrm{AT}\) は \(\triangle \mathrm{ABC}\) の外接円と接する。
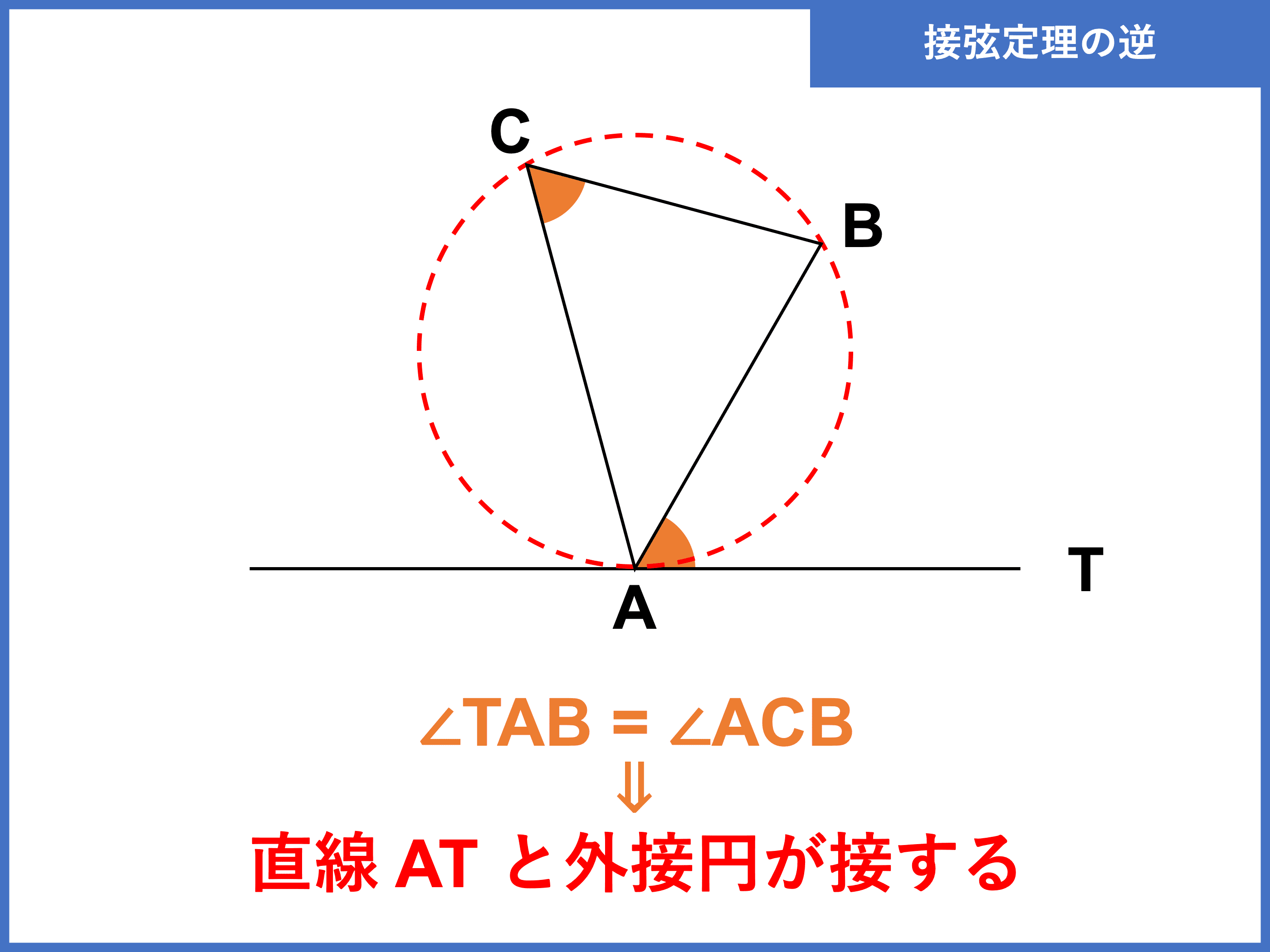
登場頻度は高くありませんが、まれに証明問題などで利用することがあります。
接弦定理の逆の証明
接弦定理の逆は、接弦定理から簡単に導けます。
\(\triangle \mathrm{ABC}\) の外接円の点 \(\mathrm{A}\) における接線上に、\(\mathrm{AB}\) に関して \(\mathrm{C}\) と反対側に点 \(\mathrm{D}\) をとると、
接弦定理より \(\angle \mathrm{ACB} = \angle \mathrm{DAB}\)
また、仮定より \(\angle \mathrm{TAB} = \angle \mathrm{DAB}\)
\(\mathrm{T}\), \(\mathrm{D}\) は \(\mathrm{AB}\) に関して同じ側にあるので \(\mathrm{A}\), \(\mathrm{T}\), \(\mathrm{D}\) は一直線上にある。
つまり直線 \(\mathrm{AT}\) は円の接線である。
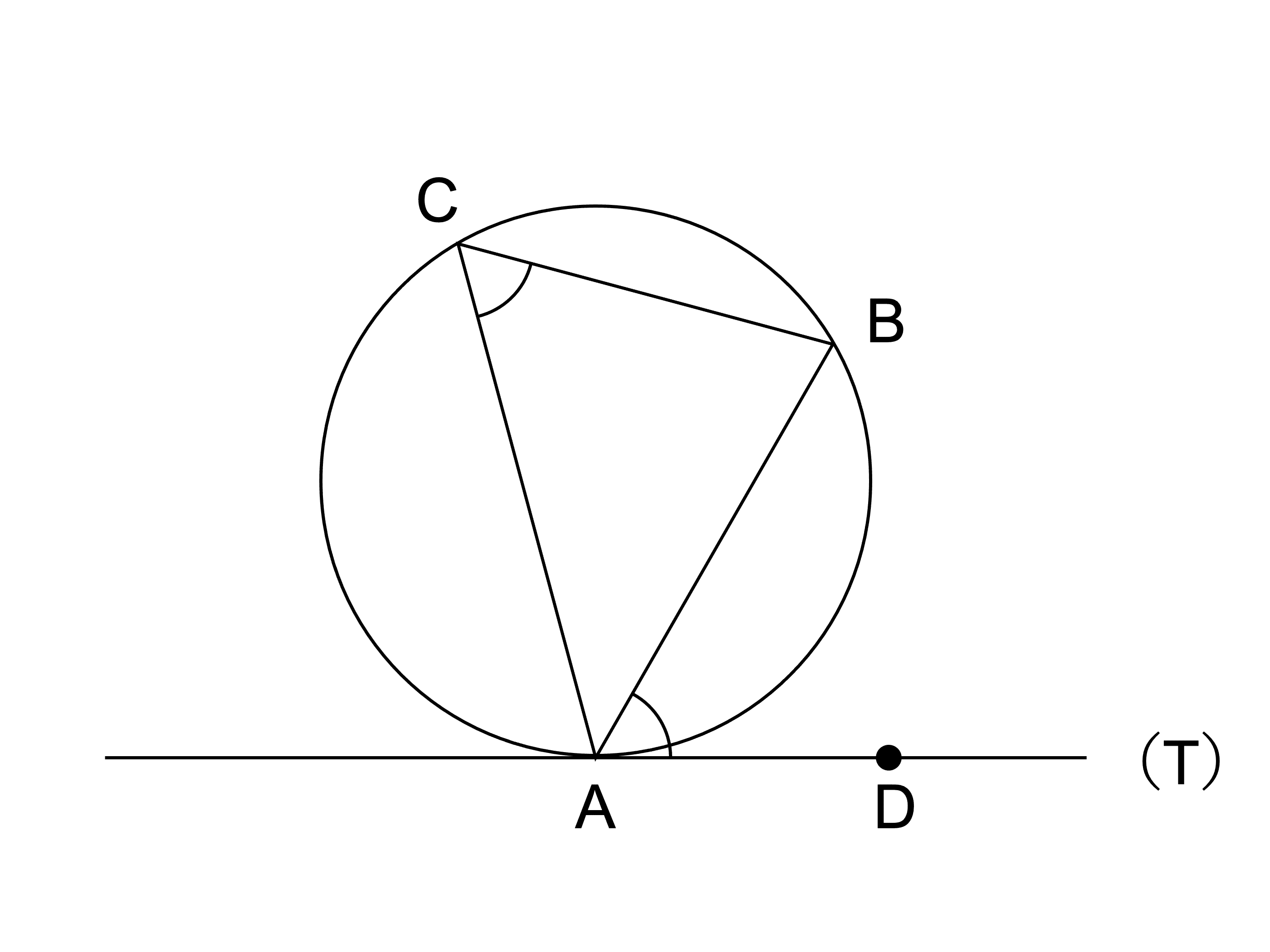
(証明終わり)
接弦定理の計算問題
それでは、接弦定理を利用した計算問題に挑戦してみましょう!
計算問題①「円に内接する三角形」
図において、円の弧 \(\mathrm{AB}\) が接線 \(\mathrm{ST}\) と接点 \(\mathrm{A}\) で接するとき、\(\angle \mathrm{CAB}\) を求めなさい。
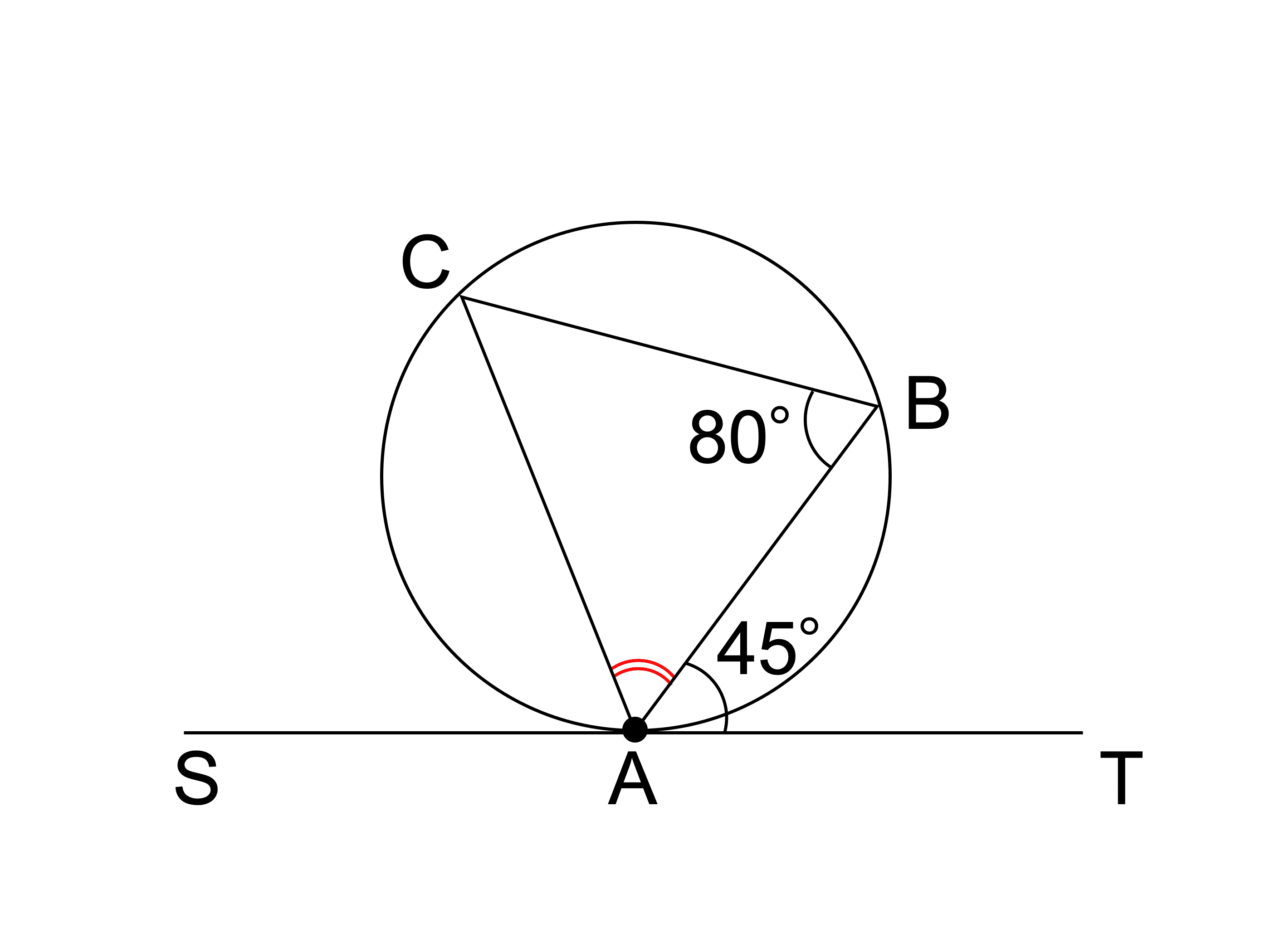

接弦定理をそのまま用いたら解けるシンプルな問題です!
どの弧において接弦定理を使うか考えましょう。
弧 \(\mathrm{AC}\) において、接弦定理より
\(\begin{align} \angle \mathrm{SAC} &= \angle \mathrm{ABC} \\ &= 80^\circ \end{align}\)
\(\begin{align} \angle \mathrm{CAB} &= 180^\circ − (\angle \mathrm{SAC} + \angle \mathrm{TAB}) \\ &= 180^\circ − (80^\circ + 45^\circ) \\ &= 55^\circ \end{align}\)
答え: \(55^\circ\)
計算問題②「円に内接する四角形」
図において、円の弧 \(\mathrm{AB}\) が接線 \(\mathrm{BT}\) と接点 \(\mathrm{B}\) で交わるとき、\(\angle x\)、\(\angle y\) を求めなさい。
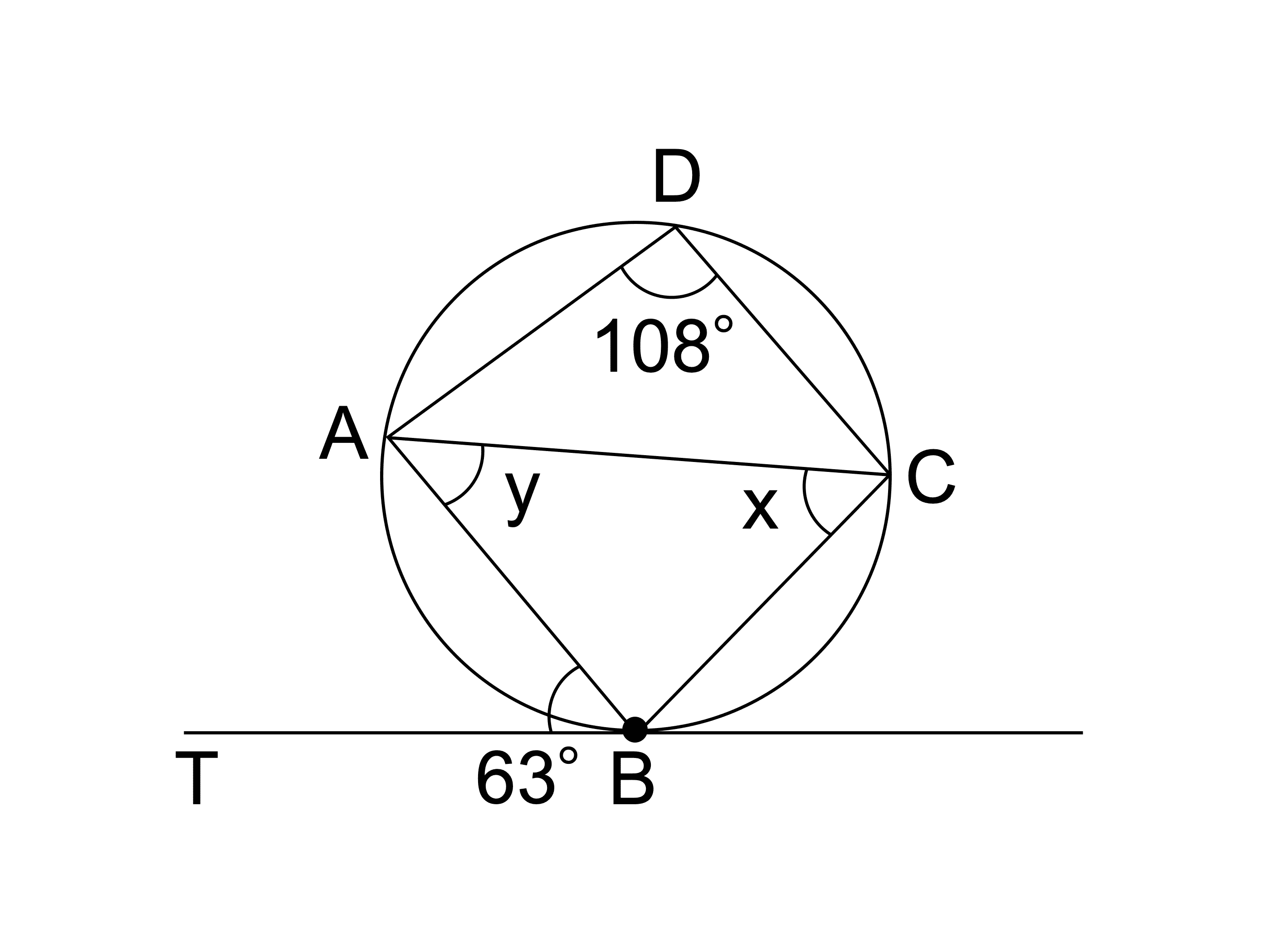

接弦定理を使うことで \(1\) つの値が求まります。
また、円に内接する四角形の性質を利用すると、もう \(1\) つの値も求められます。
弧 \(\mathrm{AB}\) において、接弦定理より
\(\angle \mathrm{ACB} = \angle \mathrm{TBA}\) なので、
\(\angle x = 63^\circ\)
また、円に内接する四角形の性質より、
\(\angle \mathrm{ABC} + \angle \mathrm{ADC} = 180^\circ\) であるから、
\(\begin{align} \angle \mathrm{ABC} &= 180^\circ − 108^\circ \\ &= 72^\circ \end{align}\)
\(\triangle \mathrm{ABC}\) において、内角の和の性質より
\(\angle \mathrm{BAC} = 180^\circ − (\angle \mathrm{ABC} + \angle \mathrm{ACB})\)
よって、
\(\begin{align} \angle y &= 180^\circ − (72^\circ + 63^\circ) \\ &= 45^\circ \end{align}\)
答え: \(\angle x = 63^\circ\)、\(\angle y = 45^\circ\)
計算問題③「円と 2 接線」
図において、直線 \(\mathrm{PA}\)、\(\mathrm{PB}\) はそれぞれ点 \(\mathrm{A}\)、\(\mathrm{B}\) で円 \(\mathrm{O}\) と接する接線である。
このとき、\(\angle x\) を求めなさい。
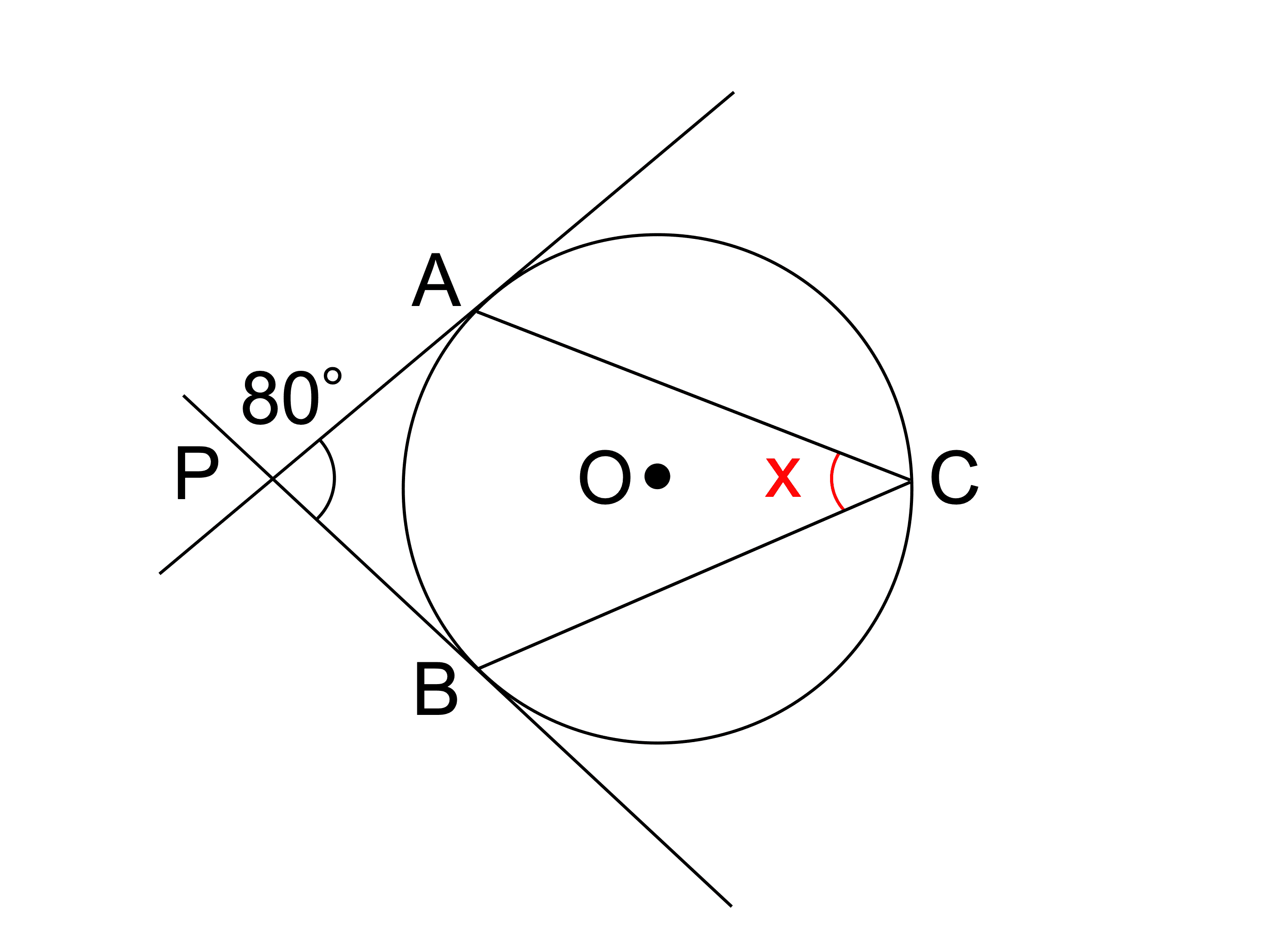

一見接弦定理が使えるのか悩む図形ですね。
接線と弦が作る角が関係する問題なので、この問題にも接弦定理が利用できます。
上手く補助線を引いてみましょう。
補助線 \(\mathrm{AB}\) を引く。
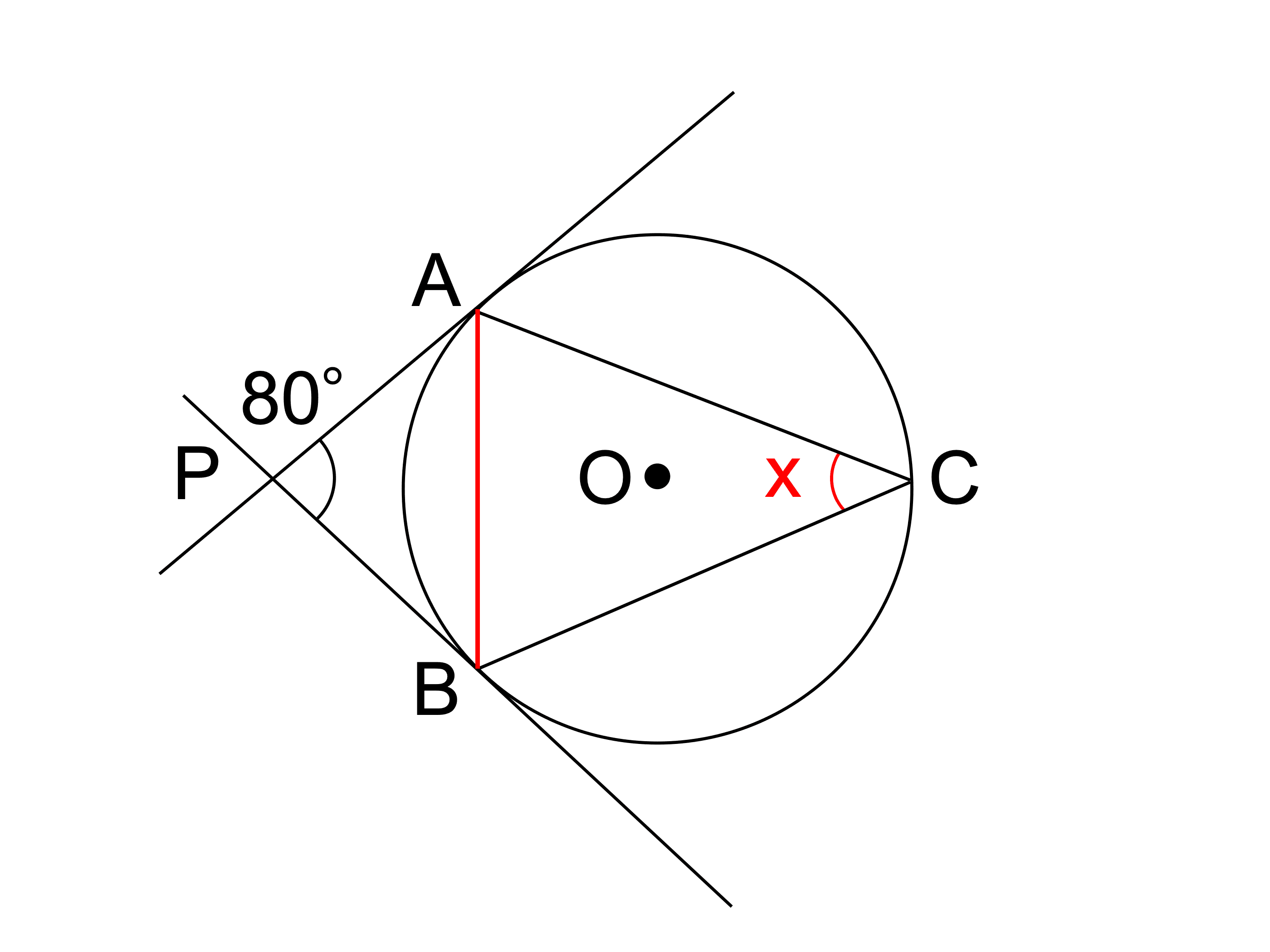
接線 \(\mathrm{PA}\) において、弧 \(\mathrm{AB}\) における接弦定理より、
\(\angle \mathrm{PAB} = \angle \mathrm{ACB} = \angle x\) …①
接線 \(\mathrm{PB}\) において、弧 \(\mathrm{AB}\) における接弦定理より、
\(\angle \mathrm{PBA} = \angle \mathrm{ACB} = \angle x\) …②
①、②より、
\(\angle \mathrm{PAB} = \angle \mathrm{PBA} = \angle x\)

\(\triangle \mathrm{PAB}\) において、内角の和の性質より、
\(\begin{align} \angle x &= \frac{180^\circ − \angle \mathrm{APB}}{2}\\ &= \frac{180^\circ − 80^\circ}{2} \\ &= \frac{100^\circ}{2}\\ &= 50^\circ \end{align}\)
答え: \(50^\circ\)
以上で問題も終わりです!
接弦定理、しっかりと覚えることができましたか?
どの角度とどの角度が等しいのか、忘れがちな公式です。図の位置関係で理解しておきましょう!

