この記事では「円周角の定理」や「円周角の定理の逆」について、図を使いながらわかりやすく解説していきます。
一緒に円周角の計算問題や証明問題をマスターしていきましょう!
目次
円周角の定理とは?
円周角の定理とは、円周角と中心角について成り立つ以下の定理です。
① \(1\) つの弧に対する円周角の大きさは、その弧に対する中心角の半分である
② \(1\) つの弧に対する円周角の大きさは等しい
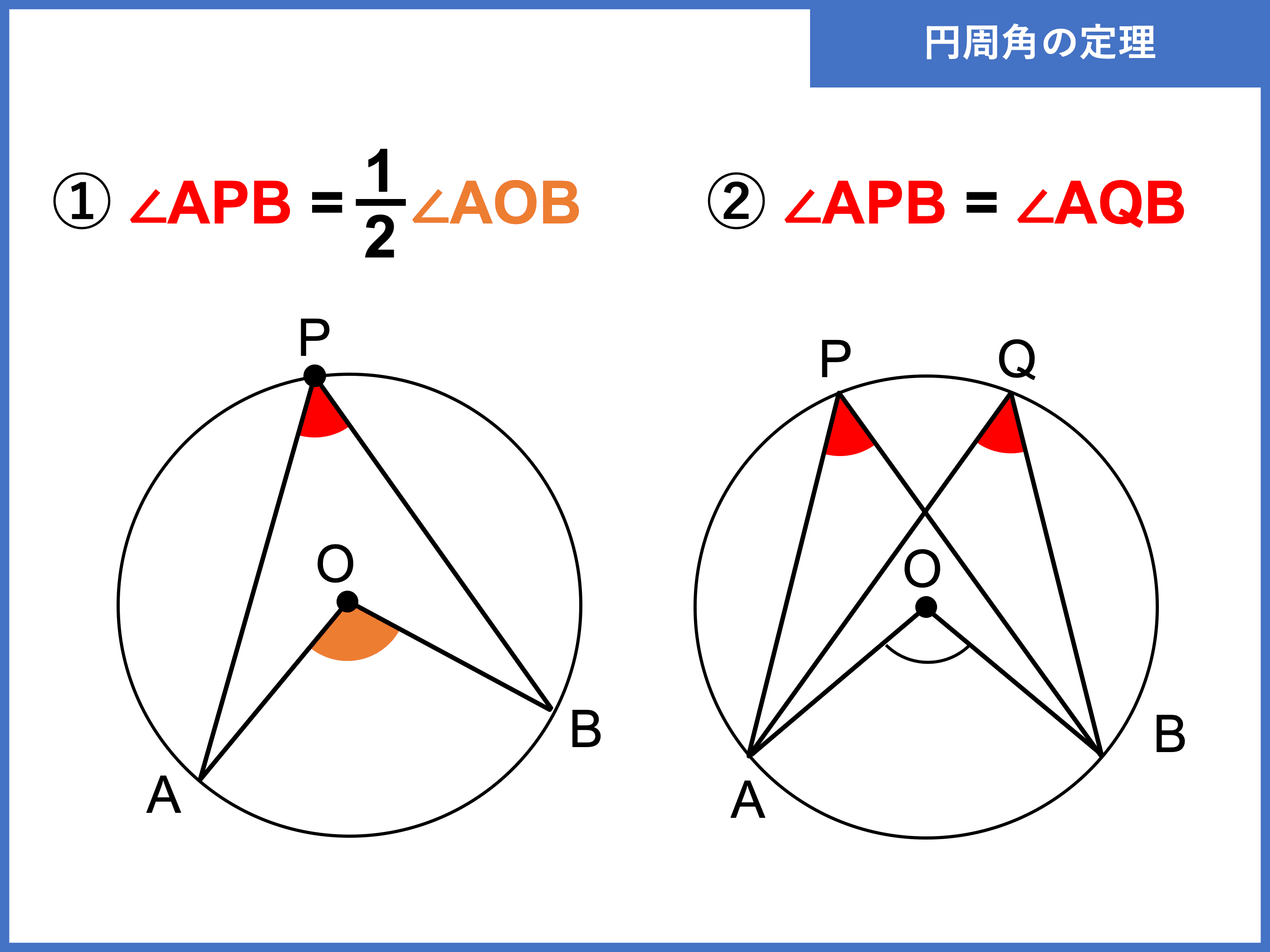
円周角の定理は \(2\) つとも絶対に覚えておくようにしましょう!
【復習】円周角と中心角
そもそも、「円周角」と「中心角」とはどんなものだったでしょうか。
- 円周角
円の弧の両端と円周上のある点がなす角 - 中心角
円の弧の両端と円の中心がなす角
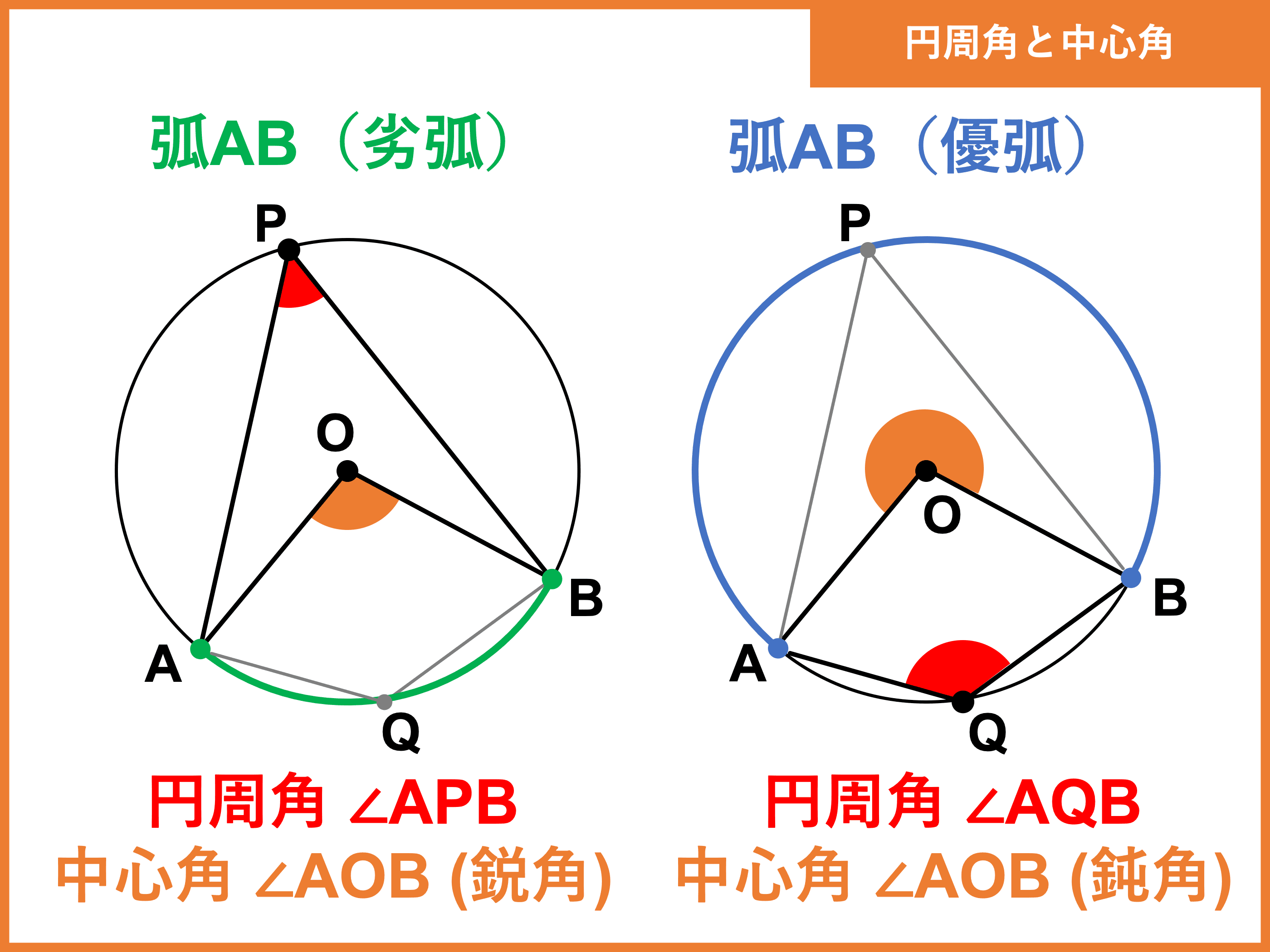
「弧 \(\mathrm{AB}\)」には、弧の長さが長い方(優弧)と短い方(劣弧)があります。
両者はまったく別物であり、それぞれに対して円周角と中心角があります。
そのため、「点 \(\mathrm{P}\) の反対側の弧」や「点 \(\mathrm{P}\) のある側の弧」など、第 \(3\) の点を用いて区別することもあります。
一般的に、ことわりがなければ劣弧を差すことが多いですが、必ずではないので注意しましょう。
円周角の性質
円周角には、次の重要な性質があります。
① \(1\) つの弧に対する円周角はその中心角の半分
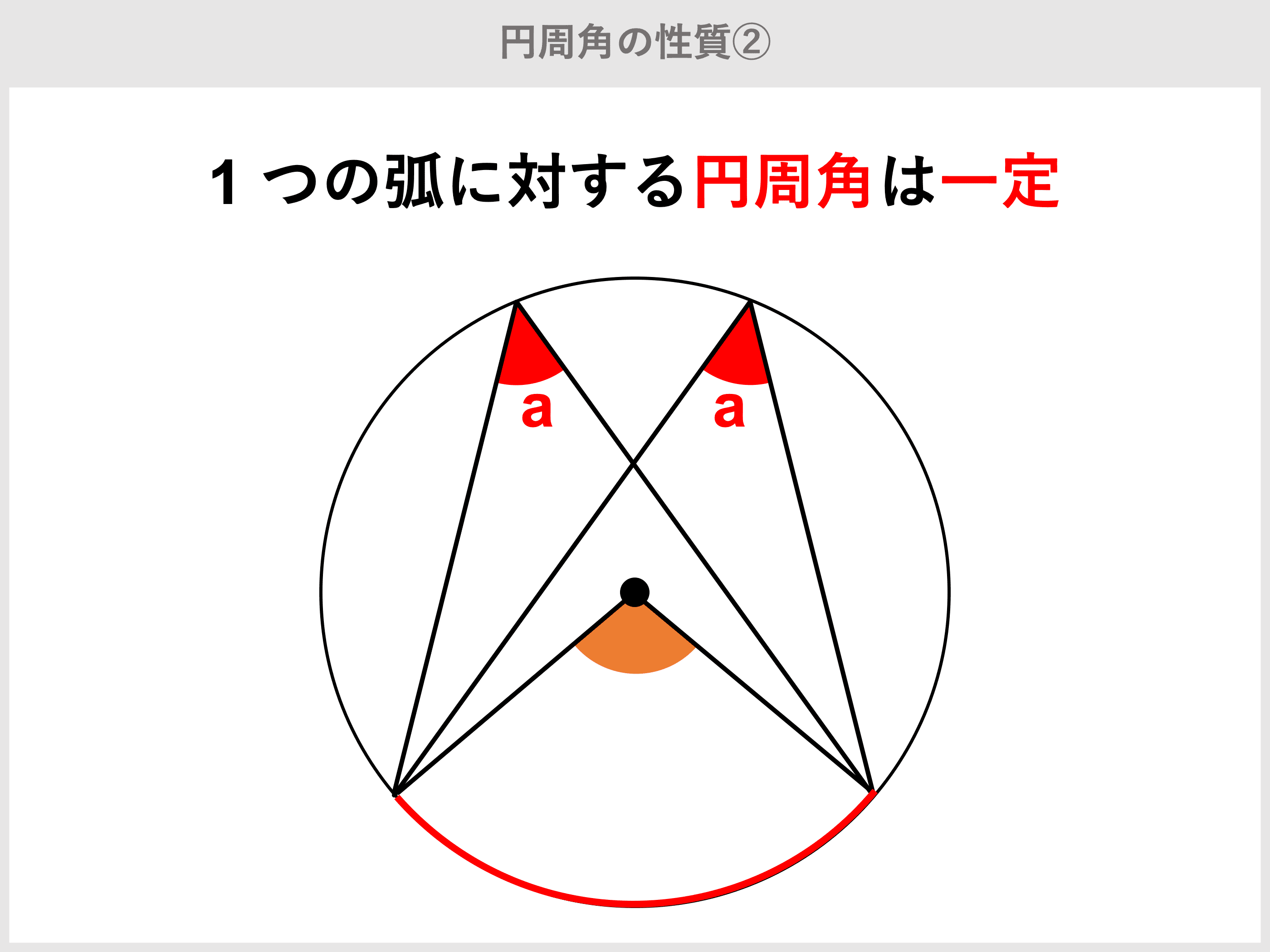
② \(1\) つの弧に対する円周角は一定
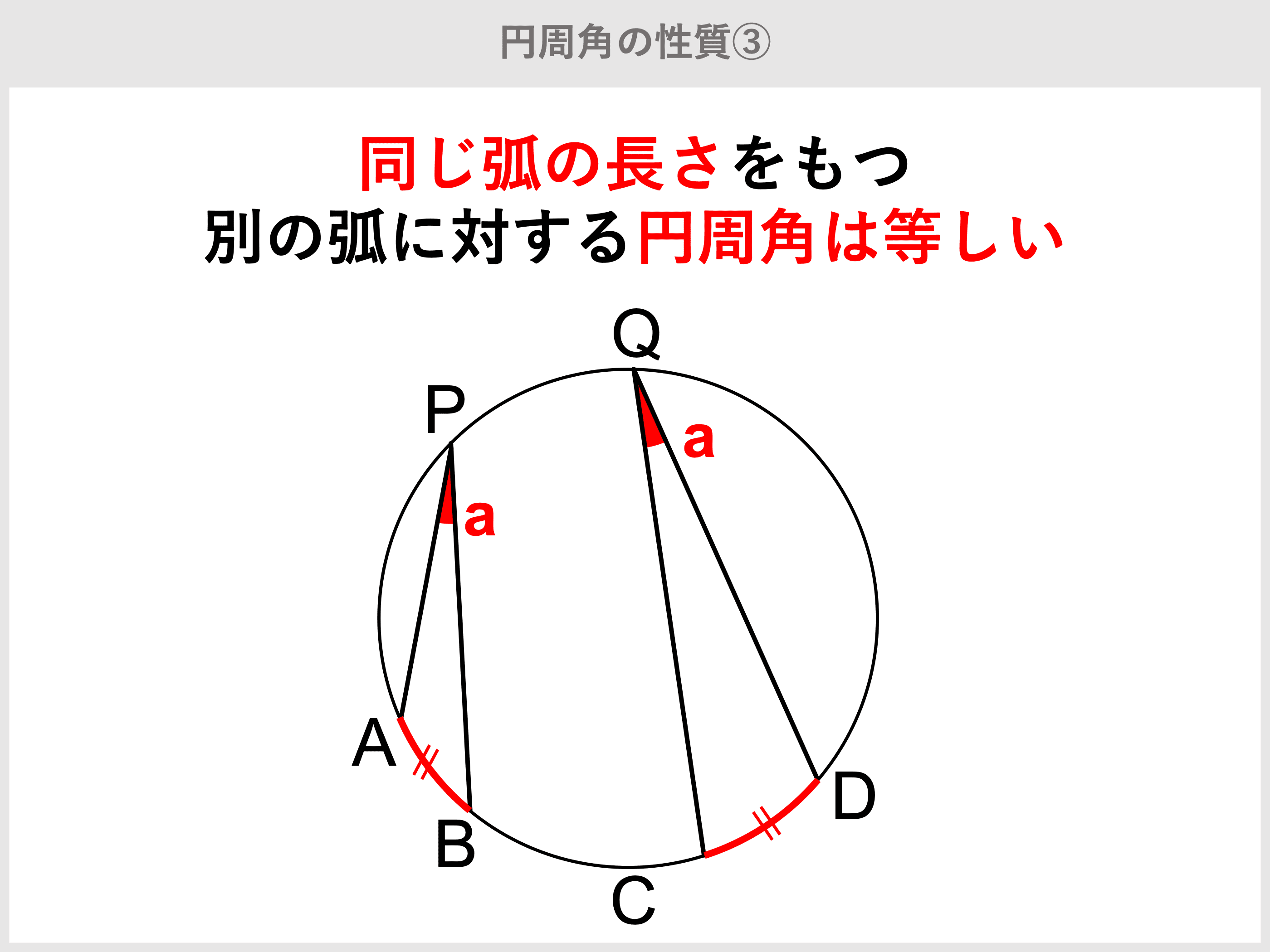
③ 同じ弧の長さをもつ別の弧に対する円周角は等しい
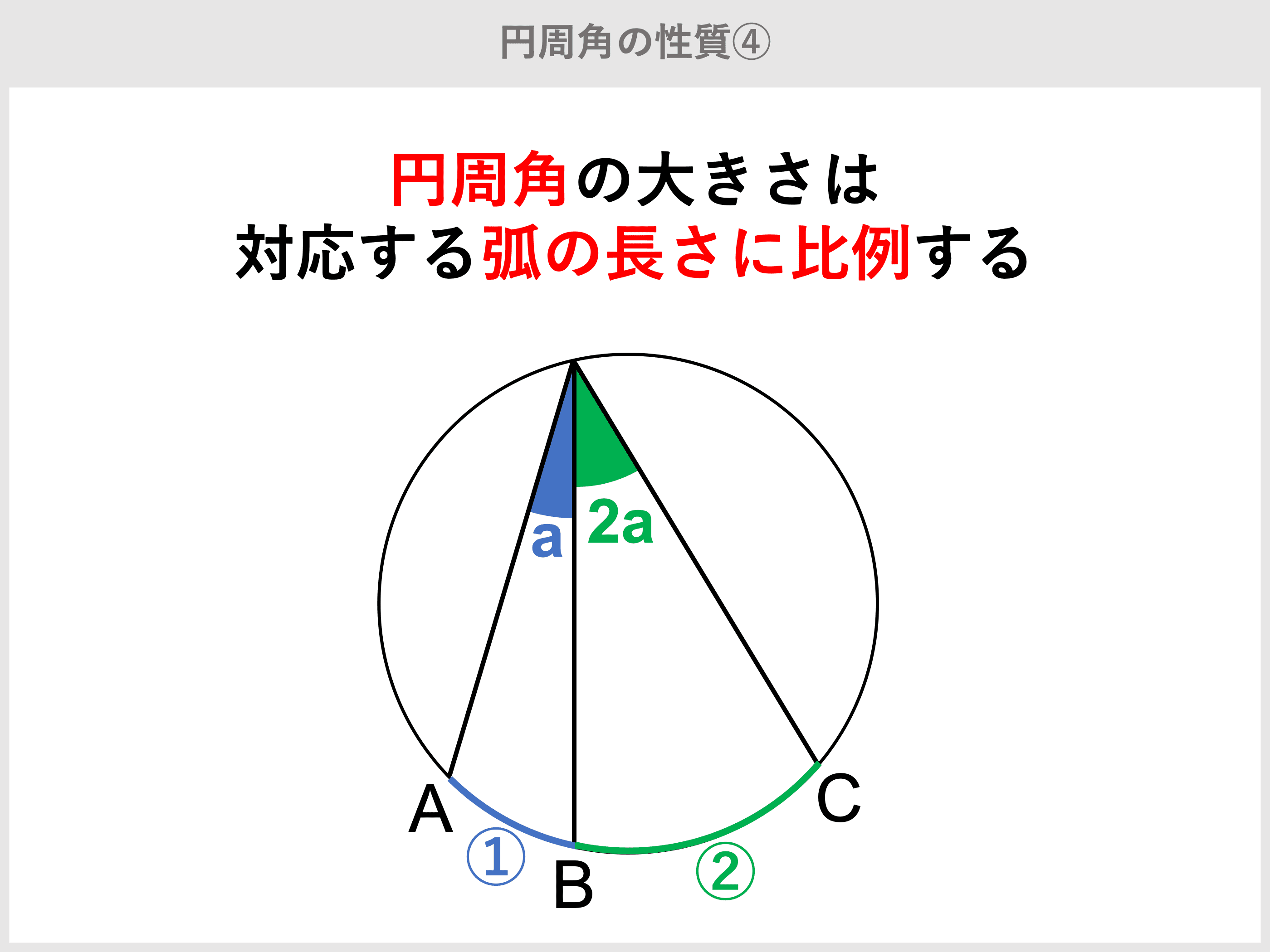
④ 円周角の大きさは対応する弧の長さに比例する
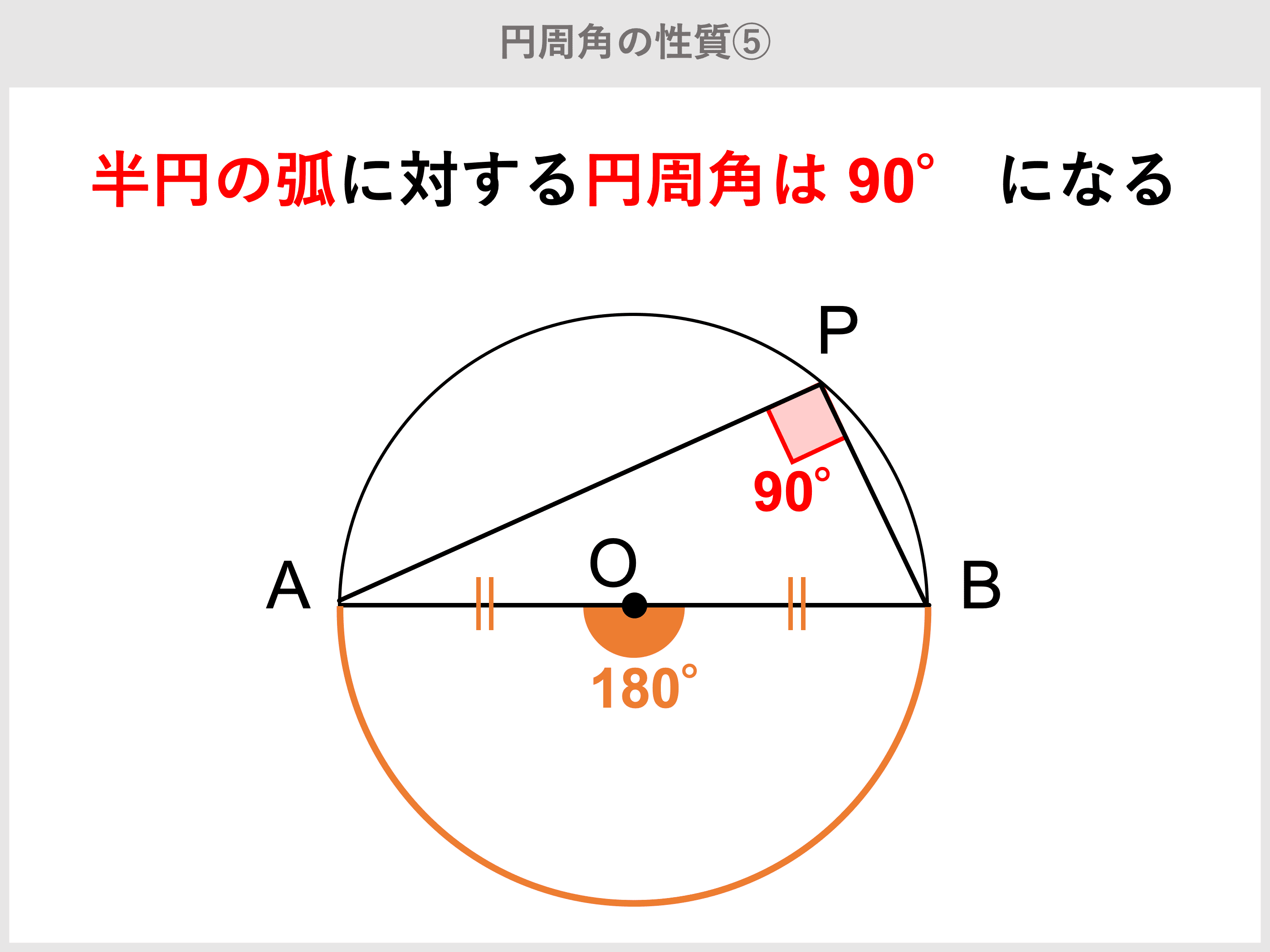
⑤ 半円の弧に対する円周角は \(90^\circ\) になる
円周角の定理の内容と合わせて、円周角の性質を図で見ていきましょう。
性質①は、円周角の定理の①のことですね。

性質②は、円周角の定理の②のことです。
\(1\) つの円周上で弧の位置が違っても、弧の長さが同じであればそれぞれの弧に対する円周角は等しくなります。
同じ円周上にあれば、弧の長さとその弧に対する円周角の大きさは比例関係にあります。
半円の弧に対する中心角が \(180^\circ\) になることから、その円周角は必ず \(90^\circ\) となります。
性質⑤はよく利用するので、必ず押さえておきましょう!
円周角の定理の逆とは?
円周角の定理は、その逆も成り立ちます。
\(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\)、\(\mathrm{Q}\) について、点 \(\mathrm{P}\)、\(\mathrm{Q}\) が直線 \(\mathrm{AB}\) の同じ側にあって、\(\angle \mathrm{APB} = \angle \mathrm{AQB}\) ならば、この \(4\) 点は \(1\) つの円周上にある。

「\(4\) 点の位置関係と角度から、それらが同一円周上にあることを示す」のが、円周角の定理の逆ということですね。
円周角の定理の逆は証明問題で使うこともあれば、角度を求める問題であと一歩ヒントが欲しいときに活用することもあります。
とりあえず、「円周角の定理は逆も成り立つんだ!」ということをインプットしておきましょう。
円周角の定理と逆の証明
ここでは、円周角の定理と、定理の逆が成り立つことを証明していきます。
① 円周角の定理の証明
まずは、円周角の定理の証明を示します。
\(1\) つの弧に対する円周角の大きさは一定であり、その弧に対する中心角の半分であることを証明せよ。
円周角の位置によって場合分けして考えます。
弧 \(\mathrm{AB}\) に対する円周角を \(\angle \mathrm{APB}\)、中心角を \(\angle \mathrm{AOB}\) とする。
(i) 円周角 \(\angle \mathrm{APB}\) の内側に円の中心 \(\mathrm{O}\) がある場合

直線 \(\mathrm{PO}\) が円周と交わる点を \(\mathrm{C}\) とする。
弧 \(\mathrm{AC}\) に対する円周角を \(a\)、弧 \(\mathrm{CB}\) に対する円周角を \(b\) とする。
\(\triangle \mathrm{APO}\) と \(\triangle \mathrm{BPO}\) は円の半径を \(2\) 辺とする二等辺三角形であるから、
\(\angle \mathrm{APO} = \angle \mathrm{OAP} = a\)
\(\angle \mathrm{BPO} = \angle \mathrm{OBP} = b\)
三角形の外角の和から
\(\angle \mathrm{AOC} = \angle \mathrm{APO} + \angle \mathrm{OAP} = 2a\)
\(\angle \mathrm{BOC} = \angle \mathrm{BPO} + \angle \mathrm{OBP} = 2b\)
\(\begin{align} \angle \mathrm{AOB} &= \angle \mathrm{AOC} + \angle \mathrm{BOC} \\ &= 2(a + b) \\ &= 2(\angle \mathrm{APO} + \angle \mathrm{BPO}) \\ &= 2 \angle \mathrm{APB} \end{align}\)
したがって、弧 \(\mathrm{AB}\) に対する円周角 \(\angle \mathrm{APB}\) は、その弧に対する中心角 \(\angle \mathrm{AOB}\) の半分である。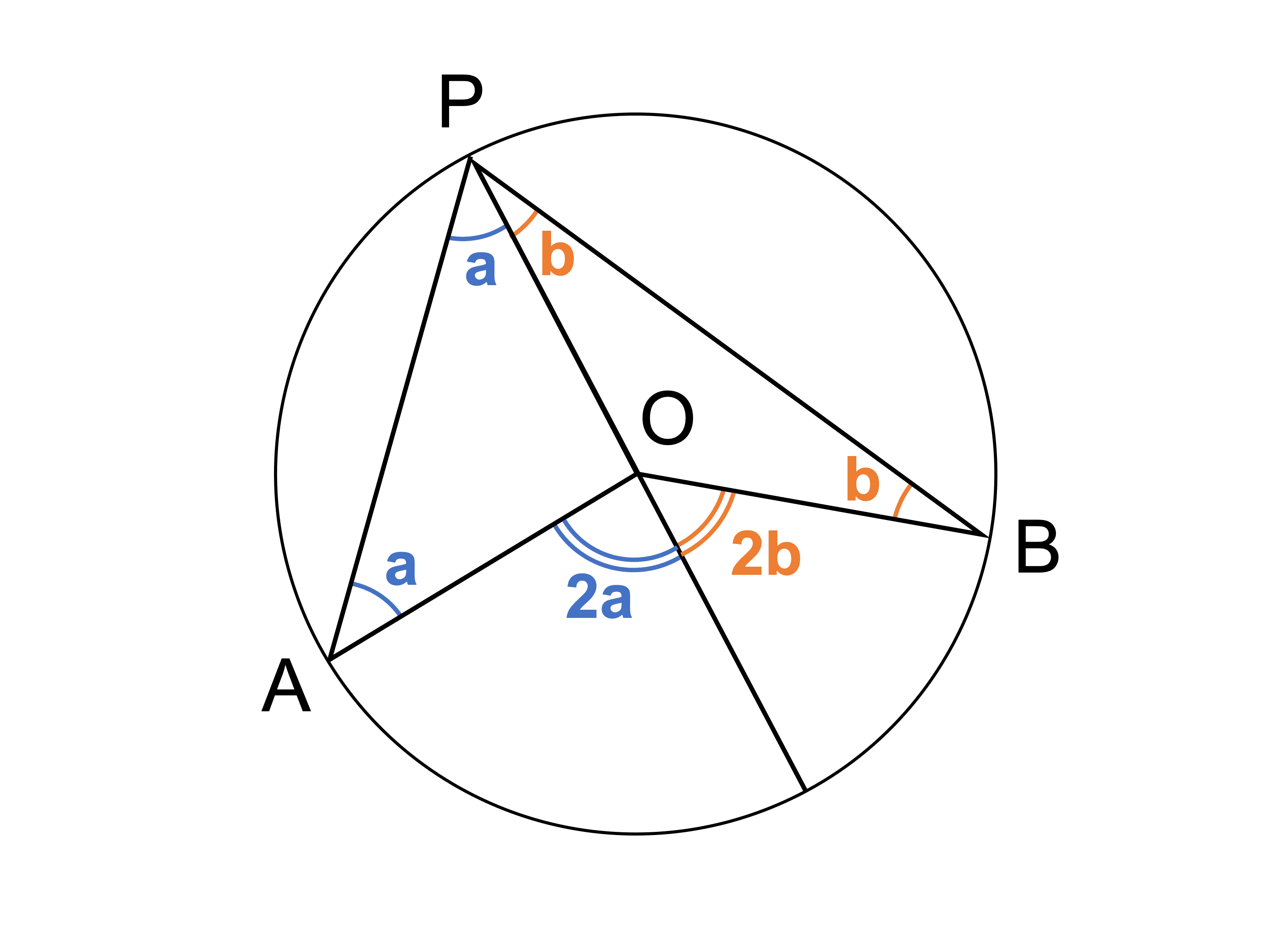
(ii) 円周角 \(\angle \mathrm{APB}\) を作る線分 \(\mathrm{PB}\) 上に円の中心 \(\mathrm{O}\) がある場合

\(\angle \mathrm{APB} = x\) とおく。
\(\mathrm{OA}\), \(\mathrm{OP}\) はともに円の半径であるから、
\(\triangle \mathrm{OAP}\) は \(\mathrm{OA} = \mathrm{OP}\) の二等辺三角形であり、
\(\angle \mathrm{OPA} = \angle \mathrm{OAP} = x\)
\(\triangle \mathrm{OAP}\) について、三角形の外角の和の性質から
\(\begin{align} \angle \mathrm{AOB} &= \angle \mathrm{OPA} + \angle \mathrm{OAP} \\ &= 2x \\ &= 2 \angle \mathrm{APB} \end{align}\)
したがって、弧 \(\mathrm{AB}\) に対する円周角 \(\angle \mathrm{APB}\) は、その弧に対する中心角 \(\angle \mathrm{AOB}\) の半分である。

(iii) 円周角 \(\angle \mathrm{APB}\) の外側に円の中心 \(\mathrm{O}\) がある場合

直線 \(\mathrm{PO}\) が円周と交わる点を \(\mathrm{C}\) とする。
弧 \(\mathrm{AC}\) に対する円周角を \(a\)、弧 \(\mathrm{CB}\) に対する円周角を \(b\) とする。
\(\triangle \mathrm{APO}\) と \(\triangle \mathrm{BPO}\) は円の半径を \(2\) 辺とする二等辺三角形であるから、
\(\angle \mathrm{APO} = \angle \mathrm{OAP} = a\)
\(\angle \mathrm{BPO} = \angle \mathrm{OBP} = b\)
三角形の外角の和から
\(\angle \mathrm{AOC} = \angle \mathrm{APO} + \angle \mathrm{OAP} = 2a\)
\(\angle \mathrm{BOC} = \angle \mathrm{BPO} + \angle \mathrm{OBP} = 2b\)
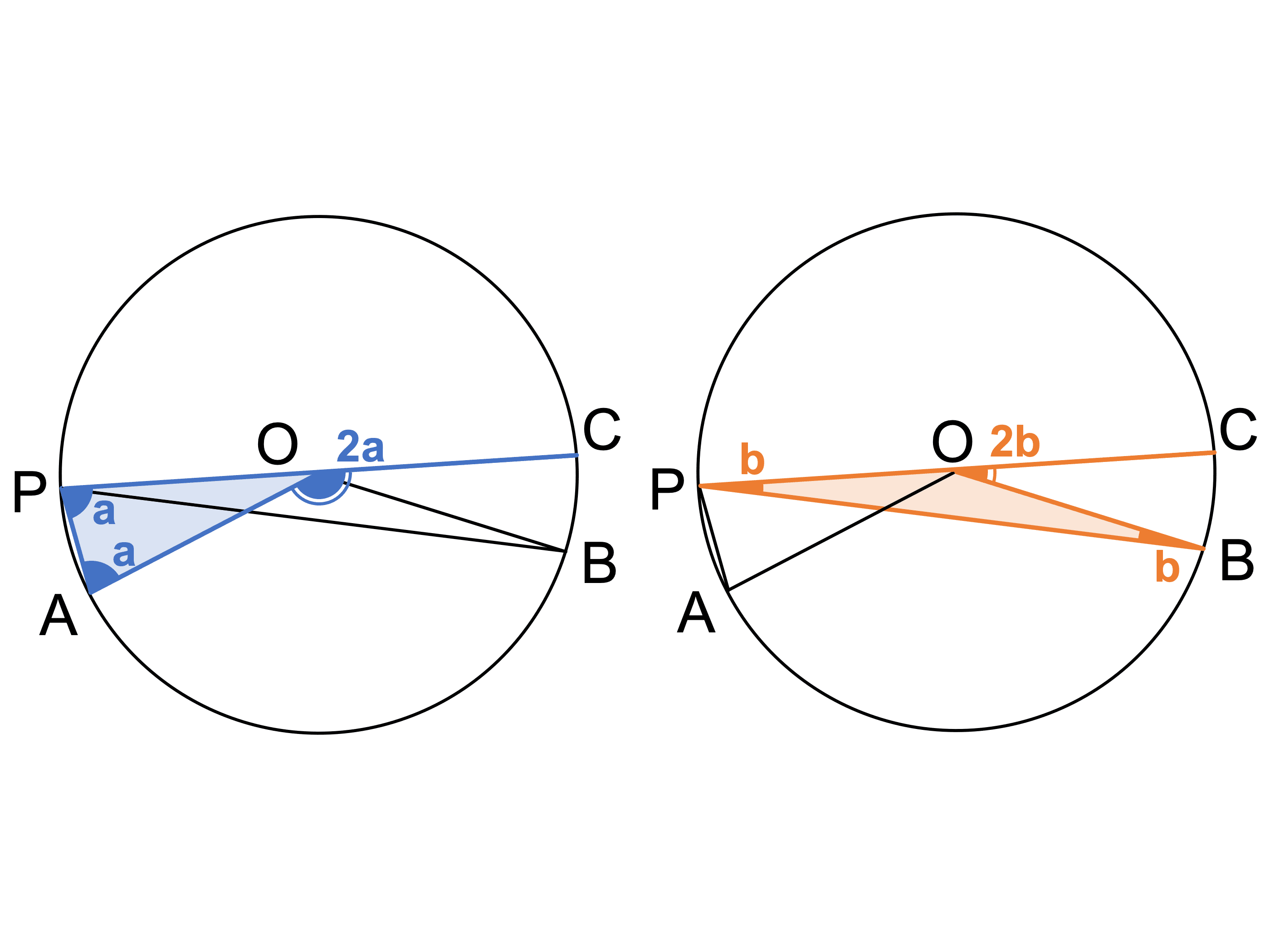
\(\begin{align} \angle \mathrm{AOB} &= \angle \mathrm{AOC} − \angle \mathrm{BOC} \\& = 2(a − b) \\ &= 2(\angle \mathrm{APO} − \angle \mathrm{BPO}) \\ &= 2 \angle \mathrm{APB} \end{align}\)
したがって、弧 \(\mathrm{AB}\) に対する円周角 \(\angle \mathrm{APB}\) は、その弧に対する中心角 \(\angle \mathrm{AOB}\) の半分である。
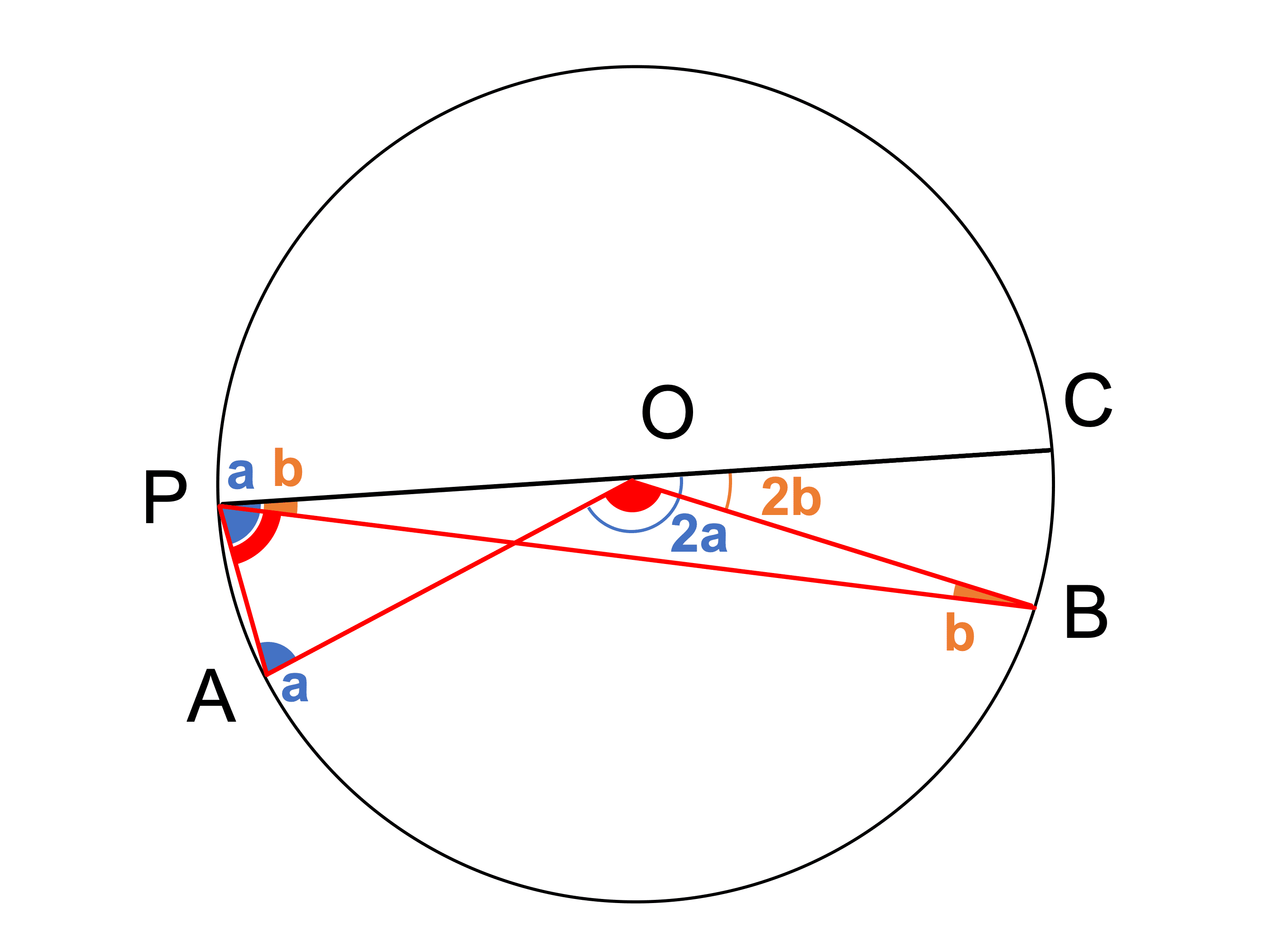
以上より、すべての場合において \(1\) つの弧に対する円周角はその弧に対する円周角の半分に等しい。
また、\(1\) つの弧に対する中心角は \(1\) つしか存在しないため、円周角がその頂点によらず常に中心角の半分であることが示されたことから、\(1\) つの弧に対する円周角は一定である。
(証明終わり)
以上が円周角の定理の証明になります。
場合分けがあって少し大変ですが、大まかな流れはほとんど同じなので、そのことを覚えておきましょう。
② 円周角の定理の逆の証明
続いて、円周角の定理の逆の証明を示します。
\(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\)、\(\mathrm{Q}\) について、点 \(\mathrm{P}\)、\(\mathrm{Q}\) が直線 \(\mathrm{AB}\) の同じ側にあって、\(\angle \mathrm{APB} = \angle \mathrm{AQB}\) ならば、この \(4\) 点は \(1\) つの円周上にあることを証明せよ。
どのような \(3\) 点も、\(1\) つの直線上になければ三角形を成し、三角形には必ずただ \(1\) つの外接円が定まります。
よって、円周角の定理の逆で登場する \(4\) 点のうち、\(3\) 点は \(1\) つの円周上にあると断言できます。
残りの \(1\) 点がその円周上にあるためには、円周角の大きさがカギになります。
\(3\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\) が同一円周上にあるとする。
(i) \(3\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\) と同じ円周上に点 \(\mathrm{Q}\) がある場合

円周角の定理から、
\(\angle \mathrm{APB} = \angle \mathrm{AQB}\)
が成り立つ。
(ii) 点 \(\mathrm{Q}\) が \(3\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\) が通る円周よりも内側にある場合
このとき、直線 \(\mathrm{AQ}\) と円との交点を \(\mathrm{Q’}\) とすると、
\(\angle \mathrm{AQ’B} = \angle \mathrm{APB} = a\)
ここで、\(\angle \mathrm{QBQ’} = b\) とおくと、三角形の外角の性質から
\(\angle \mathrm{AQB} = a + b\)
よって、点 \(\mathrm{Q}\) が 円の内側にあるときは、常に
\(\angle \mathrm{APB} < \angle \mathrm{AQB}\)
となる。
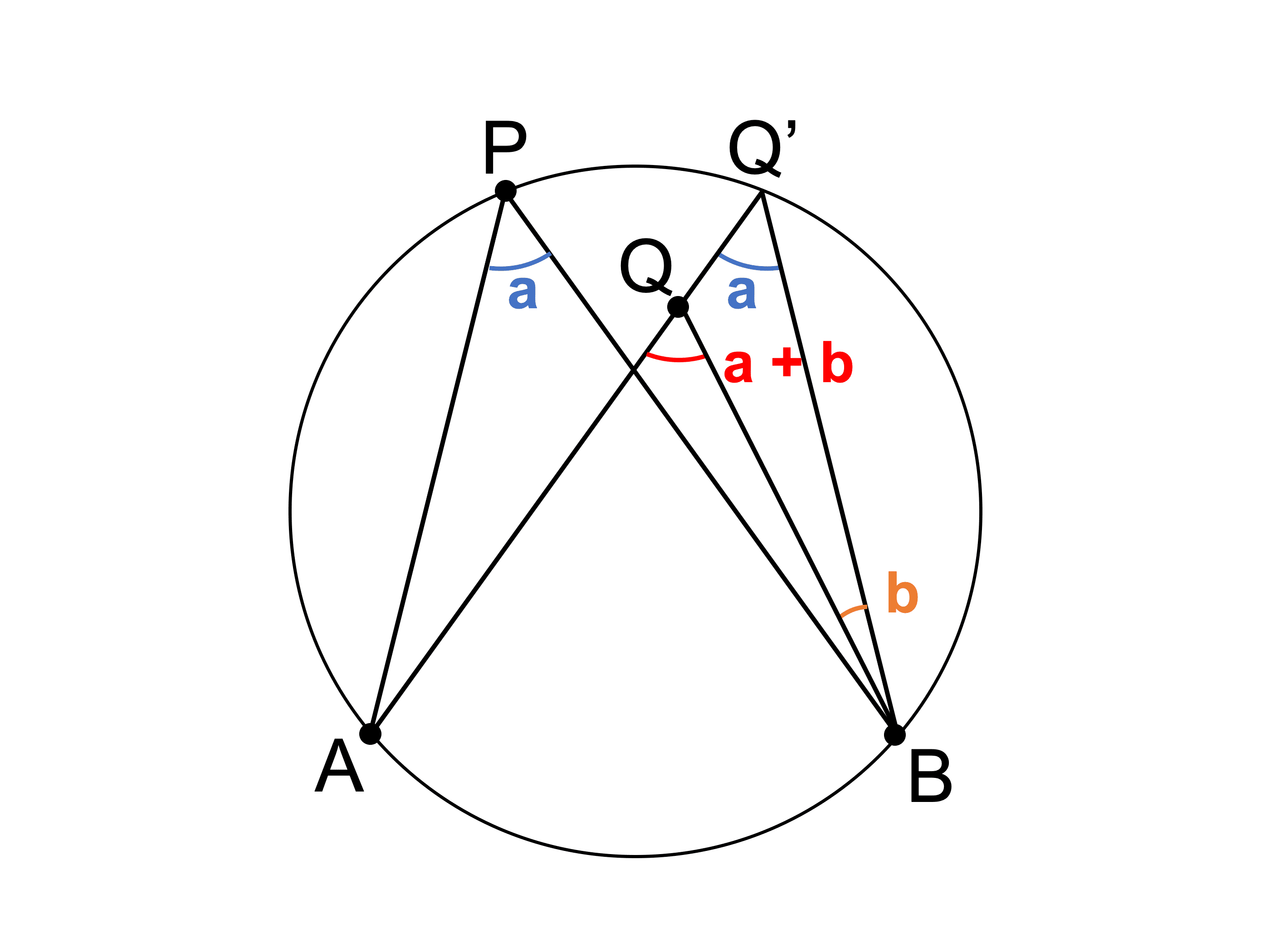
(iii) 点 \(\mathrm{Q}\) が \(3\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\) が通る円周よりも外側にある場合
直線 \(\mathrm{AQ}\) と円との交点を \(\mathrm{Q’}\) とすると
\(\angle \mathrm{AQ’B} = \angle \mathrm{APB} = a\)
三角形の外角の性質から、
\(\angle \mathrm{QBQ’} = b\) とおくと
\(\angle \mathrm{AQB} = a − b\)
よって、点 \(\mathrm{Q}\) が円の外側にあるときは、常に
\(\angle \mathrm{APB} > \angle \mathrm{AQB}\)
となる。

したがって、\(\angle \mathrm{APB} = \angle \mathrm{AQB}\) が成り立つのは、点 \(\mathrm{Q}\) が \(3\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{P}\) と同じ円周上にあるときだけである。
(証明終わり)
円周角の定理の練習問題
問題を通して、円周角の定理の使い方を確認しましょう。
計算問題①「円周角を求める」
下の図で \(\angle x\) を求めよ。


まずは、与えられた情報からすぐにわかる角度を書き出しましょう。
弧 \(\mathrm{AB}\) に対する円周角、弧 \(\mathrm{PQ}\) に対する円周角があります。
また、対頂角の関係から \(\angle \mathrm{ARP}\) も求められますね。
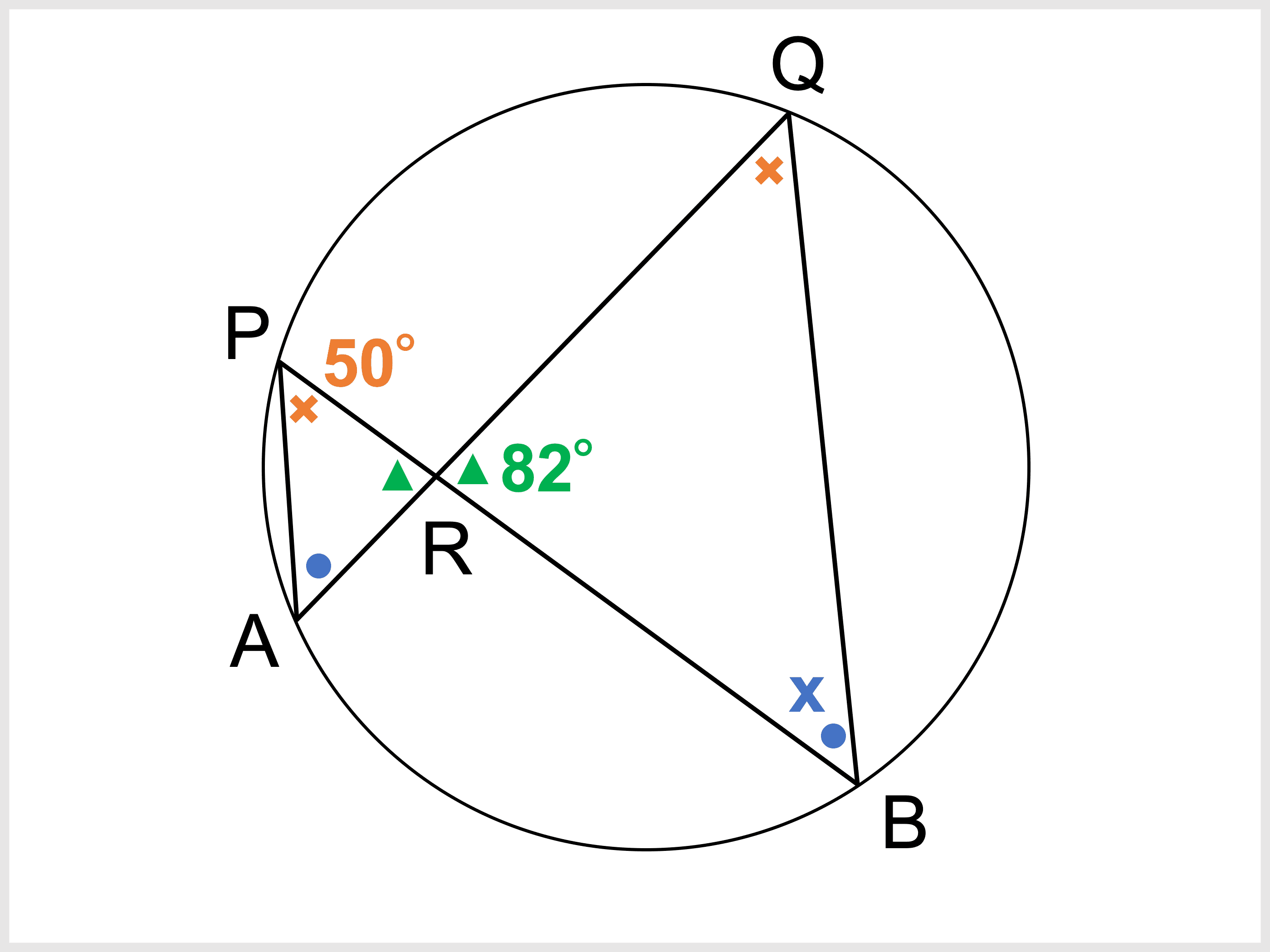
さらに、三角形の内角の和を利用して \(\angle x\) を求めましょう。
円周角の定理より、
\(\angle \mathrm{APB} = \angle \mathrm{AQB} = 50^\circ\)
\(\triangle \mathrm{BQR}\) において、内角の和の性質から
\(\begin{align} \angle x &= 180^\circ − (\angle \mathrm{RQB} + \angle \mathrm{BRQ}) \\ &= 180^\circ − (\angle \mathrm{AQB} + \angle \mathrm{BRQ}) \\ &= 180^\circ − (50^\circ + 82^\circ) \\ &= 48^\circ \end{align}\)
答え: \(\color{red}{48^\circ}\)
ちなみに、着目する三角形は \(\triangle \mathrm{APR}\) と \(\triangle \mathrm{BQR}\) のどちらでもOKですよ。
このように、円周角を求める際には、円周角の定理だけでなく、さまざまな図形の特性を利用します。
円周角の定理以外にも、よく使う特性はしっかりと理解しておきましょう。
- 対頂角、錯角、同位角
- 三角形の内角の和、外角の和
など
 図形を総まとめ!小学校〜高校で習う図形の性質・定理・公式
図形を総まとめ!小学校〜高校で習う図形の性質・定理・公式
計算問題②「円周上の \(\angle x\) を求める」
次の図で \(\angle x\) を求めよ。


円周角の定理と、二等辺三角形の底角が等しいことを利用します。
円周角の定理より、
\(\begin{align} \angle \mathrm{APB} &= \frac{1}{2} \angle \mathrm{AOB} \\ &= \frac{1}{2} \times 52^\circ \\ &= 26^\circ \end{align}\)
\(\triangle \mathrm{OBP}\) は、\(\mathrm{OB} = \mathrm{OP}\) の二等辺三角形であるから
\(\angle \mathrm{OBP} = \angle \mathrm{OPB}\)
よって
\(\begin{align} \angle x &= \angle \mathrm{OPB} \\ &= \angle \mathrm{APB} \\ &= 26^\circ \end{align}\)
答え: \(\angle x = 26^\circ\)
計算問題③「3 つの円周角を利用する」
次の図で \(\angle x\) を求めよ。
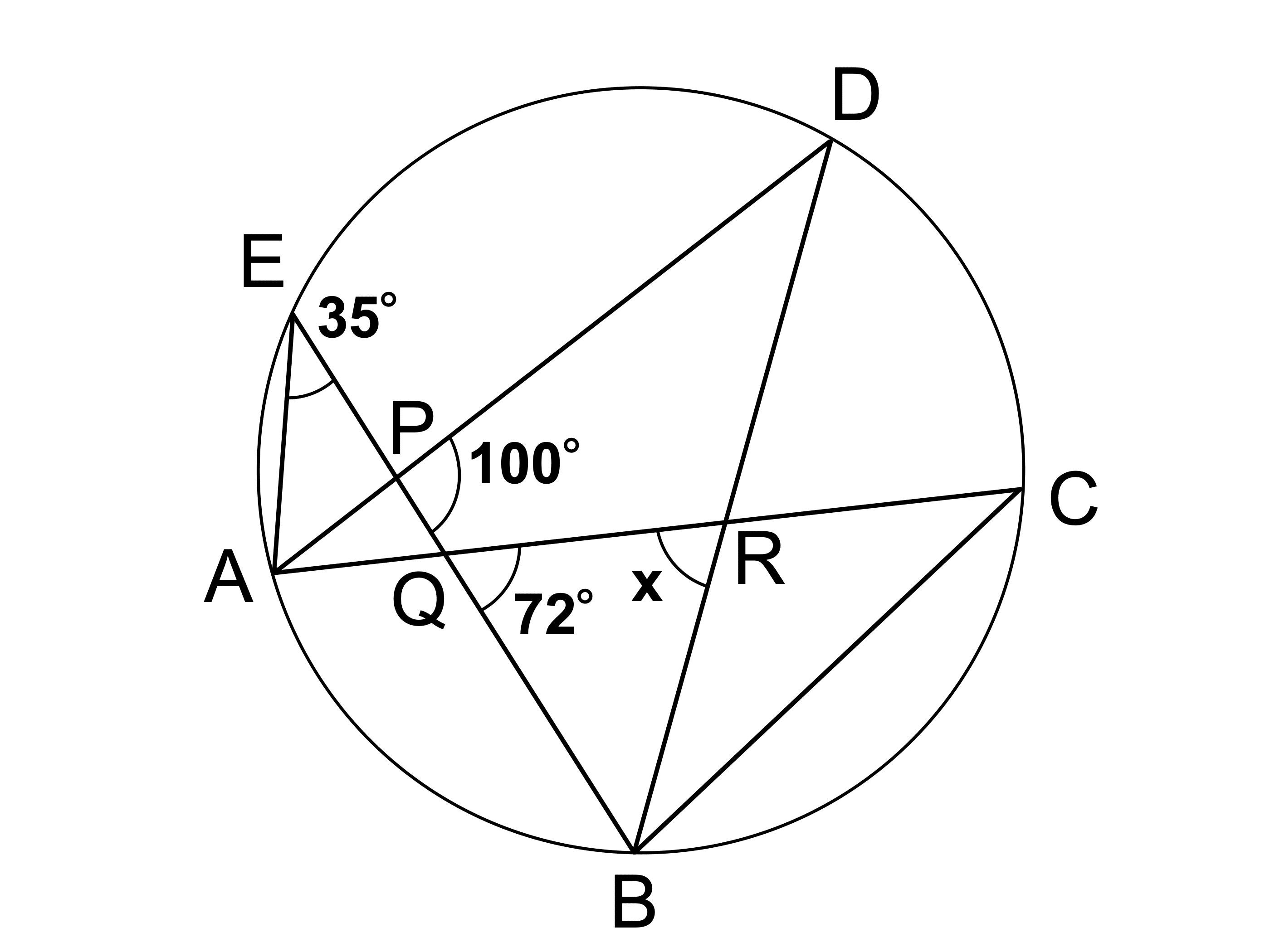

弧 \(\mathrm{AB}\) に対する円周角が \(3\) つもありますね。
対頂角や三角形の外角の性質もうまく利用しながら、目的の角度を求めましょう。
円周角の定理より
\(\begin{align} \angle \mathrm{AEB} &= \angle \mathrm{ADB} \\ &= \angle \mathrm{ACB} \\ &= 35^\circ \end{align}\)
また、対頂角は等しいので
\(\angle \mathrm{EPA} = \angle \mathrm{DPB} = 100^\circ\)
ここで、\(\angle \mathrm{AEB} = \angle \mathrm{AEP}\)、三角形の内角の和は \(180^\circ\) なので
\(\triangle \mathrm{AEP}\) において
\(\begin{align} \angle \mathrm{EAP} &= 180^\circ − (\angle \mathrm{AEP} + \angle \mathrm{EPA}) \\ &= 180^\circ − (35^\circ + 100^\circ) \\ &= 45^\circ \end{align}\)
\(\angle \mathrm{EAP} = \angle \mathrm{EAD} = 45^\circ\)
円周角の定理より
\(\angle \mathrm{EAD} = \angle \mathrm{EBD} = 45^\circ\)
\(\angle \mathrm{EBD} = \angle \mathrm{QBR} = 45^\circ\) より、
\(\triangle \mathrm{BQR}\) において
\(\begin{align} \angle x &= 180^\circ − (\angle \mathrm{BQR} + \angle \mathrm{QBR}) \\ &= 180^\circ − (72^\circ + 45^\circ) \\ &= 63^\circ \end{align}\)
答え: \(\angle x = 63^\circ\)
証明問題「4 点が同一円周上にあることを証明する」
次の図の \(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\)、\(\mathrm{D}\) に対し、直線 \(\mathrm{AB}\) と直線 \(\mathrm{CD}\) が平行であり、直線 \(\mathrm{AC}\) と直線 \(\mathrm{BD}\) の交点を \(\mathrm{E}\) とする。\(\mathrm{CE} = \mathrm{DE}\) であるとき、\(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\)、\(\mathrm{D}\) は \(1\) つの円周上にあることを示せ。
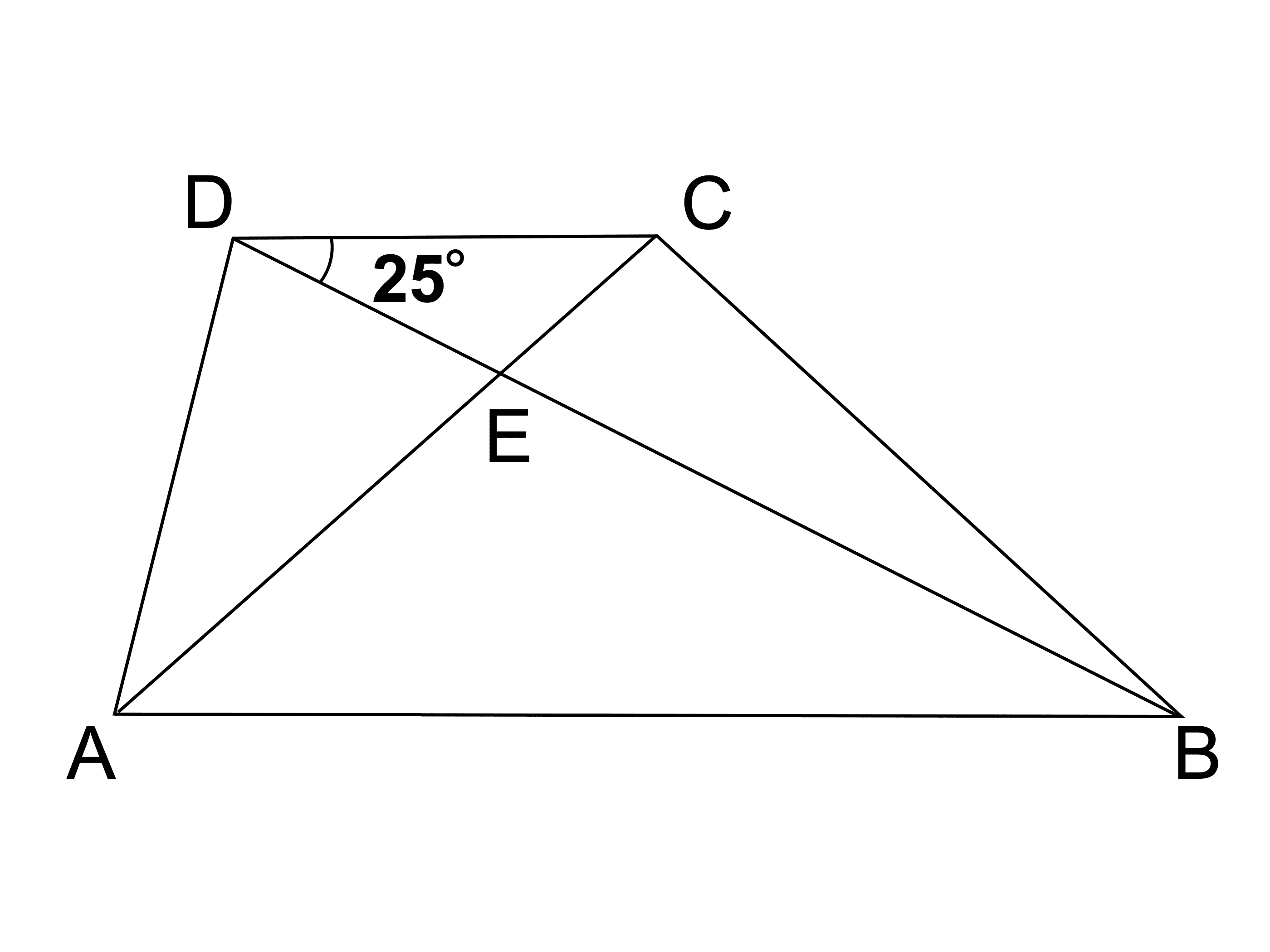

\(\angle \mathrm{ACD} = \angle \mathrm{ABD}\) が成り立てば、円周角の定理の逆から \(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\)、\(\mathrm{D}\) が \(1\) つの円周上にあることがいえます。
\(\mathrm{CE} = \mathrm{DE}\) がキモですね。
\(\mathrm{AB} \ // \ \mathrm{CD}\) より、錯角は等しいので
\(\angle \mathrm{ABD} = \angle \mathrm{CDB} = 25^\circ\) …①
また、\(\triangle \mathrm{CDE}\) は \(\mathrm{CE} = \mathrm{DE}\) の二等辺三角形であるから、
\(\angle \mathrm{ECD} = \angle \mathrm{EDC} = 25^\circ\)
である。
ここで、
\(\angle \mathrm{ACD} = \angle \mathrm{ECD} = 25^\circ\) …②
①、②より
\(\angle \mathrm{ACD} = \angle \mathrm{ABD} = 25^\circ\)
このことと、\(4\) 点 \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\)、\(\mathrm{D}\) について、点 \(\mathrm{B}\)、\(\mathrm{C}\) は直線 \(\mathrm{AD}\) に対して同じ側にあることから、円周角の定理の逆が成り立つ。
したがって、\(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\)、\(\mathrm{D}\) は \(1\) つの円周上に存在する。
(証明終わり)
以上で問題も終わりです!
円周角の定理は円にまつわる角度を求めるときに非常に便利な定理です。
円周角の定理を味方につけて、図形問題を楽々解けるようになりましょう!
