この記事では「二倍角の公式」について、語呂合わせによる覚え方や証明方法(導き方)、問題の解き方をわかりやすく解説していきます。
二倍角の公式は加法定理から簡単に導けるので、ぜひマスターしましょう!
目次
二倍角の公式とは?
二倍角の公式とは、ある角の \(2\) 倍の角度の三角関数の値を求める公式です。
- 正弦(sin)
\begin{align}\color{red}{\sin2\theta = 2\sin\theta\cos\theta}\end{align} - 余弦(cos)
\begin{align} \color{red}{\cos2\theta \ } &\color{red}{= \cos^2\theta − \sin^2\theta} \\ &\color{red}{= 1 − 2\sin^2\theta} \\ &\color{red}{= 2\cos^2\theta − 1} \end{align} - 正接(tan)
\begin{align}\color{red}{\displaystyle \tan2\theta = \frac{2\tan\theta}{1 − \tan^2\theta}}\end{align}
\(2\theta\)(\(\theta\) の \(2\) 倍)の三角関数を \(\theta\) の三角関数で表すことができていますね。
\(\cos\) の二倍角の公式は、三角比の相互関係 \(\sin^2\theta + \cos^2\theta = 1\) から \(3\) 通りの表し方ができます。
二倍角の公式の覚え方【語呂合わせ】
ここでは、二倍角の公式の語呂合わせによる覚え方を紹介します。
なお、二倍角の公式は加法定理から一瞬で導出できるため、わざわざ語呂で覚えるのはオススメしません(→ 二倍角の公式の導き方【証明】)。
- \(\sin\) の二倍角公式
菜園に咲いたコスモス
\(\color{skyblue}{\sin2\theta} = \color{red}{2}\color{skyblue}{\sin\theta}\color{limegreen}{\cos\theta}\)
- \(\cos\) の二倍角公式
こす兄はこす爺信じない
\(\color{limegreen}{\cos2\theta} = \color{limegreen}{\cos^2\theta} \color{red}{−} \color{skyblue}{\sin^2\theta}\)
- \(\tan\) の二倍角公式
単に 1 マイタンジ、2 タン
\(\displaystyle \color{orange}{\tan2\theta} = \frac{\color{limegreen}{2\tan\theta}}{\color{red}{1 − \tan^2\theta}}\)
二倍角の公式の導き方【証明】
二倍角の公式は、加法定理において \(\alpha\) と \(\beta\) を同じ角度 \((= \theta)\) とするだけで簡単に導くことができます。
加法定理
- \(\sin(\alpha + \beta) = \sin\alpha\cos\beta + \cos\alpha\sin\beta\)
- \(\cos(\alpha + \beta) = \cos\alpha\cos\beta − \sin\alpha\sin\beta\)
- \(\displaystyle \tan(\alpha + \beta) = \frac{\tan\alpha + \tan\beta}{1 − \tan\alpha\tan\beta}\)
sin の二倍角の公式の証明
加法定理より、
\(\begin{align}\color{red}{\sin2\theta \ } &= \sin(\theta + \theta) \\&= \sin\theta\cos\theta + \cos\theta\sin\theta \\&\color{red}{= 2\sin\theta\cos\theta}\end{align}\)
(証明終わり)
cos の二倍角の公式の証明
加法定理より、
\(\begin{align}\color{red}{\cos2\theta \ } &= \cos(\theta + \theta) \\&= \cos\theta\cos\theta − \sin\theta\sin\theta \\&\color{red}{= \cos^2\theta − \sin^2\theta}\end{align}\)
ここで、三角比の相互関係 \(\sin^2\theta + \cos^2\theta = 1\) から
\(\cos^2\theta = 1 − \sin^2\theta\) …①
\(\sin^2\theta = 1 − \cos^2\theta\) …②
①を \(\cos2\theta = \cos^2\theta − \sin^2\theta\) に代入して、
\(\begin{align} \color{red}{\cos2\theta \ } &= (1 − \sin^2\theta) − \sin^2\theta \\&\color{red}{= 1 − 2\sin^2\theta} \end{align}\)
②を \(\cos2\theta = \cos^2\theta − \sin^2\theta\) に代入して、
\(\begin{align} \color{red}{\cos2\theta \ } &= \cos^2\theta − (1 − \cos^2\theta) \\&\color{red}{= 2\cos^2\theta − 1} \end{align}\)
(証明終わり)
tan の二倍角の公式の証明
加法定理より、
\(\begin{align} \color{red}{\tan2\theta \ } &= \tan(\theta + \theta) \\&= \frac{\tan\theta + \tan\theta}{1 − \tan\theta\tan\theta} \\&\color{red}{=\frac{2\tan\theta}{1 − \tan^2\theta}} \end{align}\)
(証明終わり)
加法定理の角度を書き換えるだけなので、慣れれば一瞬で導けます。
三角関数はただでさえ公式が多いので、無理に丸暗記しないことも大切ですよ。
二倍角の公式の使い方
二倍角の公式は、次のような場面で役に立ちます。
- 値が既知の三角関数を使って、その二倍角の三角関数の値を求めたい
→ 練習問題①、練習問題② - 三角関数の種類や角度をそろえたり、次数を下げたりしたい
(三角関数の方程式・不等式・微分積分の問題など)
→ 練習問題③
対応する練習問題を解いて、二倍角の公式の使い方をマスターしましょう。
練習問題①「sin α から 2α の三角関数を求める」
\(\displaystyle \sin\alpha = −\frac{1}{3}\) のとき、\(\sin2\alpha\), \(\cos2\alpha\), \(\tan2\alpha\) を求めよ。ただし、\(\displaystyle \frac{3}{2}\pi < \alpha < 2\pi\) とする。

まず \(\cos\alpha\) の値を求め、それから二倍角の公式を使います。
\(\alpha\) の範囲に注意して、符号を間違えないようにしましょう。
\(\sin^2\alpha + \cos^2\alpha = 1\) より、
\(\begin{align} \cos^2\alpha &= 1 − \sin^2\alpha \\ &= 1 − \left( −\frac{1}{3} \right)^2 \\ &= 1 − \frac{1}{9} \\ &= \frac{8}{9} \end{align}\)
\(\displaystyle \frac{3}{2}\pi < \alpha < 2\pi\) より、 \(\cos\alpha > 0\) であるから
\(\displaystyle \cos\alpha = +\sqrt{\frac{8}{9}} = \frac{2\sqrt{2}}{3}\)
二倍角の公式より、
\(\begin{align} \sin2\alpha &= 2\sin\alpha\cos\alpha \\ &= 2 \left( −\frac{1}{3} \right) \cdot \frac{2\sqrt{2}}{3} \\ &= −\frac{4\sqrt{2}}{9} \end{align}\)
二倍角の公式より、
\(\begin{align} \cos2\alpha &= 1 − 2\sin^2\alpha \\ &= 1 − 2 \left( −\frac{1}{3} \right)^2 \\ &= 1 − \frac{2}{9} \\ &= \frac{7}{9} \end{align}\)
\(\begin{align} \tan2\alpha &= \frac{\sin2\alpha}{\cos2\alpha} \\ &= \frac{−\frac{4\sqrt{2}}{9}}{\frac{7}{9}} \\ &= −\frac{4\sqrt{2}}{7} \end{align}\)
答え:
\(\color{red}{\displaystyle \sin2\alpha = −\frac{4\sqrt{2}}{9}}\)
\(\color{red}{\displaystyle \cos2\alpha = \frac{7}{9}}\)
\(\color{red}{\displaystyle \tan2\alpha = −\frac{4\sqrt{2}}{7}}\)
練習問題②「tan θ から tan 2θ を求める」
\(\displaystyle \frac{\pi}{2} < \theta < \pi\)、\(\displaystyle \tan\theta = −\frac{2}{\sqrt{3}}\) のとき、\(\tan2\theta\) を求めよ。

\(\tan\) の二倍角の公式で、簡単に求められますね。
二倍角の公式より、
\(\begin{align}\displaystyle \tan2\theta &= \frac{2\tan\theta}{1 − \tan^2\theta}\\ &= \frac{2 \left( −\frac{2}{\sqrt{3}} \right)}{1 − \left( −\frac{2}{\sqrt{3}} \right)^2}\\ &= \frac{−\frac{4}{\sqrt{3}}}{1 − \frac{4}{3}}\\ &= \frac{−\frac{4}{\sqrt{3}}}{−\frac{1}{3}}\\ &= −\frac{4}{\sqrt{3}} \cdot (−3)\\&= 4\sqrt3\end{align}\)
答え: \(\color{red}{\tan2\theta = 4\sqrt{3}}\)
練習問題③「cos θ と cos 2θ の不等式を解く」
\(0 \leq \theta < 2\pi\) のとき、\(\cos2\theta \leq \sqrt{3} \cos\theta + 2\) を解け。

\(\cos\) の二倍角の公式を使って、不等式の変数が \(\cos\theta\) だけになるように式変形するのがポイントです。
\(\cos\theta\) のとりうる範囲は
\(−1 \leq \cos\theta \leq 1\) …①
\(\cos2\theta \leq \sqrt{3} \cos\theta + 2\) において、
二倍角の公式より
\(2\cos^2\theta − 1 \leq \sqrt{3} \cos\theta + 2\)
\(2\cos^2\theta − \sqrt{3} \cos\theta − 3 \leq 0\)
\((\cos\theta − \sqrt{3})(2\cos\theta + \sqrt{3}) \leq 0\)
\(\displaystyle −\frac{\sqrt{3}}{2} \leq \cos\theta \leq \sqrt{3}\)
①との共通範囲は
\(\displaystyle −\frac{\sqrt{3}}{2} \leq \cos\theta \leq 1\)
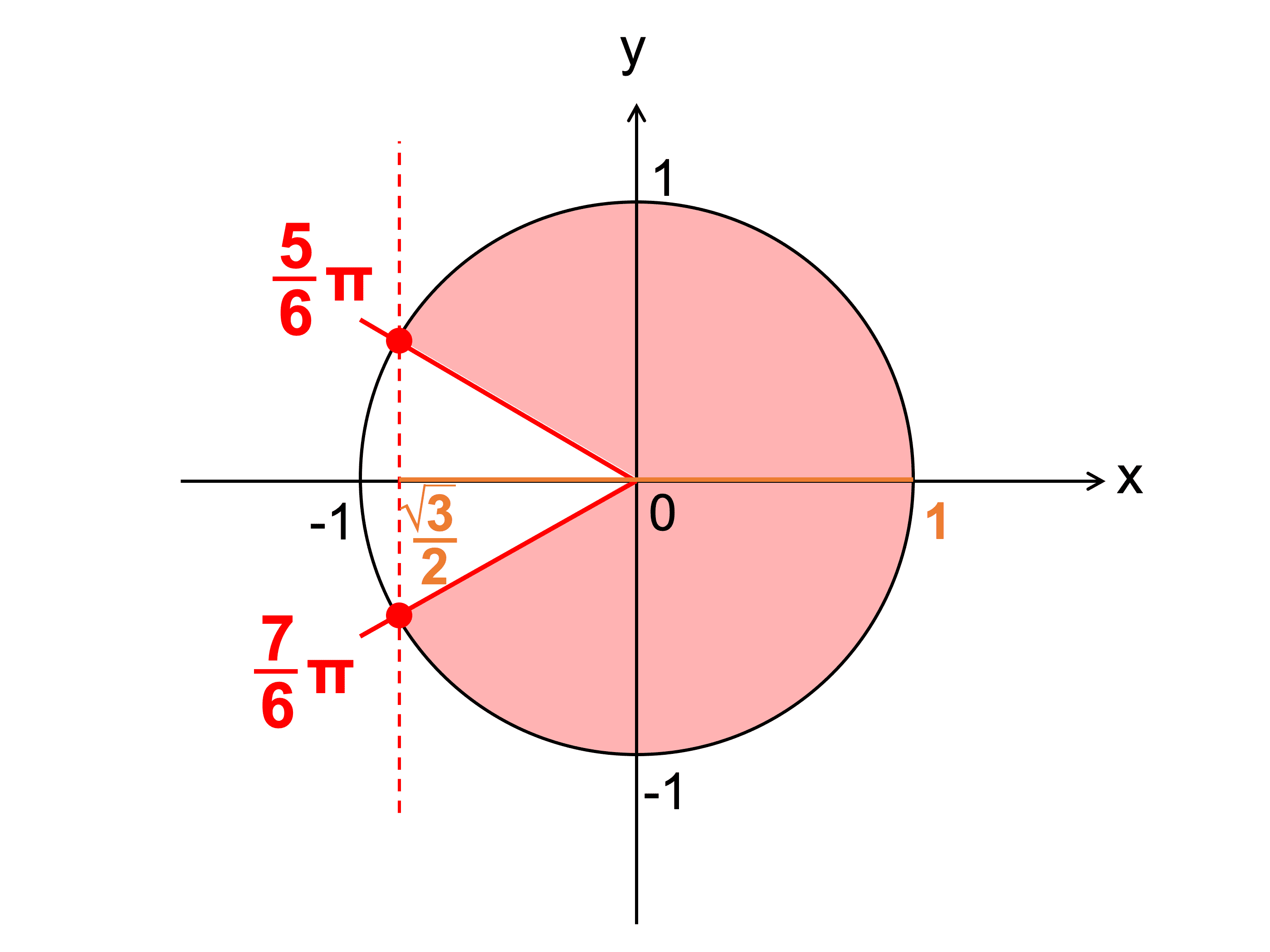
よって、
\(\displaystyle 0 \leq \theta \leq \frac{5}{6}\pi, \displaystyle \frac{7}{6}\pi \leq \theta < 2\pi\)
答え: \(\color{red}{\displaystyle 0 \leq \theta \leq \frac{5}{6}\pi, \displaystyle \frac{7}{6}\pi \leq \theta < 2\pi}\)
以上で二倍角の公式の解説は終わりです。
二倍角の公式はよく使うので、繰り返し練習してマスターしてくださいね。
三角関数に関するほかの定理や公式について調べたい方は、以下のまとめ記事から探してみてください!
 三角比・三角関数を総まとめ!定義・定理・公式一覧
三角比・三角関数を総まとめ!定義・定理・公式一覧

