三角比・三角関数の定義や高校で習うすべての定理と公式をまとめていきます。
詳細記事へのリンクも載せていますので、ぜひ勉強の参考にしてくださいね!
目次
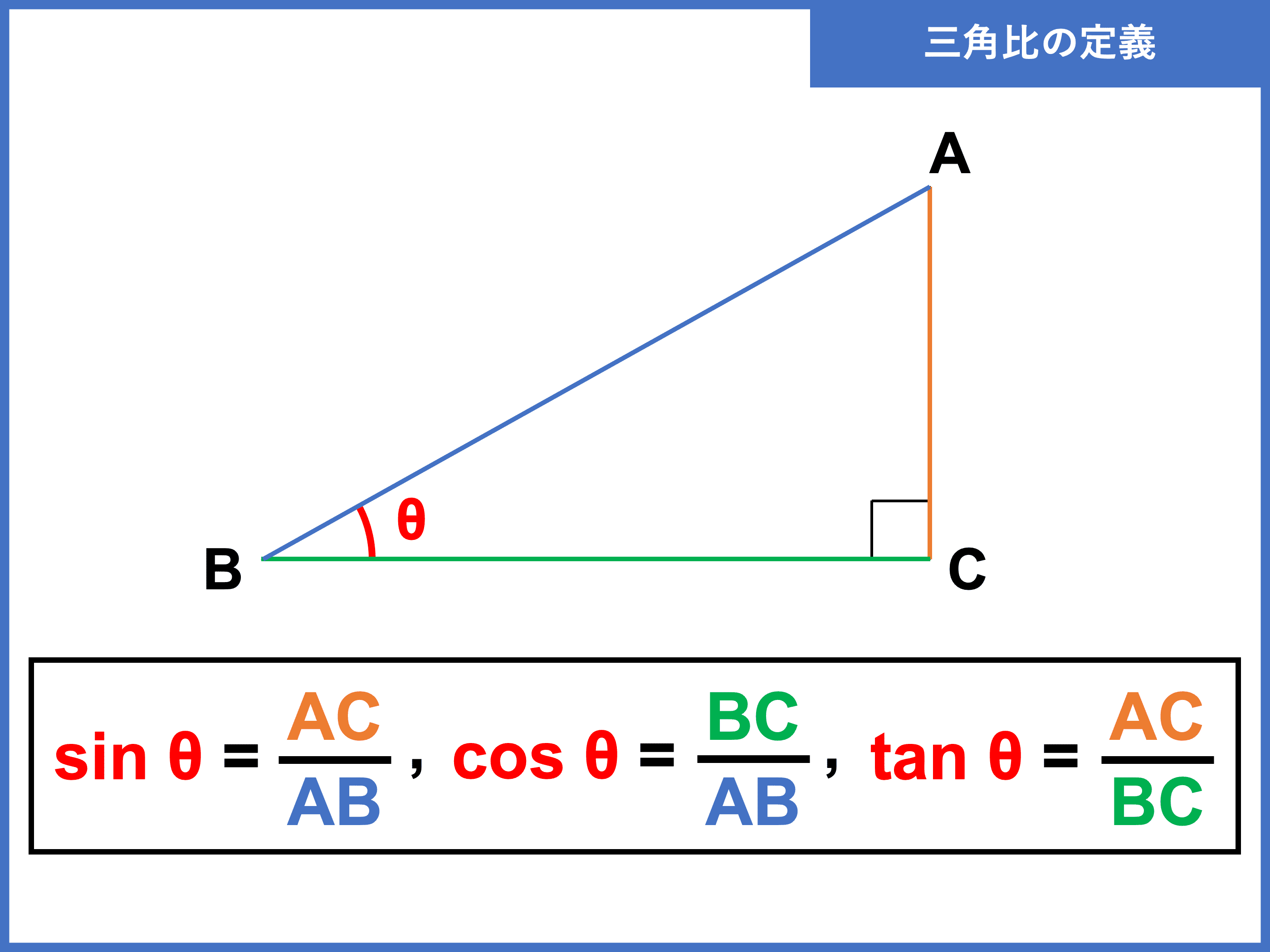
三角比の定義
三角比は、直角三角形の鋭角に対する \(2\) 辺の比として定義されます。
\(\angle \mathrm{C} = 90^\circ\) の直角三角形 \(\triangle{\mathrm{ABC}}\) において、
- \(\sin \theta = \displaystyle \frac{\mathrm{AC}}{\mathrm{AB}}\)
- \(\cos \theta = \displaystyle \frac{\mathrm{BC}}{\mathrm{AB}}\)
- \(\tan \theta = \displaystyle \frac{\mathrm{AC}}{\mathrm{BC}}\)
三角比については、以下の記事で詳しく説明しています。
 三角比とは?定義や三角比の相互関係をわかりやすく解説!
三角比とは?定義や三角比の相互関係をわかりやすく解説!
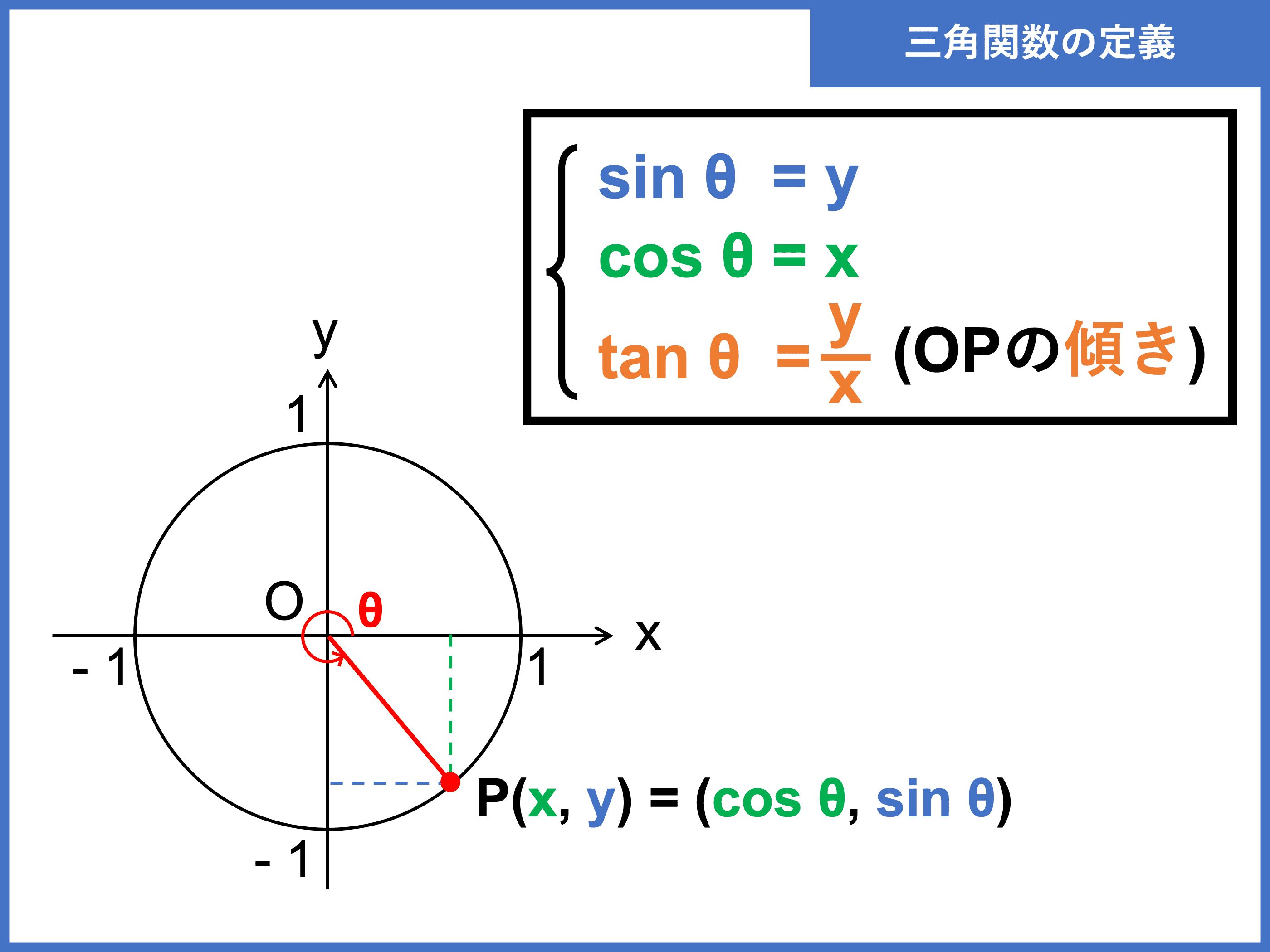
三角関数の定義(三角比の拡張)
三角比を単位円周上を回転する点の座標として定義しなおし、任意の実数をとる角度に対して定義した関数を「三角関数」といいます。
\(x\) 軸の正の部分を始線とした一般角 \(\theta\) の動径と、単位円との交点を \(\mathrm{P} = (x, y)\) とすると、
- \(\sin \theta = y\)
- \(\cos \theta = x\)
- \(\tan \theta = \displaystyle \frac{y}{x}\)
以下の記事では、単位円を使って主要な三角比の値を求める方法、および、三角関数表(教科書の巻末にある三角比の表)を読み取って三角比を求める方法を説明しています。
 三角関数表(1°ごとの三角比の表)と有名角の三角比の求め方!
三角関数表(1°ごとの三角比の表)と有名角の三角比の求め方!
三角比の相互関係
三角比(三角関数)には、以下の関係式が成り立ちます。
- \(\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}\)
- \(\displaystyle \sin^2 \theta + \cos^2 \theta = 1\)
- \(\displaystyle 1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}\)
度数とラジアンの変換
角度は、度数法(\(^\circ\))と弧度法(\(\mathrm{rad}\))の \(2\) 通りの表し方ができます。
- 度数 → 弧度(ラジアン)
\begin{align}\theta \ (^\circ) = \theta \times \displaystyle \frac{\pi}{180} \ \text{(rad)}\end{align} - 弧度(ラジアン) → 度数
\begin{align}\theta \ \text{(rad)} = \theta \times \displaystyle \frac{180}{\pi} \ (^\circ)\end{align}
度数法と弧度法の変換については以下の記事で説明しています。
 ラジアンとは?弧度法とは?定義や角度変換をわかりやすく解説
ラジアンとは?弧度法とは?定義や角度変換をわかりやすく解説
三角関数の変換公式
三角関数は、その対称性から別の三角関数に変換することができます。
- 度数
\begin{align}\sin (90^\circ − \theta) &= \cos \theta \\ \cos (90^\circ − \theta) &= \sin \theta \\ \tan (90^\circ − \theta) &= \frac{1}{\tan \theta}\end{align} - 弧度 (ラジアン)
\begin{align}\sin \left( \frac{\pi}{2} − \theta \right) &= \cos \theta \\ \cos \left( \frac{\pi}{2} − \theta \right) &= \sin \theta \\ \tan \left( \frac{\pi}{2} − \theta \right) &= \frac{1}{\tan \theta}\end{align}
- 度数
\begin{align}\sin (90^\circ + \theta) &= \cos \theta \\ \cos (90^\circ + \theta) &= −\sin \theta \\ \tan (90^\circ + \theta) &= −\frac{1}{\tan \theta}\end{align} - 弧度 (ラジアン)
\begin{align}\sin \left( \frac{\pi}{2} + \theta \right) &= \cos \theta \\ \cos \left( \frac{\pi}{2} + \theta \right) &= −\sin \theta \\ \tan \left( \frac{\pi}{2} + \theta \right) &= −\frac{1}{\tan \theta}\end{align}
- 度数
\begin{align}\sin (180^\circ − \theta) &= \sin \theta \\ \cos (180^\circ − \theta) &= −\cos \theta \\ \tan (180^\circ − \theta) &= −\tan \theta\end{align} - 弧度 (ラジアン)
\begin{align}\sin (\pi − \theta) &= \sin \theta \\ \cos (\pi − \theta) &= −\cos \theta \\ \tan (\pi − \theta) &= −\tan \theta\end{align}
- 度数
\begin{align}\sin (180^\circ + \theta) &= −\sin \theta \\ \cos (180^\circ + \theta) &= −\cos \theta \\ \tan (180^\circ + \theta) &= \tan \theta\end{align} - 弧度 (ラジアン)
\begin{align}\sin (\pi + \theta) &= −\sin \theta \\ \cos (\pi + \theta) &= −\cos \theta \\ \tan (\pi + \theta) &= \tan \theta\end{align}
- 度数
\begin{align}\sin (− \theta) &= −\sin \theta \\ \cos (− \theta) &= \cos \theta \\ \tan (− \theta) &= −\tan \theta\end{align} - 弧度 (ラジアン)
\begin{align}\sin (− \theta) &= −\sin \theta \\ \cos (− \theta) &= \cos \theta \\ \tan (− \theta) &= −\tan \theta\end{align}
以下の記事では、三角関数の変換公式の使い方、および、三角関数の値から角度を求める方法について説明しています。
 三角関数の角度の求め方と変換公式をわかりやすく解説!
三角関数の角度の求め方と変換公式をわかりやすく解説!
正弦定理・余弦定理
正弦定理と余弦定理の使い分けについては、以下の記事で説明しています。
 正弦定理と余弦定理の使い分けを徹底解説!練習問題も
正弦定理と余弦定理の使い分けを徹底解説!練習問題も
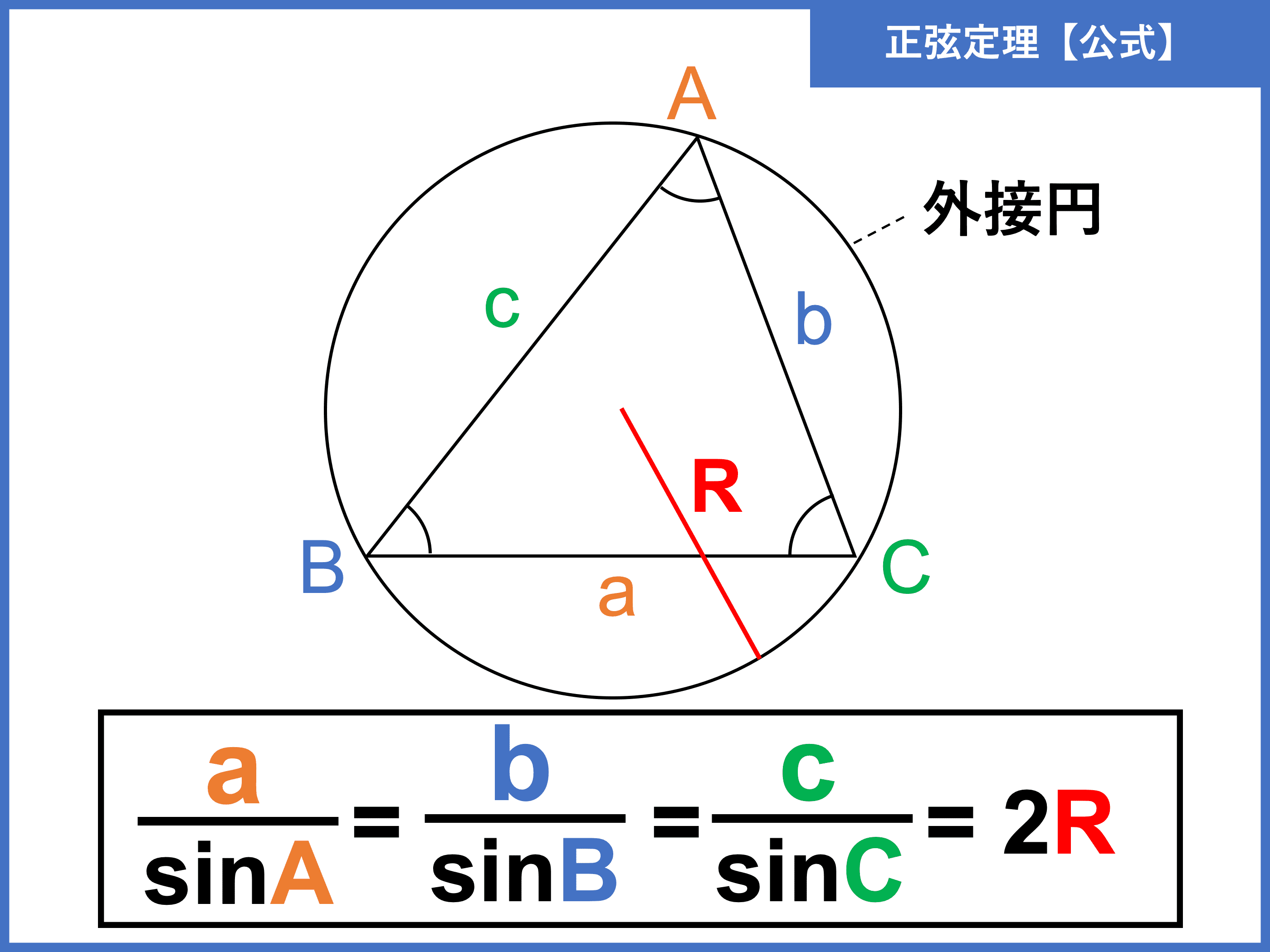
正弦定理
\(\triangle \mathrm{ABC}\) と、その外接円(半径 \(r\))について、
\begin{align}\frac{a}{\sin \mathrm{A}} = \frac{b}{\sin \mathrm{B}} = \frac{c}{\sin \mathrm{C}} = 2R\end{align}
 正弦定理とは?公式や証明、計算問題をわかりやすく解説
正弦定理とは?公式や証明、計算問題をわかりやすく解説
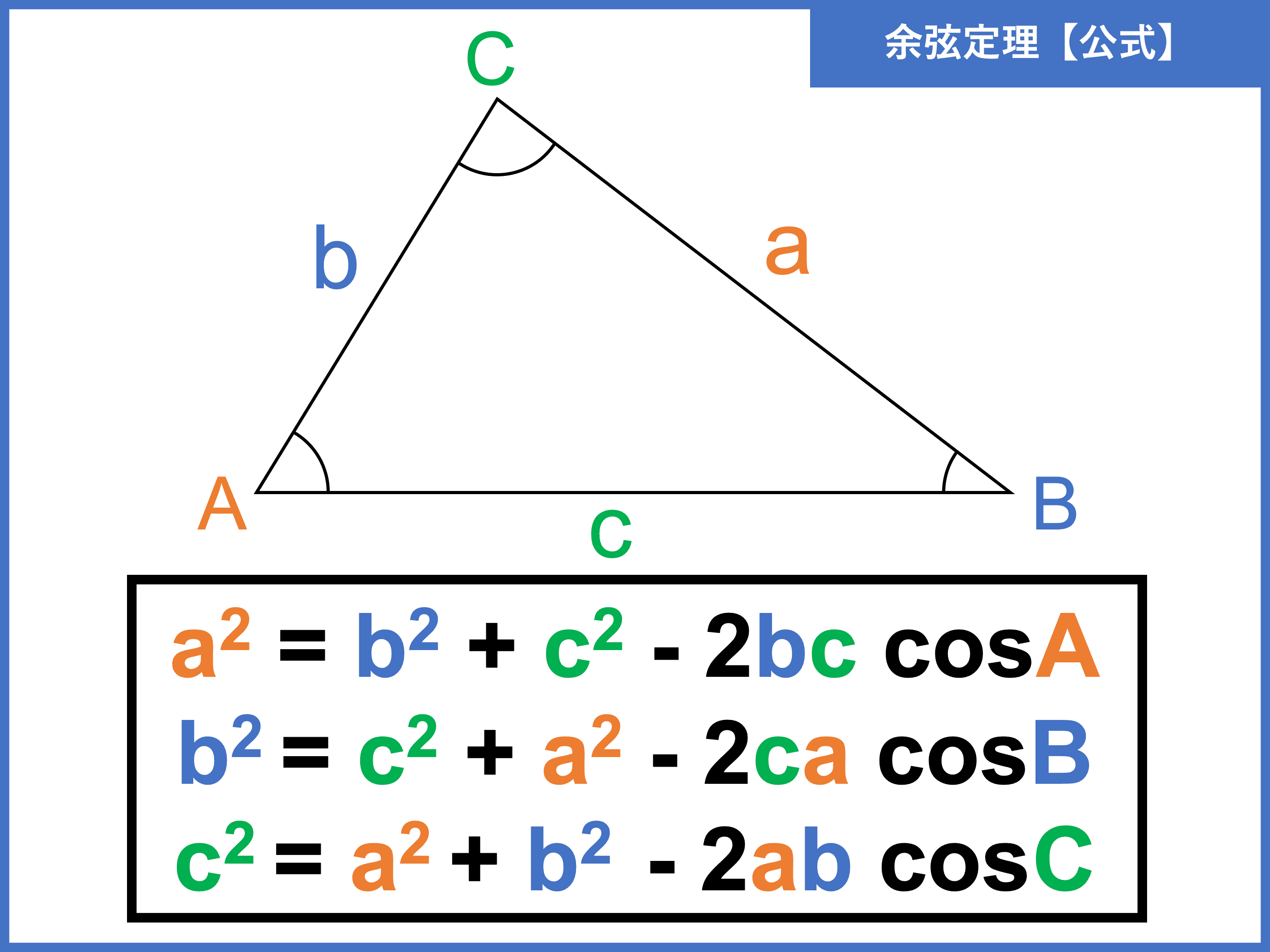
余弦定理
\(\triangle \mathrm{ABC}\) について、
\begin{align}a^2 = b^2 + c^2 − 2bc \cos \mathrm{A}\end{align}
\begin{align}b^2 = c^2 + a^2 − 2ca \cos \mathrm{B}\end{align}
\begin{align}c^2 = a^2 + b^2 − 2ab \cos \mathrm{C}\end{align}
 余弦定理とは?公式の覚え方や証明、計算問題の解き方
余弦定理とは?公式の覚え方や証明、計算問題の解き方
三角形への応用
三角比を用いて、三角形の面積や外接円・内接円の半径を求めることができます。
三角比を用いた三角形の面積公式
三角形の \(2\) 辺の長さを \(a\), \(b\)、その間の角を \(\theta\) とおくと、三角形の面積 \(S\) は
\begin{align}\displaystyle S = \frac{1}{2} ab \sin \theta\end{align}
 三角形の面積公式まとめ!求め方をわかりやすく解説
三角形の面積公式まとめ!求め方をわかりやすく解説
ヘロンの公式(三角形の面積)
三角形の面積公式 \(\displaystyle S = \frac{1}{2} ab \sin \theta\) を余弦定理で変形すると、もう \(1\) つの面積公式、ヘロンの公式が導けます。
三角形の \(3\) 辺をそれぞれ \(a\), \(b\), \(c\)、三角形の面積を \(S\) とすると、
\begin{align}S = \sqrt{s(s − a)(s − b)(s − c)}\end{align}
ただし、\(\displaystyle s = \frac{a + b + c}{2}\)
 ヘロンの公式とは?証明や四角形版もわかりやすく解説!
ヘロンの公式とは?証明や四角形版もわかりやすく解説!
三角形の外接円の半径
正弦定理や三角形の面積から、三角形に外接する円の半径が導けます。
\(\triangle \mathrm{ABC}\) の \(3\) 辺の長さを \(a\), \(b\), \(c\)、面積を \(S\)、その外接円の半径を \(R\) とすると、
- \(\displaystyle R = \frac{a}{2\sin \mathrm{A}} = \frac{b}{2\sin \mathrm{B}} = \frac{c}{2\sin \mathrm{C}}\)(正弦定理)
- \(\displaystyle R = \frac{abc}{4S}\)
 外接円とは?半径の公式や求め方、性質をわかりやすく解説!
外接円とは?半径の公式や求め方、性質をわかりやすく解説!
三角形の内接円の半径
三角形の面積から、三角形に内接する円の半径が導けます。
\(\triangle \mathrm{ABC}\) の \(3\) 辺の長さを \(a\), \(b\), \(c\)、面積を \(S\)、その内接円の半径を \(r\) とすると、
\begin{align}\displaystyle r = \frac{2S}{a + b + c}\end{align}
 内接円とは?半径の公式や求め方、性質をわかりやすく解説!
内接円とは?半径の公式や求め方、性質をわかりやすく解説!
加法定理
三角関数の計算において最重要な定理です。
- \(\sin (\alpha \pm \beta) = \sin \alpha \cos \beta \pm \cos \alpha \sin \beta\)
- \(\cos (\alpha \pm \beta) = \cos \alpha \cos \beta \mp \sin \alpha \sin \beta\)
- \(\displaystyle \tan (\alpha \pm \beta) = \frac{\tan \alpha \pm \tan \beta}{1 \mp \tan \alpha \tan \beta}\)
(複合同順)
 加法定理とは?覚え方や証明、応用問題をわかりやすく解説
加法定理とは?覚え方や証明、応用問題をわかりやすく解説
倍角の公式
\(n\theta\) の三角関数を \(\theta\) の三角関数で表せる公式を「\(n\) 倍角の公式」といいます。
二倍角の公式
- \(\sin2\theta = 2\sin\theta\cos\theta\)
- \(\begin{align} \cos2\theta &= \cos^2\theta − \sin^2\theta \\ &= 1 − 2\sin^2\theta \\ &= 2\cos^2\theta − 1 \end{align}\)
- \(\displaystyle \tan2\theta = \frac{2\tan\theta}{1 − \tan^2\theta}\)
 二倍角の公式の覚え方(語呂合わせ)と証明、問題での使い方
二倍角の公式の覚え方(語呂合わせ)と証明、問題での使い方
三倍角の公式
- \(\sin3\theta = 3\sin\theta − 4\sin^3\theta\)
- \(\cos3\theta = −3\cos\theta + 4\cos^3\theta\)
 三倍角の公式の覚え方(語呂合わせ)と証明、問題での使い方
三倍角の公式の覚え方(語呂合わせ)と証明、問題での使い方
半角の公式
- \(\displaystyle \sin^2 \frac{\theta}{2} = \frac{1 − \cos\theta}{2}\)
- \(\displaystyle \cos^2 \frac{\theta}{2} = \frac{1 + \cos\theta}{2}\)
- \(\displaystyle \tan^2 \frac{\theta}{2} = \frac{1 − \cos\theta}{1 + \cos\theta}\)
 半角の公式の覚え方(語呂合わせ)と証明、問題での使い方
半角の公式の覚え方(語呂合わせ)と証明、問題での使い方
積和の公式・和積の公式
三角関数の積を和に、和を積に変換する公式です。
 積和の公式・和積の公式とは?覚え方(語呂合わせ)や証明
積和の公式・和積の公式とは?覚え方(語呂合わせ)や証明
積和の公式
- \(\displaystyle \sin\alpha\cos\beta = \frac{1}{2} \{\sin(\alpha + \beta) + \sin(\alpha − \beta)\}\)
- \(\displaystyle \cos\alpha\sin\beta = \frac{1}{2} \{\sin(\alpha + \beta) − \sin(\alpha − \beta)\}\)
- \(\displaystyle \cos\alpha\cos\beta = \frac{1}{2} \{\cos(\alpha + \beta) + \cos(\alpha − \beta)\}\)
- \(\displaystyle \sin\alpha\sin\beta = −\frac{1}{2} \{\cos(\alpha + \beta) − \cos(\alpha − \beta)\}\)
(見切れる場合は横へスクロール)
和積の公式
- \(\displaystyle \sin A + \sin B = 2\sin\frac{A + B}{2}\cos\frac{A − B}{2}\)
- \(\displaystyle \sin A − \sin B = 2\cos\frac{A + B}{2}\sin\frac{A − B}{2}\)
- \(\displaystyle \cos A + \cos B = 2\cos\frac{A + B}{2}\cos\frac{A − B}{2}\)
- \(\displaystyle \cos A − \cos B = −2\sin\frac{A + B}{2}\sin\frac{A − B}{2}\)
(見切れる場合は横へスクロール)
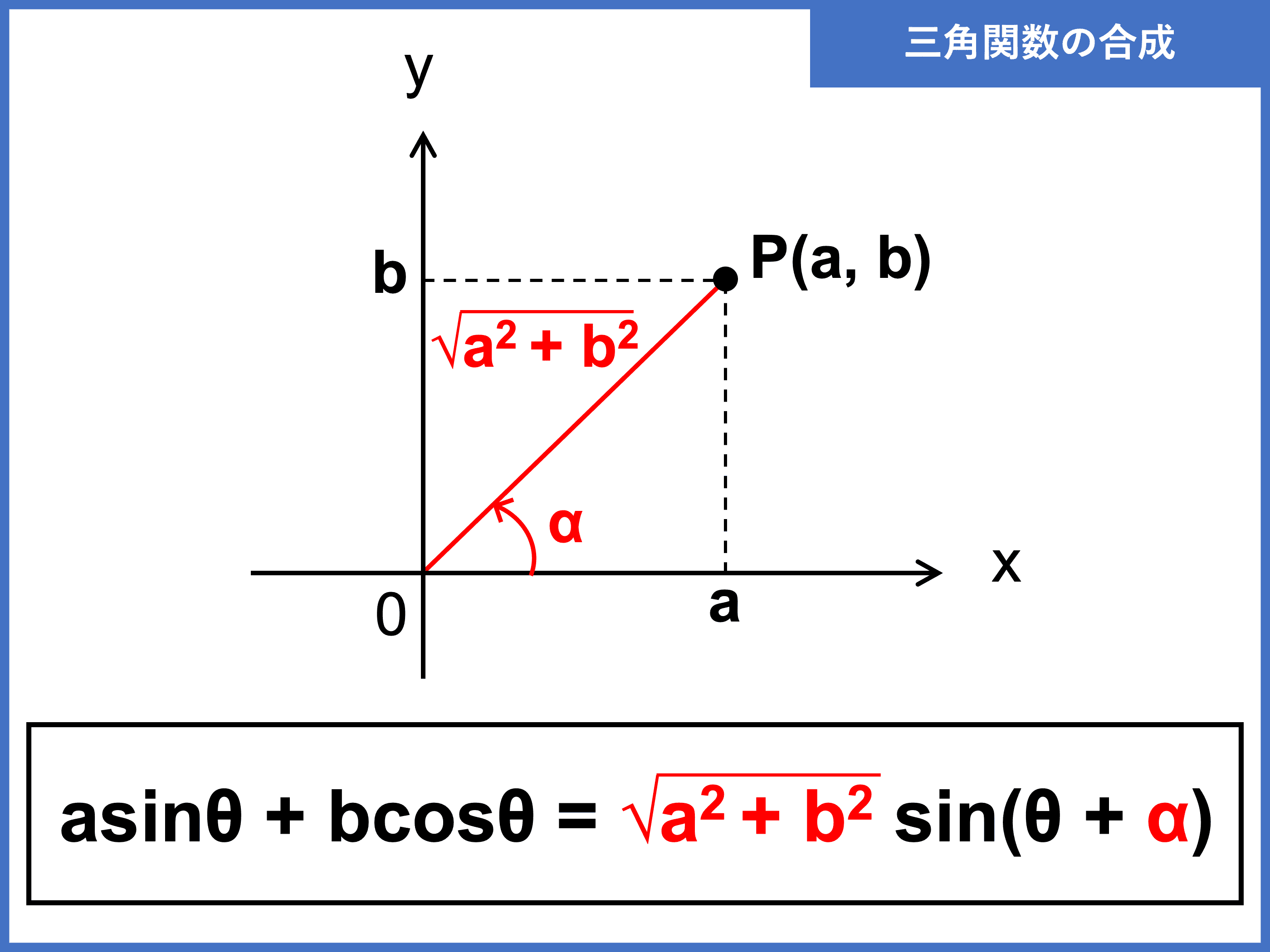
三角関数の合成
\(\sin\) と \(\cos\) の和は、\(\sin\) だけ、または \(\cos\) だけの式に合成することができます。
\(a \neq 0\), \(b \neq 0\) のとき、
\begin{align}a \sin\theta + b \cos\theta = \sqrt{a^2 + b^2} \sin(\theta + \alpha)\end{align}
ただし、
\begin{align}\displaystyle \cos\alpha = \frac{a}{\sqrt{a^2 + b^2}}, \displaystyle \sin\alpha = \frac{b}{\sqrt{a^2 + b^2}}\end{align}
 三角関数の合成とは?公式と証明、範囲つき最大最小の問題
三角関数の合成とは?公式と証明、範囲つき最大最小の問題
三角関数のグラフ
角度 \(\theta\) を横軸に、三角関数 \(\sin \theta\), \(\cos \theta\), \(\tan \theta\) それぞれを縦軸にとると、次のようなグラフが描けます。

三角関数のグラフの書き方については、以下の記事で詳しく説明しています。
 三角関数のグラフの書き方を徹底解説!平行移動や周期の問題も
三角関数のグラフの書き方を徹底解説!平行移動や周期の問題も
三角関数の微分公式
暗記しておくべき三角関数の微分公式です。
- \((\sin x)’ = \cos x\)
- \((\cos x)’ = −\sin x\)
- \(\displaystyle (\tan x)’ = \frac{1}{\cos^2 x}\)
- \(\displaystyle \left(\frac{1}{\tan x}\right)’ = −\frac{1}{\sin^2 x}\)
微分公式については、以下の記事で詳しく説明しています。
 微分とは?微分のやり方と全公式をわかりやすく解説!
微分とは?微分のやり方と全公式をわかりやすく解説!
三角関数の積分公式
暗記しておくべき三角関数の積分公式です。
積分定数を \(C\) とする。
- \(\displaystyle \int \sin x \ dx = −\cos x + C\)
- \(\displaystyle \int \cos x \ dx = \sin x + C\)
- \(\displaystyle \int \tan x \ dx = −\log |\cos x| + C\)
- \(\displaystyle \int \frac{1}{\cos^2 x} \ dx = \tan x + C\)
- \(\displaystyle \int \frac{1}{\sin^2 x} \ dx = −\frac{1}{\tan x} + C\)
積分公式については、以下の記事で詳しく説明しています。
 不定積分とは?公式ややり方をわかりやすく解説(分数の問題も)
不定積分とは?公式ややり方をわかりやすく解説(分数の問題も)
三角関数の極限公式
三角関数の極限において、暗記しておくべき公式です。
- \(\displaystyle \lim_{x \to 0} \frac{\sin x}{x} = 1\)
- \(\displaystyle \lim_{x \to 0} \frac{x}{\sin x} = 1\)
- \(\displaystyle \lim_{x \to 0} \frac{\tan x}{x} = 1\)
(ただし、角 \(x\) の単位は弧度法)
極限公式の使い方や三角関数の極限の求め方については、以下の記事で詳しく説明しています。
 極限とは?公式一覧や極限計算のポイントをわかりやすく解説!
極限とは?公式一覧や極限計算のポイントをわかりやすく解説!
以上が三角比・三角関数の公式一覧でした!
詳しい内容については、それぞれの関連記事を確認してみてくださいね。
