この記事では、「三角比」の定義や基本公式(三角比の相互関係)をできるだけわかりやすく解説していきます。
\(\sin\) \(\cos\) \(\tan\)(サイン コサイン タンジェント)とは何かについて、この記事を通して理解してくださいね!
目次
三角比とは?
三角比とは、長さの測量のために生み出された概念で、直角三角形の \(2\) 辺の比を角度を使って表したものです。
直角三角形の場合、\(1\) つの鋭角の大きさを決めるとすべての角の大きさが決まり、辺の比も決まります。このことを利用し、角度と辺の比を対応させたのが三角比です。
三角比には、注目する \(2\) 辺の位置に応じて「正弦 \(\sin\)」「余弦 \(\cos\)」「正接 \(\tan\)」の \(3\) 種類があります。
三角比の定義
\(\angle \mathrm{C} = 90^\circ\) の直角三角形 \(\mathrm{ABC}\) において、基準とする鋭角を \(\angle \mathrm{B} = \theta\) とおくと、三角比は次のように定義できます。
- 正弦 \(\sin \theta\) (サイン シータ)
\begin{align}\color{red}{\sin \theta = \displaystyle \frac{\text{たて}}{\text{斜辺}} = \displaystyle \frac{\mathrm{AC}}{\mathrm{AB}}}\end{align} - 余弦 \(\cos \theta\) (コサイン シータ)
\begin{align}\color{red}{\cos \theta = \displaystyle \frac{\text{よこ}}{\text{斜辺}} = \displaystyle \frac{\mathrm{BC}}{\mathrm{AB}}}\end{align} - 正接 \(\tan \theta\) (タンジェント シータ)
\begin{align}\color{red}{\tan \theta = \displaystyle \frac{\text{たて}}{\text{よこ}} = \displaystyle \frac{\mathrm{AC}}{\mathrm{BC}}}\end{align}

今回は頂点が \(\mathrm{A}\)、\(\mathrm{B}\)、\(\mathrm{C}\) の直角三角形ですが、頂点の記号は問題によって異なります。
ですので、記号ではなく辺の位置関係で覚えるようにしましょう!
三角比を考えるときには、直角三角形の直角を右下に、注目する鋭角 \(\theta\) を左下に配置するのが慣例です。
三角比の定義の覚え方
三角比の定義を覚える方法として、筆記体を利用した覚え方があります。
三角比のそれぞれの \(\bf{1}\) 文字目の筆記体を思い浮かべてください。
筆記体の書き順で直角三角形の辺をたどって「分母→分子」とみると、三角比の値になります。
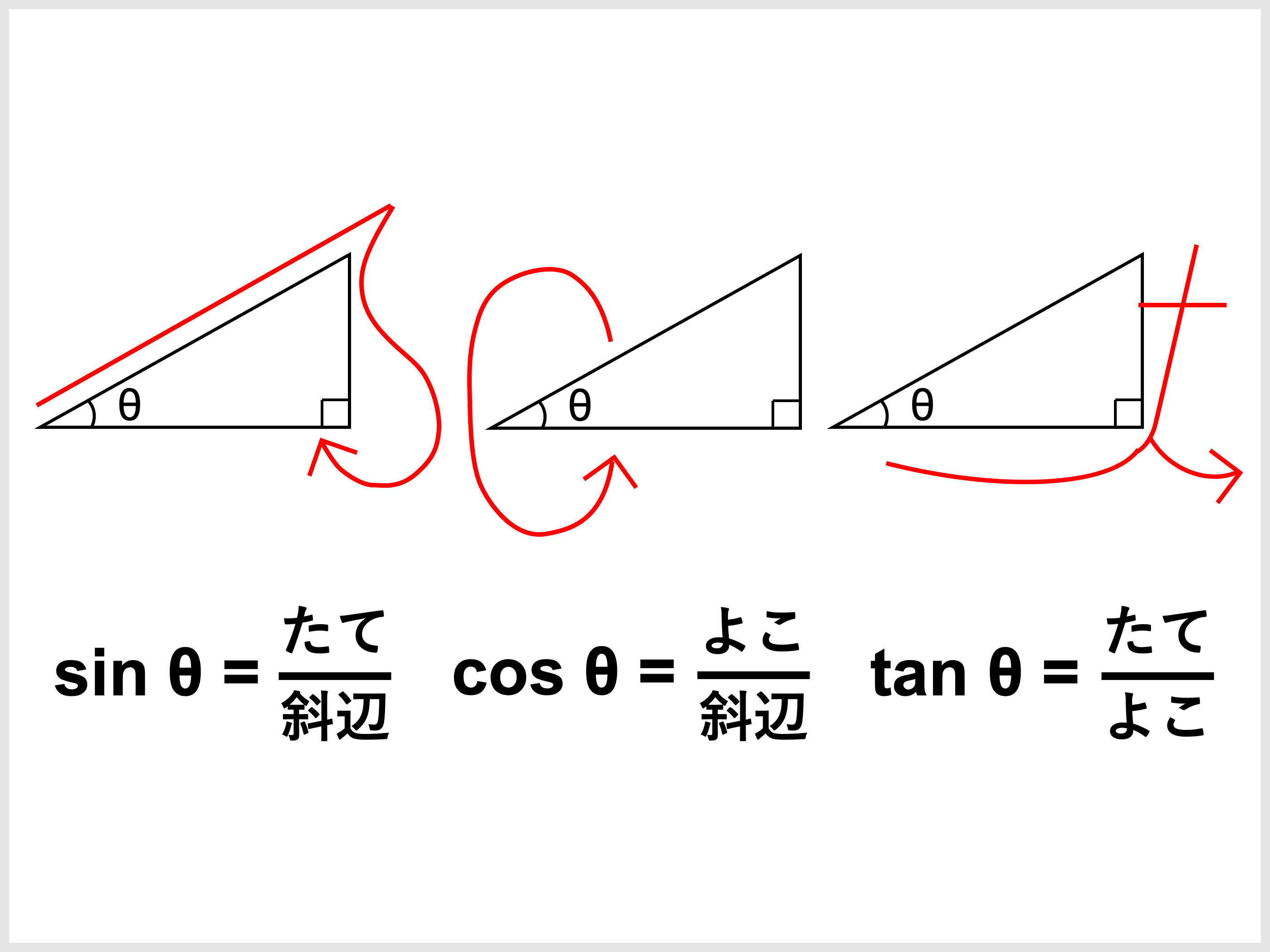
\(\sin\) は s、\(\cos\) は c、\(\tan\) は t です。
三角比に慣れるまでは、このような方法も知っておくと便利ですね!
三角比の拡張
さらに、もう少し三角比の考え方を拡張してみます。
半径 \(1\) の円を書き、その円周上に点 \(\mathrm{P}(x, y)\) をとります。
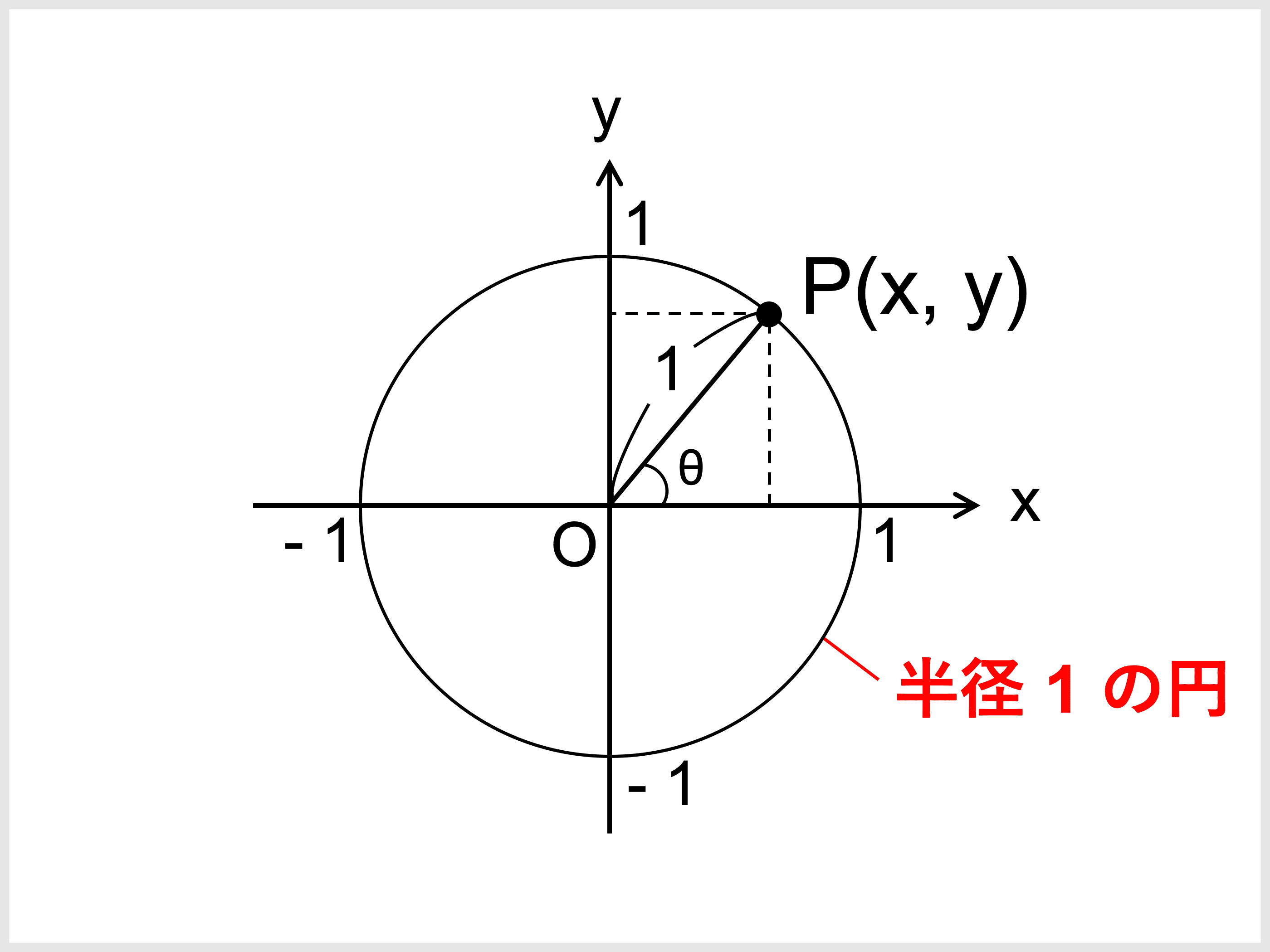
このような円を「単位円」といいます。
点 \(\mathrm{P}\) から \(x\) 軸上に垂線を下ろすと、直角三角形ができます。
すると、三角比の定義から、
\(\theta\) が鋭角のとき
- \(\displaystyle \sin \theta = \frac{y}{1} = y\)
- \(\displaystyle \cos \theta = \frac{x}{1} = x\)
- \(\displaystyle \tan \theta = \frac{y}{x}\)
という式が成り立ちます。
実は、点 \(\mathrm{P}\) の位置を動かしてみると、\(\theta\) が鋭角のときに限らず、\(0^\circ \leq \theta \leq 360^\circ\) すべての範囲で上記の関係式が成り立ちます。
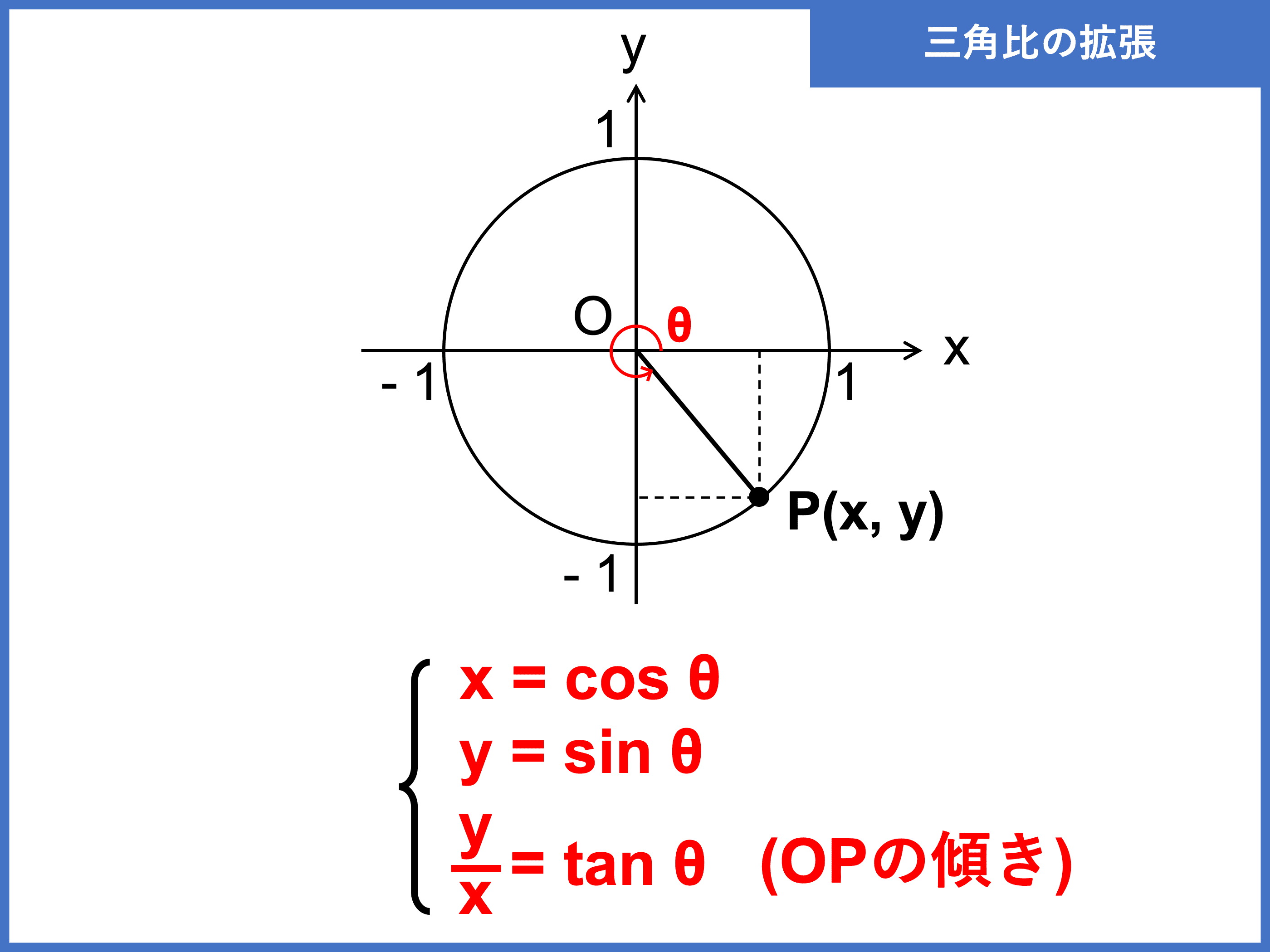
このように、もともとは直角三角形について定義された三角比ですが、角度 \(\theta\) の大きさによらず成り立つのですね。
この考え方は後で紹介する三角比の相互関係を求めるときにも利用するので、単位円の書き方とともに理解しておいてください。
ただし、\(\theta = 90^\circ\)、\(270^\circ\) のときは、\(x = 0\) となるため \(\tan \theta\) は定義されません。
\(\tan \theta\) だけは \(\theta \neq 90^\circ\), \(270^\circ\) に注意しましょう。
象限ごとの三角比の符号
また、象限ごとの三角比の符号は以下の通りです。

問題で角度の範囲が決まっているとき、三角比がとれる値の範囲も限られてくるので意識しておきましょう。
三角比の相互関係【公式】
\(\sin \theta\)、\(\cos \theta\)、\(\tan \theta\) の間には、必ず成り立つ特殊な関係性があり、「三角比の相互関係」と呼ばれています。
- \(\color{red}{\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}}\)
- \(\color{red}{\displaystyle \sin^2 \theta + \cos^2 \theta = 1}\)
- \(\color{red}{\displaystyle 1 + \tan^2 \theta = \frac{1}{\cos^2 \theta}}\)
三角比の相互関係から、\(\sin \theta\)、\(\cos \theta\)、\(\tan \theta\) のうちどれか \(1\) つでも値がわかれば、残りの \(2\) つの値を求められるのです!
三角比の問題では必須の公式なので、すべて正確に覚えましょう。
三角比の相互関係の覚え方
初めはなかなか覚えづらい三角比の相互関係ですが、式の導出方法を知ると意外とすんなり覚えられます!
三角比の相互関係は、単位円における三角比の定義式から簡単に導けます。
単位円とその円周上の点 \(\mathrm{P}(x, y)\) において、

- \(x = \cos \theta\) …①
- \(y = \sin \theta\) …②
- \(\displaystyle \frac{y}{x} = \tan \theta\) (\(\mathrm{OP}\) の傾き) …③
③に①、②を代入すると、
\(\displaystyle \frac{\sin \theta}{\cos \theta} = \tan \theta\)
すなわち
\(\color{red}{\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}}\)(\(1\) つ目の相互関係)
また、図の直角三角形において、三平方の定理より
\(x^2 + y^2 = 1\)
①、②を代入すると、
\(\color{red}{\sin^2 \theta + \cos^2 \theta = 1}\)(\(2\) つ目の相互関係)
さらに、\(\sin^2 \theta + \cos^2 \theta = 1\) の両辺を \(\cos^2 \theta\) で割ると、
\(\displaystyle \frac{\sin^2 \theta}{\cos^2 \theta} + \frac{\cos^2 \theta}{\cos^2 \theta} = \frac{1}{\cos^2 \theta}\)
\(\displaystyle \frac{\sin^2 \theta}{\cos^2 \theta} + 1 = \frac{1}{\cos^2 \theta}\)
\(\displaystyle \left( \frac{\sin \theta}{\cos \theta} \right)^2 + 1 = \frac{1}{\cos^2 \theta}\)
\(\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}\) より、
\(\color{red}{\displaystyle \tan^2 \theta + 1 = \frac{1}{\cos^2 \theta}}\)(\(3\) つ目の相互関係)
(証明終わり)
以上が、三角比の相互関係を導き出す一連の流れです。
単位円の図と共に理解しておけば、覚えやすく、かつ思い出しやすくなりますよ!
三角比の計算問題
それでは最後に、実際の計算を通して三角比の使い方をマスターしていきましょう。
計算問題①「45° の三角比の値を求める」
\(\sin 45^\circ\)、\(\cos 45^\circ\)、\(\tan 45^\circ\) の値を求めよ。

\(\theta = 45^\circ\) の直角三角形を実際に書いてみましょう。
辺の比が「\(1 : 1 : \sqrt{2}\)」になることを利用します。
\(\theta = 45^\circ\) の直角三角形の辺の比は \(1 : 1 : \sqrt{2}\) となる。
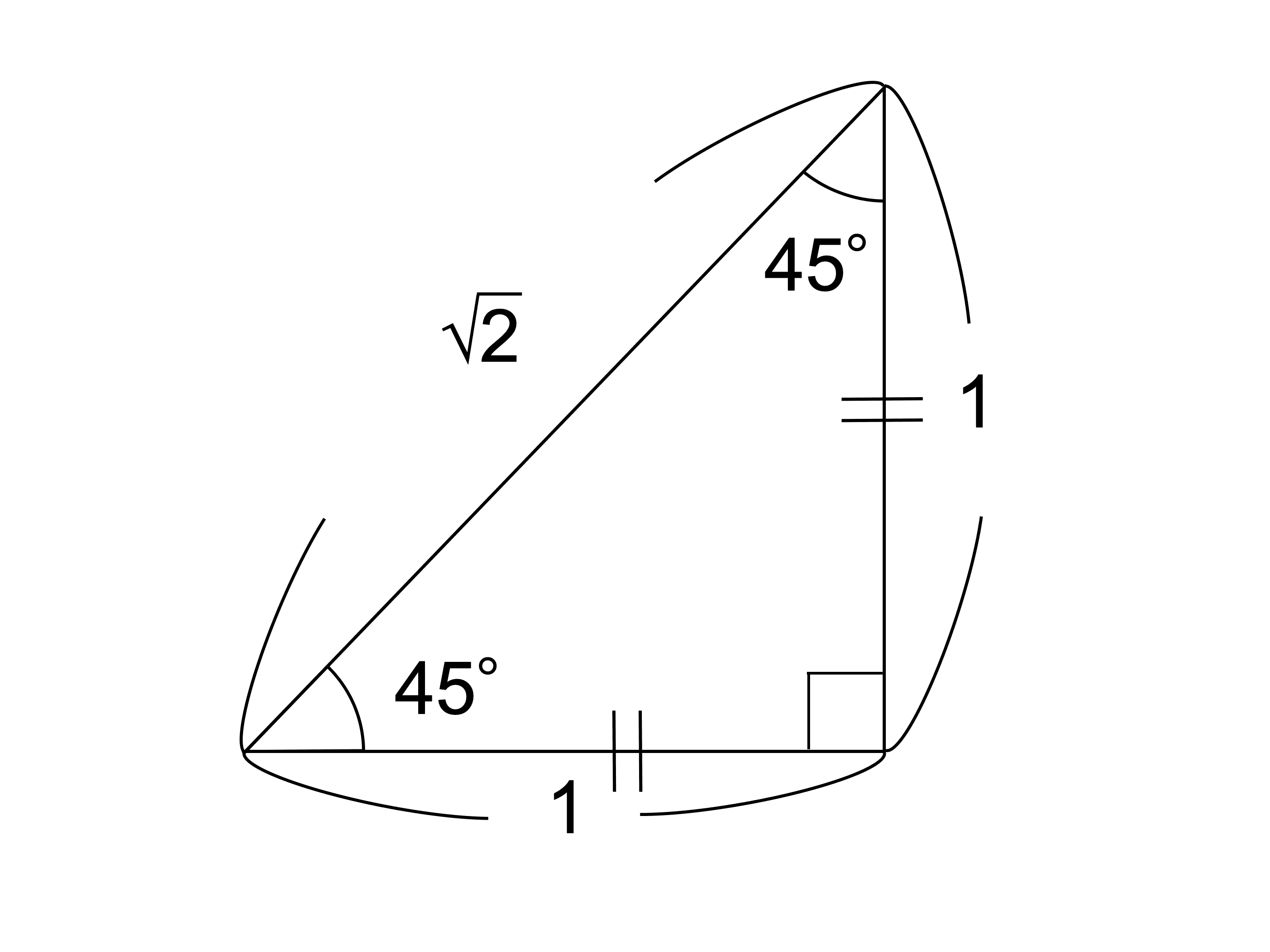
\(\sin \theta\) は \(\displaystyle \frac{\text{たて}}{\text{斜辺}}\)なので
\(\displaystyle \sin 45^\circ = \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)
\(\cos \theta\) は \(\displaystyle \frac{\text{よこ}}{\text{斜辺}}\) なので
\(\displaystyle \cos 45^\circ = \frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}\)
\(\tan \theta\) は \(\displaystyle \frac{\text{たて}}{\text{よこ}}\) なので
\(\displaystyle \tan 45^\circ = \frac{1}{1} = 1\)
答え:
\(\displaystyle \sin 45^\circ = \frac{\sqrt{2}}{2}\)、\(\displaystyle \cos 45^\circ = \frac{\sqrt{2}}{2}\)、\(\tan 45^\circ = 1\)
「代表的な直角三角形の角度と辺の比」については、以下の記事で紹介しています。
 直角三角形とは?定義や定理、辺の長さの比、合同条件
直角三角形とは?定義や定理、辺の長さの比、合同条件
計算問題②「直角三角形の三角比の値を求める」
以下の直角三角形について、\(\sin \theta\)、\(\cos \theta\)、\(\tan \theta\) をそれぞれ求めよ。


角度 \(\theta\) がわからなくても、\(3\) 辺の長さから三角比を求められますね。
図をしっかりと見て、三角比の値を考えましょう。
\(\sin \theta\) は \(\displaystyle \frac{\text{たて}}{\text{斜辺}}\) なので
\(\displaystyle \sin \theta = \frac{\mathrm{CA}}{\mathrm{BC}} = \frac{\sqrt{3}}{3}\)
\(\cos \theta\) は \(\displaystyle \frac{\text{よこ}}{\text{斜辺}}\) なので
\(\displaystyle \cos \theta = \frac{\mathrm{AB}}{\mathrm{BC}} = \frac{2\sqrt{3}}{3}\)
\(\tan \theta\) は \(\displaystyle \frac{\text{たて}}{\text{よこ}}\) なので
\(\displaystyle \tan \theta = \frac{\mathrm{CA}}{\mathrm{AB}} = \frac{\sqrt{3}}{2\sqrt{3}} = \frac{1}{2}\)
答え:
\(\displaystyle \sin \theta = \frac{\sqrt{3}}{3}\)、\(\displaystyle \cos \theta = \frac{2\sqrt{3}}{3}\)、\(\displaystyle \tan \theta = \frac{1}{2}\)
計算問題③「sin θ の値から cos θ, tan θ を求める」
\(\theta\) は鋭角とする。\(\displaystyle \sin \theta =\frac{ 2\sqrt{2}}{3}\) のとき、\(\cos \theta\) と \(\tan \theta\) の値を求めよ。

\(\sin \theta\) の値がわかっているので、三角形の相互関係を使って \(\cos \theta\)、\(\tan \theta\) が順に求められます。
三角比の相互関係を使う際は、角度 \(\theta\) の範囲を常に意識しましょう。
三角比の相互関係より、
\(\sin^2 \theta + \cos^2 \theta = 1\)
\(\displaystyle \sin \theta = \frac{2\sqrt{2}}{3}\) を代入すると、
\(\displaystyle \left( \frac{2\sqrt{2}}{3} \right)^2 + \cos^2 \theta = 1\)
\(\displaystyle \frac{8}{9} + \cos^2 \theta = 1\)
\(\begin{align} \cos^2 \theta &= 1 − \frac{8}{9} \\ &= \frac{1}{9} \end{align}\)
\(\theta\) は鋭角なので、\(\cos \theta > 0\) であるから
\(\displaystyle \cos \theta = \frac{1}{3}\)
三角形の相互関係より、
\(\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}\)
\(\displaystyle \sin \theta = \frac{2\sqrt{2}}{3}\)、\(\displaystyle \cos \theta = \frac{1}{3}\) を代入すると、
\(\begin{align} \tan \theta &= \frac{2\sqrt{2}}{3} \div \frac{1}{3} \\ &= \frac{2\sqrt{2}}{3} \times 3 \\ &= 2\sqrt{2} \end{align}\)
答え: \(\displaystyle \cos \theta = \frac{1}{3}\)、\(\tan \theta = 2\sqrt{2}\)
以上で計算問題も終わりです!
三角比について、理解が深まりましたか?
まずは直角三角形の図や単位円の図とともに、三角比の導き方をしっかりと理解しておきましょう。
「主要な三角比の値」については、以下の記事で説明しています。
 三角関数表(1°ごとの三角比の表)と有名角の三角比の求め方!
三角関数表(1°ごとの三角比の表)と有名角の三角比の求め方!
