この記事では、「二点間の距離」について、一次元、二次元、三次元それぞれの公式をわかりやすく解説していきます。
平面・空間座標の問題も取り上げていますので、ぜひこの記事を通してマスターしてくださいね!
目次
二点間の距離の公式
二点間の距離とは、\(2\) 点 \(\mathrm{A, B}\) があるとき、その距離(線分 \(\mathrm{AB}\) の長さ)のことです。
一次元(数直線上)、二次元(座標平面上)、三次元(座標空間上)のそれぞれにおいて、二点間の距離を表す公式があります。
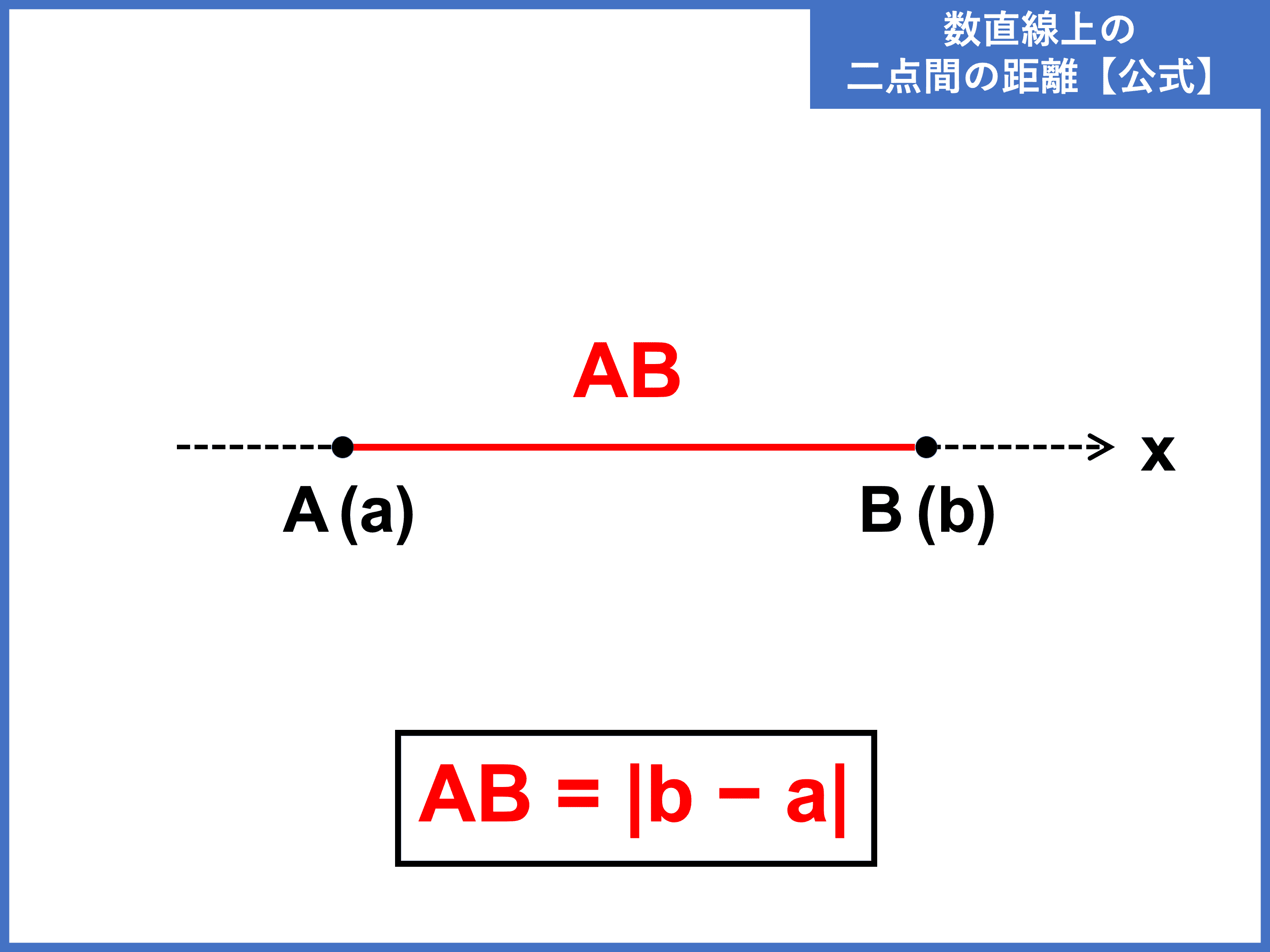
① 数直線上の \(2\) 点 \(\mathrm{A}(a)\), \(\mathrm{B}(b)\) の距離は
\begin{align}\color{red}{\mathrm{AB} = |b − a|}\end{align}
② 座標平面上の \(2\) 点 \(\mathrm{A}(x_1, y_1)\), \(\mathrm{B}(x_2, y_2)\) の距離は
\begin{align}\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2}}\end{align}
③ 座標空間上の \(2\) 点 \(\mathrm{A}(x_1, y_1, z_1)\), \(\mathrm{B}(x_2, y_2, z_2)\) の距離は
\begin{align}\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2 + (z_2 − z_1)^2}}\end{align}
(見切れる場合は横へスクロール)
それぞれ詳しく解説していきます。
公式① 数直線上の二点間の距離
まず最初に、数直線上における二点間の距離の公式です。
数直線上に \(2\) 点 \(\mathrm{A}(a)\), \(\mathrm{B}(b)\) があるとき、二点間の距離 \(\mathrm{AB}\) は
\begin{align}\color{red}{\mathrm{AB} = |b − a|}\end{align}
特に、原点 \(\mathrm{O}(0)\) と点 \(\mathrm{A}(a)\) の二点間の距離は
\begin{align}\color{red}{\mathrm{OA} = |a|}\end{align}
点 \(\mathrm{A}(8)\) と点 \(\mathrm{B}(5)\) の距離 \(\mathrm{AB}\) を求めなさい。
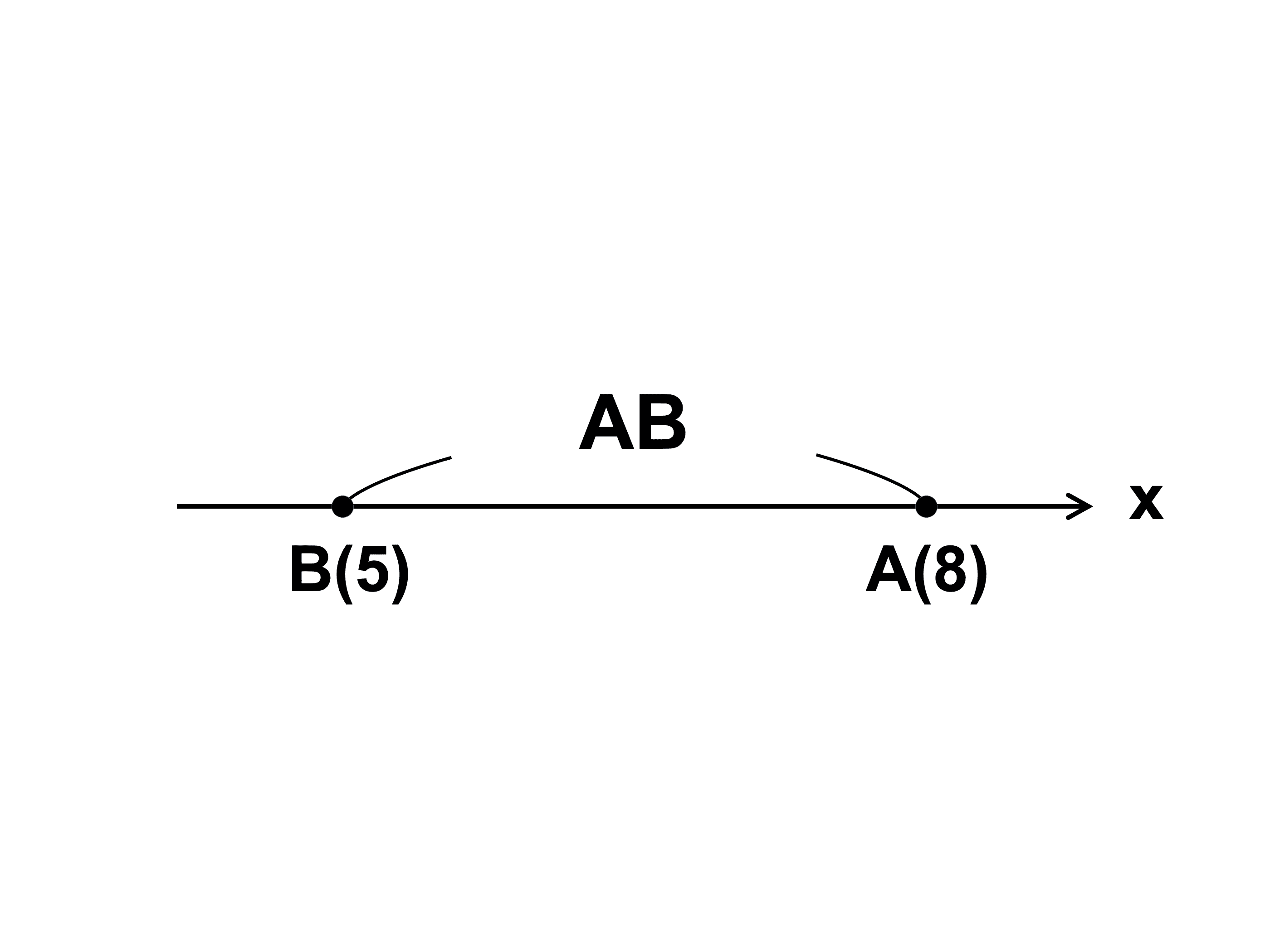
公式に当てはめます。
ちなみに、絶対値があるのでどちらからどちらを引いても構いません。
\(\begin{align} \mathrm{AB} &= |b − a| \\ &= |5 − 8| \\ &= |−3| \\ &= 3 \end{align}\)
答え: \(\color{red}{\mathrm{AB} = 3}\)
公式①の証明
数直線上における距離は、絶対値をとることで表せます。
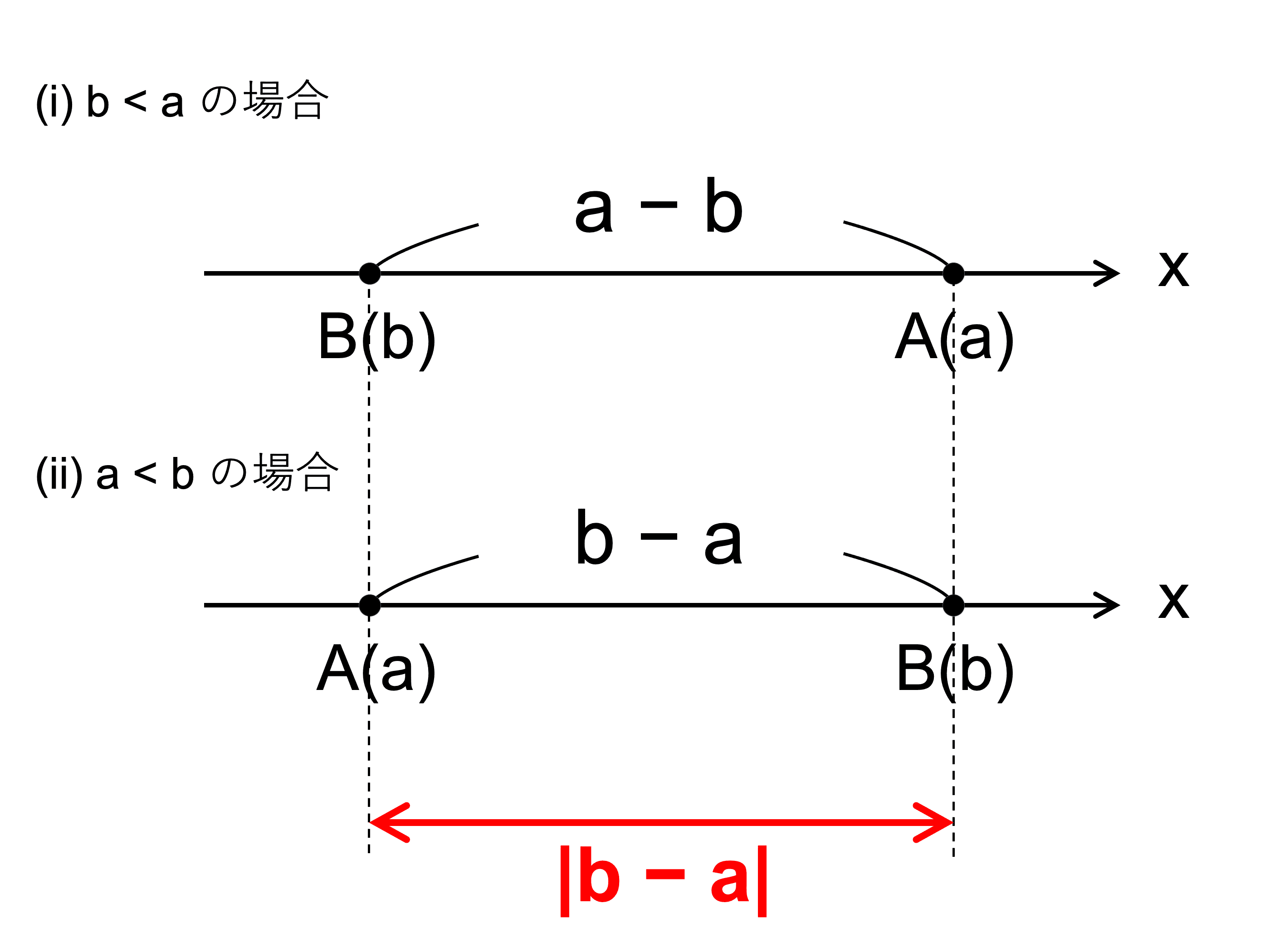
(i) \(b < a\) の場合
\(\mathrm{AB} = a − b = −(b − a) = |b − a|\)
(ii) \(a < b\) の場合
\(\mathrm{AB} = b − a = |b − a|\)
したがって、\(2\) 点 \(\mathrm{A}(a)\), \(\mathrm{B}(b)\) の距離 \(\mathrm{AB}\) は
\(\color{red}{\mathrm{AB} = |b − a|}\)
このように、数直線上の二点間の距離は、座標の差の絶対値となります。
公式② 平面上の二点間の距離
次に、座標平面上における二点間の距離の公式です。
座標平面上に \(2\) 点 \(\mathrm{A}(x_1, y_1)\), \(\mathrm{B}(x_2, y_2)\) があるとき、二点間の距離 \(\mathrm{AB}\) は
\begin{align}\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2}}\end{align}

特に、原点 \(\mathrm{O}(0, 0)\) と点 \(\mathrm{A}(x_1, y_1)\) の二点間の距離は
\begin{align}\color{red}{\mathrm{OA} = \sqrt{x_1^2 + y_1^2}}\end{align}
点 \(\mathrm{A}(4, 3)\) と点 \(\mathrm{B}(7, 5)\) の距離 \(\mathrm{AB}\) を求めなさい。
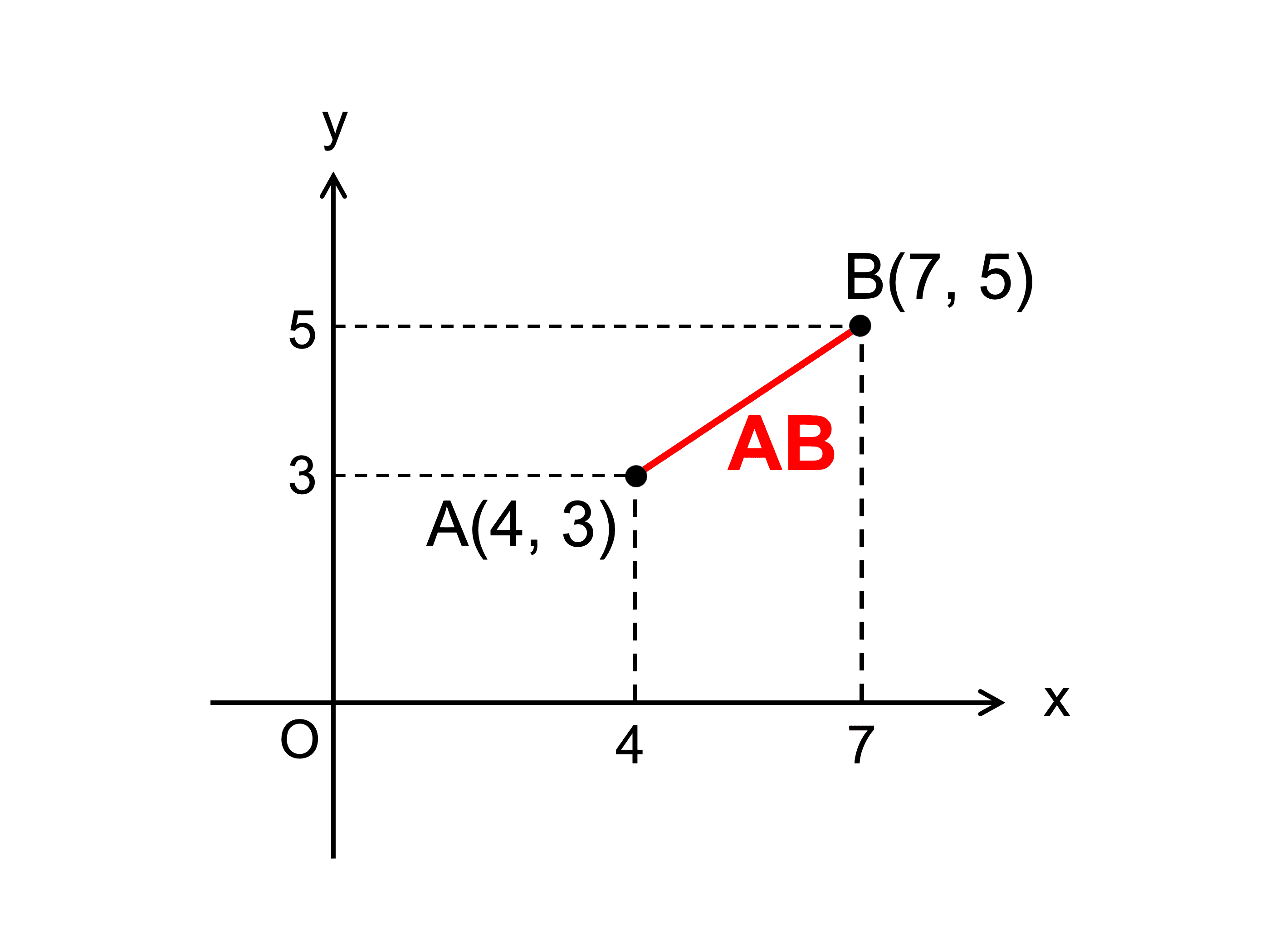
点 \(\mathrm{A}\) の座標から点 \(\mathrm{B}\) の座標を引いても、あるいは反対でも構いません。ただし、\(x\), \(y\) 座標でどちらからどちらを引くかは必ず統一させましょう。
\(\begin{align} \mathrm{AB} &= \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2} \\ &= \sqrt{(7 − 4)^2 + (5 − 3)^2} \\ &= \sqrt{3^2 + 2^2} \\ &= \sqrt{9 + 4} \\ &= \sqrt{13} \end{align}\)
答え: \(\color{red}{\mathrm{AB} = \sqrt{13}}\)
公式②の証明
この公式は、三平方の定理を使って証明できます。
三平方の定理
直角三角形の直角を挟む \(2\) 辺の長さを \(a\), \(b\) とし、斜辺を \(c\) とすると、
\begin{align}a^2 + b^2 = c^2\end{align}
線分 \(\mathrm{AB}\) が斜辺となるように直角三角形 \(\mathrm{ABC}\) を作るのがポイントです。
点 \(\mathrm{C}(x_2, y_1)\) をとる。
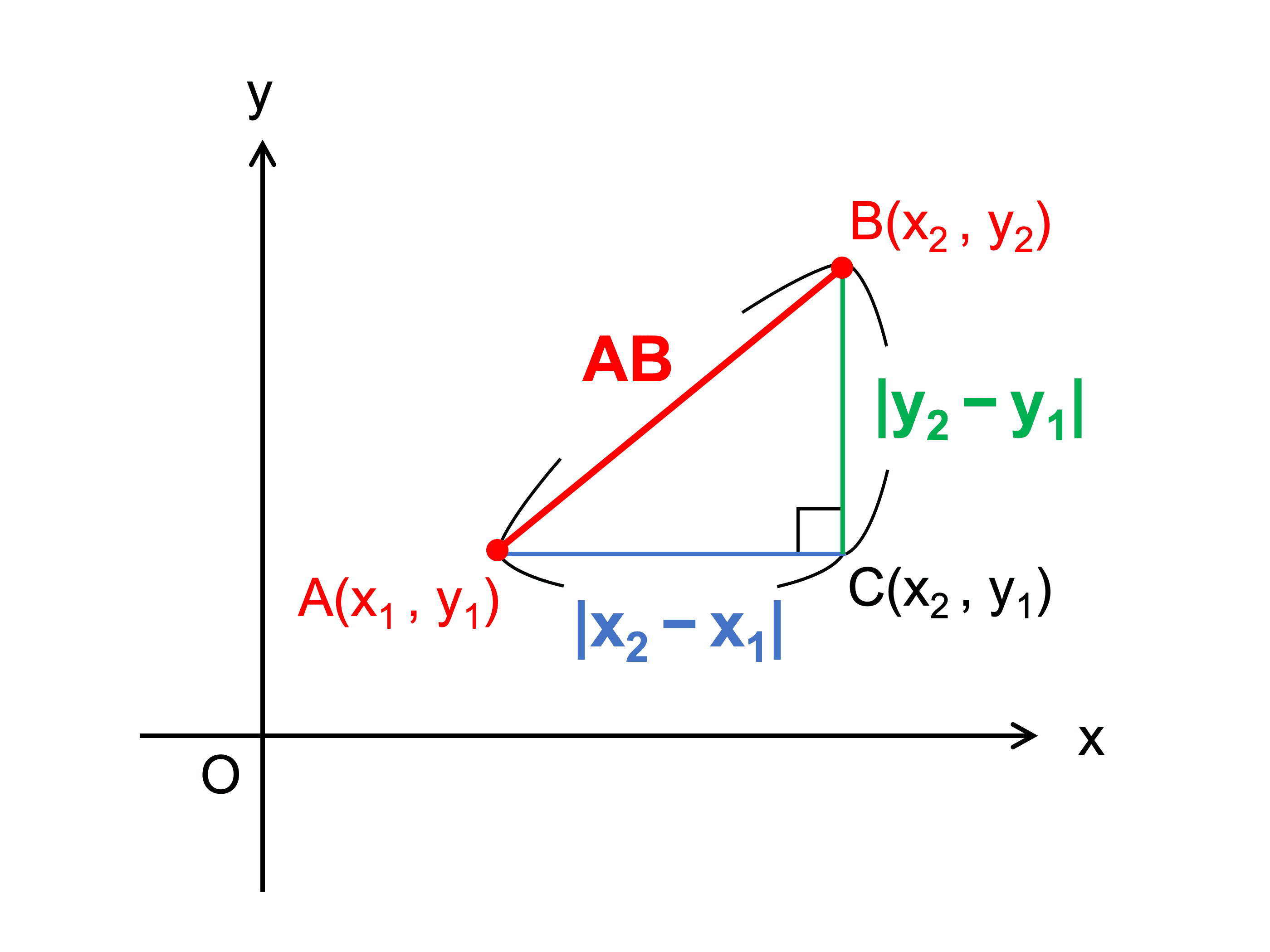
\(\triangle{\mathrm{ABC}}\) において、三平方の定理より
\(\begin{align} \mathrm{AB}^2 &= \mathrm{AC}^2 + \mathrm{BC}^2 \\ &= (x_2 − x_1)^2 + (y_2 − y_1)^2 \end{align}\)
\(\mathrm{AB} > 0\) より、
\(\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2}}\)
(証明終わり)
このように、図とあわせて理解しておくとより定着しますよ!
公式③ 空間上の二点間の距離
最後は、空間上における二点間の距離の公式です。
座標空間上に \(2\) 点 \(\mathrm{A}(x_1, y_1, z_1)\), \(\mathrm{B}(x_2, y_2, z_2)\) があるとき、二点間の距離 \(\mathrm{AB}\) は
\begin{align}\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2 + (z_2 − z_1)^2}}\end{align}
(見切れる場合は横へスクロール)
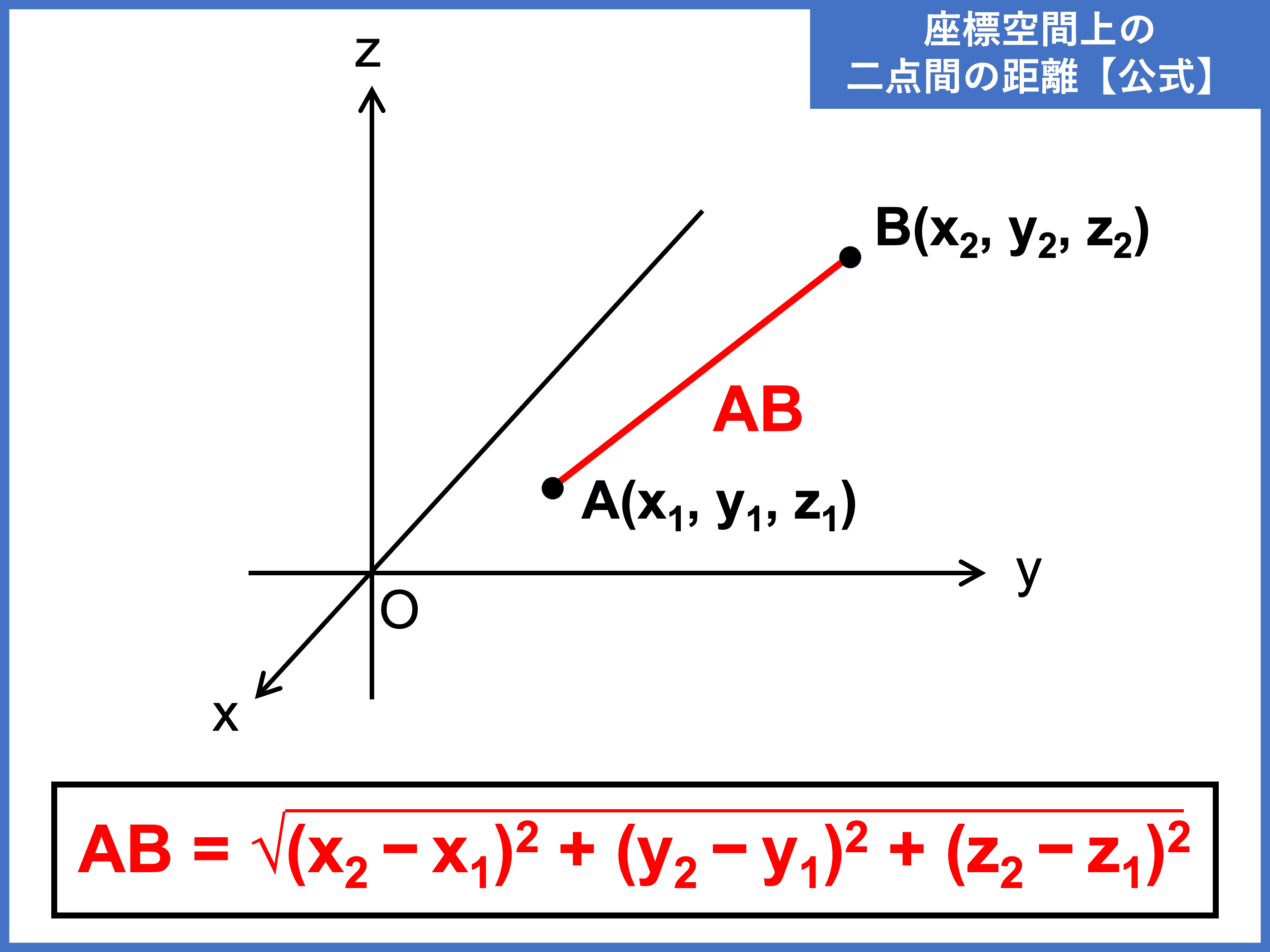
特に、原点 \(\mathrm{O}(0, 0, 0)\) と点 \(\mathrm{A}(x_1, y_1, z_1)\) の二点間の距離は
\begin{align}\color{red}{\mathrm{OA} = \sqrt{x_1^2 + y_1^2 + z_1^2}}\end{align}
点 \(\mathrm{A}(4, 3, 2)\) と点 \(\mathrm{B}(1, 1, 4)\) の距離 \(\mathrm{AB}\) を求めなさい。
公式に当てはめます。\(x, y, z\) 座標で引く順番が入れ替わらないように注意してくださいね。
\(\mathrm{AB}\)
\(= \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2 + (z_2 − z_1)^2}\)
\(= \sqrt{(1 − 4)^2 + (1 − 3)^2 + (4 − 2)^2}\)
\(= \sqrt{(− 3)^2 + (− 2)^2 + 2^2}\)
\(= \sqrt{9 + 4 + 4}\)
\(= \sqrt{17}\)
答え: \(\color{red}{\mathrm{AB} = \sqrt{17}}\)
公式③の証明
この公式も、三平方の定理で証明できます。
点 \(\mathrm{A}\), \(\mathrm{B}\) を対角にもつ直方体をイメージするとわかりやすいです。
(見切れる場合は横へスクロール)
点 \(\mathrm{C}(x_2, y_2, z_1)\) とおく。
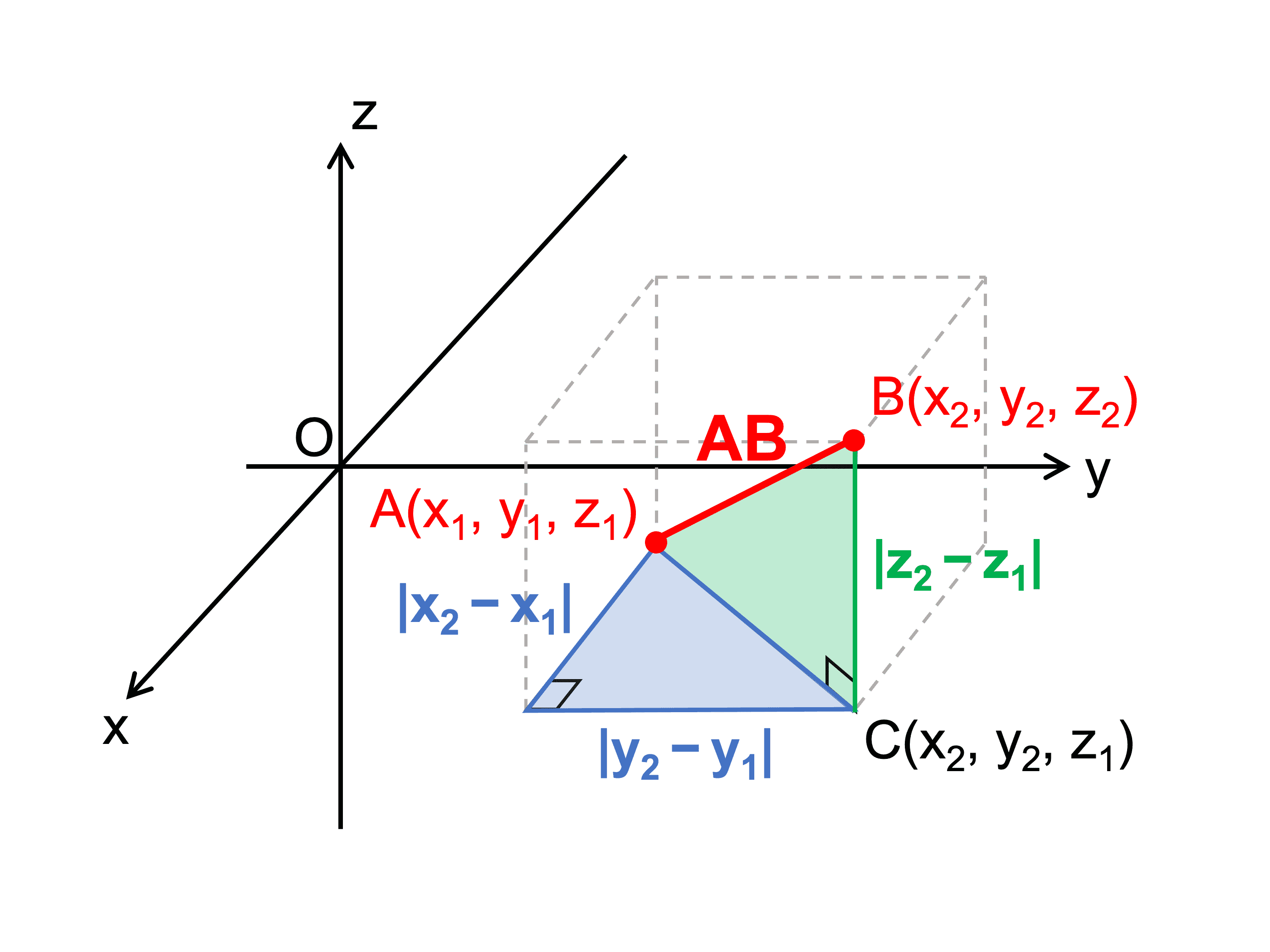
\(\triangle{\mathrm{ABC}}\) において、三平方の定理より
\(\mathrm{AB}^2 = \mathrm{AC}^2 + \mathrm{BC}^2\)
ここで、
\(\mathrm{AC}^2 = (x_2 − x_1)^2 + (y_2 − y_1)^2\)
\(\mathrm{BC}^2 = (z_2 − z_1)^2\)
であるから
\(\begin{align}\mathrm{AB}^2 &= \mathrm{AC}^2 + \mathrm{BC}^2 \\&= \{(x_2 − x_1)^2 + (y_2 − y_1)^2\} + (z_2 − z_1)^2 \\&= (x_2 − x_1)^2 + (y_2 − y_1)^2 + (z_2 − z_1)^2\end{align}\)
\(\mathrm{AB} > 0\) より、
\(\color{red}{\mathrm{AB} = \sqrt{(x_2 − x_1)^2 + (y_2 − y_1)^2 + (z_2 − z_1)^2}}\)
(証明終わり)
二点間の距離の計算問題
それでは、二点間の距離の計算問題に挑戦してみましょう!
計算問題①「数直線上の 2 点から等距離にある点」
数直線上において、\(2\) 点 \(\mathrm{A}(7)\), \(\mathrm{B}(−21)\) から等距離にある座標を求めなさい。

求めたい点を \(\mathrm{P}\) などと文字でおいて考えましょう。
求める点は \(\mathrm{AB}\) の間に存在することに注意です。
求める点を \(\mathrm{P}(p)\) とおくと、
\(\mathrm{AP} = \mathrm{PB}\)
\(− 21 < p < 7\) より
\(7 − p = p − (− 21)\)
\(2p = − 14\)
よって \(p = − 7\)
答え: \(\mathrm{P}(− 7)\)
計算問題②「平面上の三角形の面積」
頂点 \(\mathrm{A}(2, 2)\)、\(\mathrm{B}(6, 8)\)、\(\mathrm{C}(8, 0)\) からなる \(\triangle \mathrm{ABC}\) があり、頂点 \(\mathrm{B}\) から辺 \(\mathrm{AC}\) に下ろした垂線の足を \(\mathrm{H}\) とおく。
このとき、\(\mathrm{AC}\) と \(\mathrm{BH}\) の長さを使って \(\triangle \mathrm{ABC}\) の面積を求めよ。
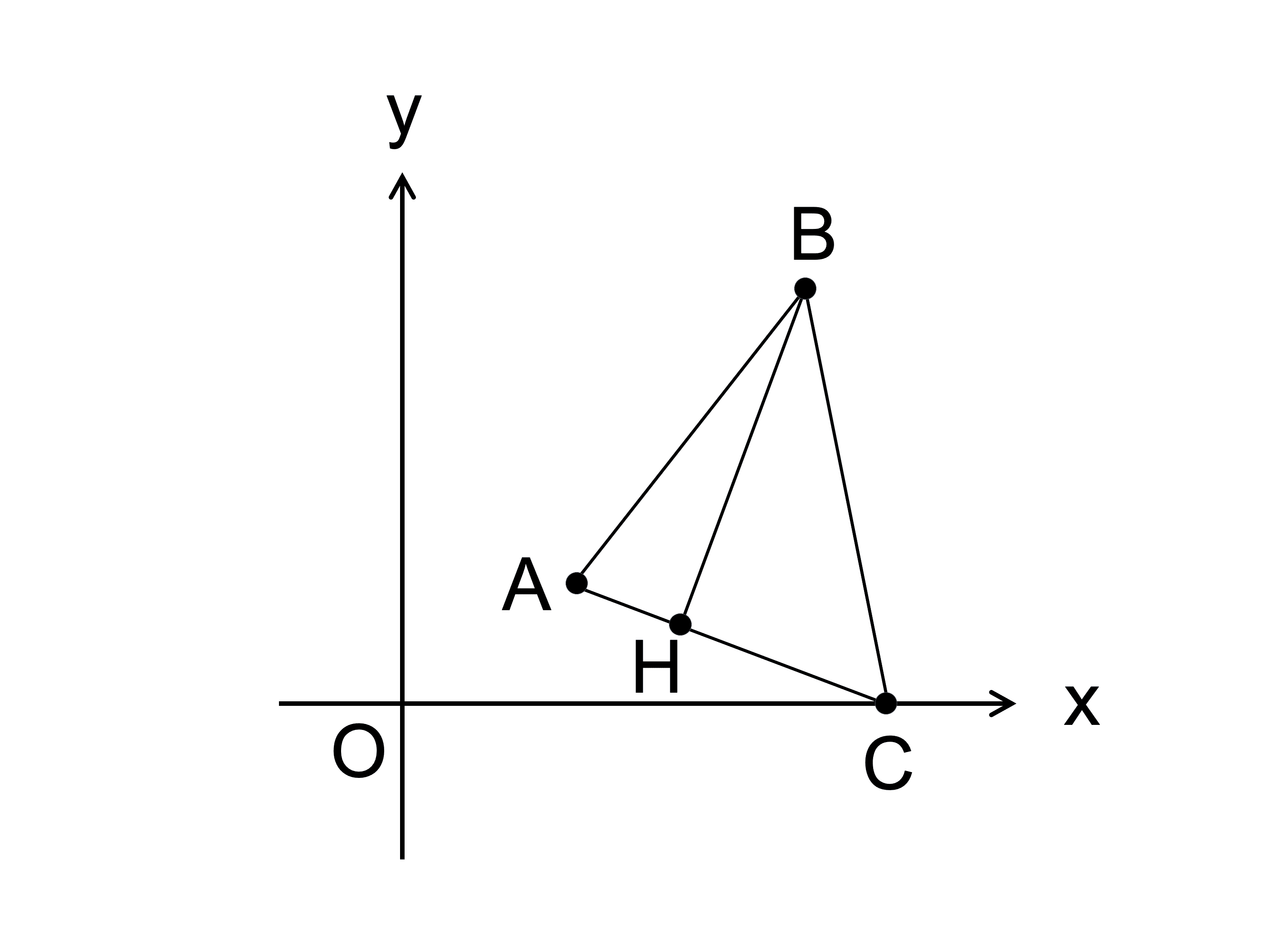

\(2\) つの直角三角形に注目して、\(\triangle \mathrm{ABC}\) の底辺 \(\mathrm{AC}\) と高さ \(\mathrm{BH}\) の長さを求めましょう。
複数の二点間の距離を計算する場合は、途中計算で根号を取らずに \(2\) 乗のまま計算すると楽ですよ!
\(\mathrm{A}(2, 2)\)、\(\mathrm{B}(6, 8)\) より、
\(\begin{align} \mathrm{AB}^2 &= (6 − 2)^2 + (8 − 2)^2 \\ &= 4^2 + 6^2 \\ &= 16 + 36 \\ &= 52 \end{align}\)
\(\mathrm{B}(6, 8)\)、\(\mathrm{C}(8, 0)\) より、
\(\begin{align} \mathrm{BC}^2 &= (8 − 6)^2 + (0 − 8)^2 \\ &= 2^2 + (− 8)^2 \\ &= 4 + 64 \\ &= 68 \end{align}\)
\(\mathrm{A}(2, 2)\)、\(\mathrm{C}(8, 0)\) より、
\(\begin{align} \mathrm{AC}^2 &= (8 − 2)^2 + (0 − 2)^2 \\ &= 6^2 + (− 2)^2 \\ &= 36 + 4 \\ &= 40 \end{align}\)
\(\mathrm{AC} > 0\) より \(\mathrm{AC} = 2\sqrt{10}\)
ここで、
\(\begin{align}\mathrm{HC} &= \mathrm{AC} − \mathrm{AH} \\ &= 2\sqrt{10} − \mathrm{AH}\end{align}\)
\(\triangle \mathrm{ABH}\) において、三平方の定理より
\(\mathrm{AB}^2 = \mathrm{AH}^2 + \mathrm{BH}^2\)
\(52 = \mathrm{AH}^2 + \mathrm{BH}^2\) …①
\(\triangle \mathrm{BCH}\) において、三平方の定理より
\(\mathrm{BC}^2 = \mathrm{BH}^2 + \mathrm{HC}^2\)
\(68 = \mathrm{BH}^2 + (2\sqrt{10} − \mathrm{AH})^2\)
\(68 = \mathrm{BH}^2 + 40 − 4\sqrt{10} \mathrm{AH} + \mathrm{AH}^2\)
\(28 = \mathrm{BH}^2 − 4\sqrt{10} \mathrm{AH} + \mathrm{AH}^2\) …②
① − ② より
\(24 = 4\sqrt{10} \mathrm{AH}\)
\(\begin{align} \mathrm{AH} &= \frac{24}{4\sqrt{10}} \\ &= \frac{6}{\sqrt{10}} \\ &= \frac{6\sqrt{10}}{10} \\ &= \frac{3\sqrt{10}}{5} \end{align}\)
① より
\(\begin{align} \mathrm{BH}^2 &= 52 − \mathrm{AH}^2 \\ &= 52 − \frac{90}{25} \\ &= 52 − \frac{18}{5} \\ &= \frac{260 − 18}{5} \\ &= \frac{242}{5} \end{align}\)
\(\mathrm{BH} > 0\) より
\(\begin{align} \mathrm{BH} &= \sqrt{\frac{242}{5}} \\ &= \sqrt{\frac{484}{10}} \\ &= \frac{22}{\sqrt{10}} \\ &= \frac{22\sqrt{10}}{10} \\ &= \frac{11\sqrt{10}}{5} \end{align}\)
したがって、
\(\begin{align} \triangle \mathrm{ABC} &= \frac{1}{2} \mathrm{AC} \cdot \mathrm{BH} \\ &= \frac{1}{2} \cdot 2\sqrt{10} \cdot \frac{11\sqrt{10}}{5} \\ &= \frac{11 \cdot 10}{5} \\ & = 22 \end{align}\)
答え: \(22\)
計算問題③「空間上の 3 点から等距離にある点」
\(xy\) 平面上にあって、\(3\) 点 \(\mathrm{A}(2, −3, 1)\)、\(\mathrm{B}(0, 4, 2)\)、\(\mathrm{C}(2, 4, 0)\) から等距離にある座標を求めなさい。

\(xy\) 平面上の点は \(z\) 座標が \(0\) です。
求めたい点を \(\mathrm{P}(x, y, 0)\) とおいて、二点間の距離の公式を利用しましょう。
(見切れる場合は横へスクロール)
求める点を点 \(\mathrm{P}(x, y, 0)\) とおく。
二点間の距離の公式から、
\(\begin{align} \mathrm{PA}^2 &= (x − 2)^2 + \{y − (−3)\}^2 + (0 − 1)^2 \\ &= x^2 − 4x + 4 + y^2 + 6y + 9 + 1 \\ &= x^2 − 4x + y^2 + 6y + 14 \text{ …①}\end{align}\)
\(\begin{align} \mathrm{PB}^2 &= (x − 0)^2 + (y − 4)^2 + (0 − 2)^2 \\ &= x^2 + y^2 − 8y + 16 + 4 \\ &= x^2 + y^2 − 8y + 20 \text{ …②}\end{align}\)
\(\begin{align} \mathrm{PC}^2 &= (x − 2)^2 + (y − 4)^2 + (0 − 0)^2 \\ &= x^2 − 4x + 4 + y^2 − 8y + 16 \\ &= x^2 − 4x + y^2 − 8y + 20 \text{ …③} \end{align}\)
\(\mathrm{PA} = \mathrm{PB} = \mathrm{PC}\) より
\(\mathrm{PA}^2 = \mathrm{PB}^2 = \mathrm{PC}^2\) であるから、
① = ②より
\(x^2 − 4x + y^2 + 6y + 14 = x^2 + y^2 − 8y + 20\)
\(−2x + 7y − 3 = 0\) …④
② = ③より
\(x^2 + y^2 − 8y + 20 = x^2 − 4x + y^2 − 8y + 20\)
\(4x = 0\)
\(x = 0\) …⑤
④、⑤より、
\(7y = 3\)
\(\displaystyle y = \frac{3}{7}\)
したがって、 \(\displaystyle \mathrm{P} \left( 0, \frac{3}{7}, 0 \right)\)
答え: \(\displaystyle \left( 0, \frac{3}{7}, 0 \right)\)
以上で問題も終わりです!
二点間の距離について、理解が深まりましたか?
公式としては \(3\) つ出てきましたが、基本的な考え方は同じです。
図とともに公式の導き方を理解しておくと、頭に定着しますよ!

