この記事では、「極方程式」についてわかりやすく解説していきます。
グラフの書き方や \((x, y)\) の方程式との変換方法、面積や曲線の長さの公式なども説明していきますので、ぜひこの記事を通してマスターしてくださいね。
目次
極方程式とは？
極方程式とは、極座標 \((r, \theta)\) に関する方程式です。
平面上のある曲線が極座標 \((r, \theta)\) に関する方程式
\(\color{red}{r = f(\theta)}\) または \(\color{red}{F(r, \theta) = 0}\)
で表されるとき、これを「曲線の極方程式」という。
極方程式では、\(\theta\) も \(r\) も負の値をとることができ、次の関係が成り立ちます。
\((r, \theta) = (−r, \theta + \pi)\)
さまざまな極方程式【公式】
ここでは、代表的な図形の極方程式を示します。
極方程式は、公式を丸暗記するだけでは理解が深まりません。
実際に自分で図を書いてみて、極方程式の意味を理解できるように心がけましょう。
直線の極方程式
直線には、次の \(2\) 通りの極方程式があります。
- 極 \(\mathrm{O}\) を通り、始線となす角が \(\alpha\) の直線
\begin{align}\color{red}{\theta = \alpha}\end{align} - 点 \(\mathrm{A}(a, \alpha)\) を通り、\(\mathrm{OA}\) に垂直な直線
\begin{align}\color{red}{r\cos(\theta − \alpha) = a (a > 0)}\end{align}
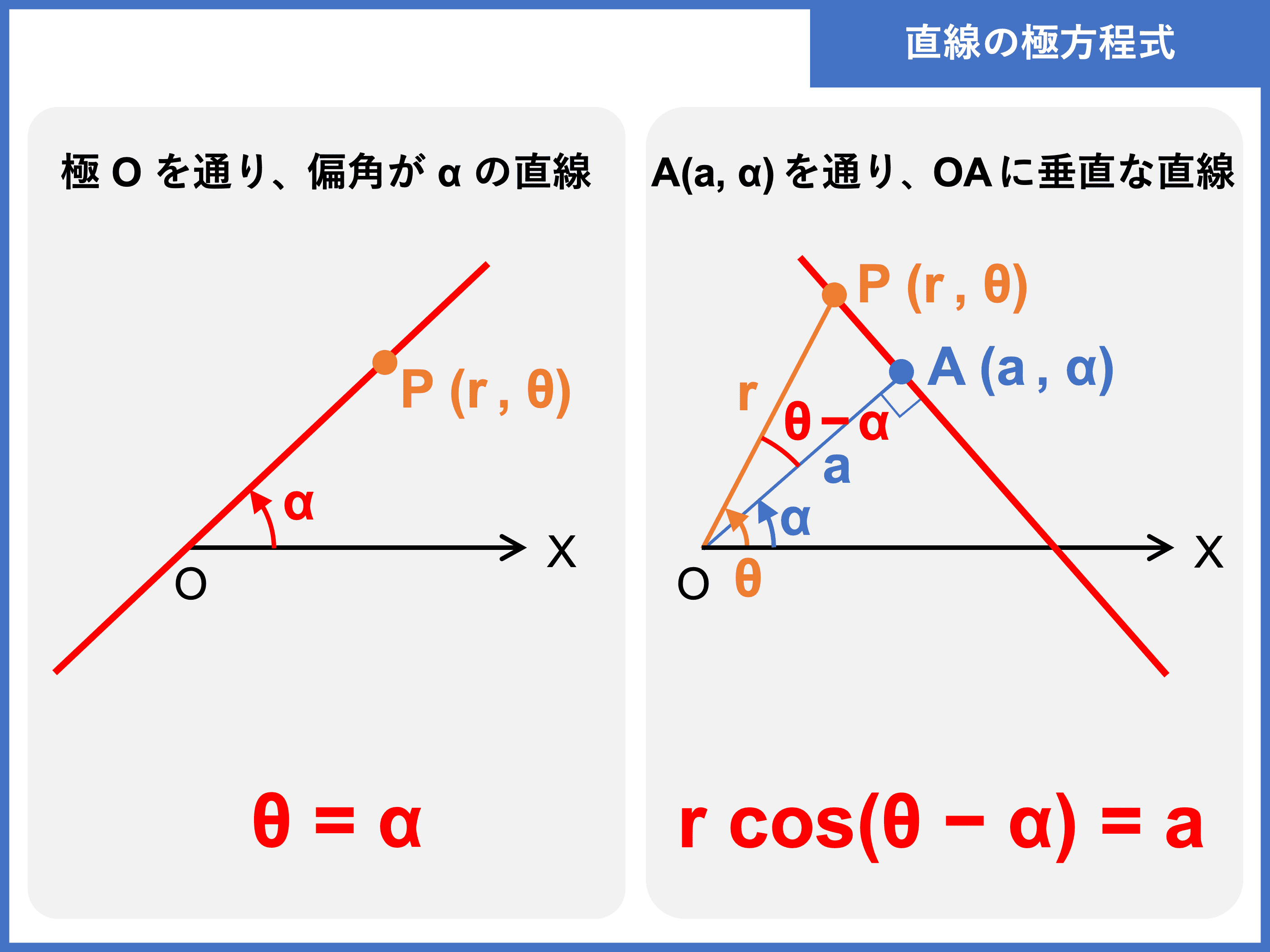
直線上の任意の点 \(\mathrm{P}(r, \theta)\) において成り立つ関係性に着目します。
極 \(\mathrm{O}\) を通り、始線となす角が \(\alpha\) の直線
→ 原点からの距離 \(r\) によらず、偏角 \(\theta = \alpha\) で一定である。
よって極方程式は、\(\color{red}{\theta = \alpha}\)
（証明終わり）
点 \(\mathrm{A}(a, \alpha)\) を通り、\(\mathrm{OA}\) に垂直な直線
→ 直角三角形 \(\mathrm{OAP}\) において、三角比を考える。
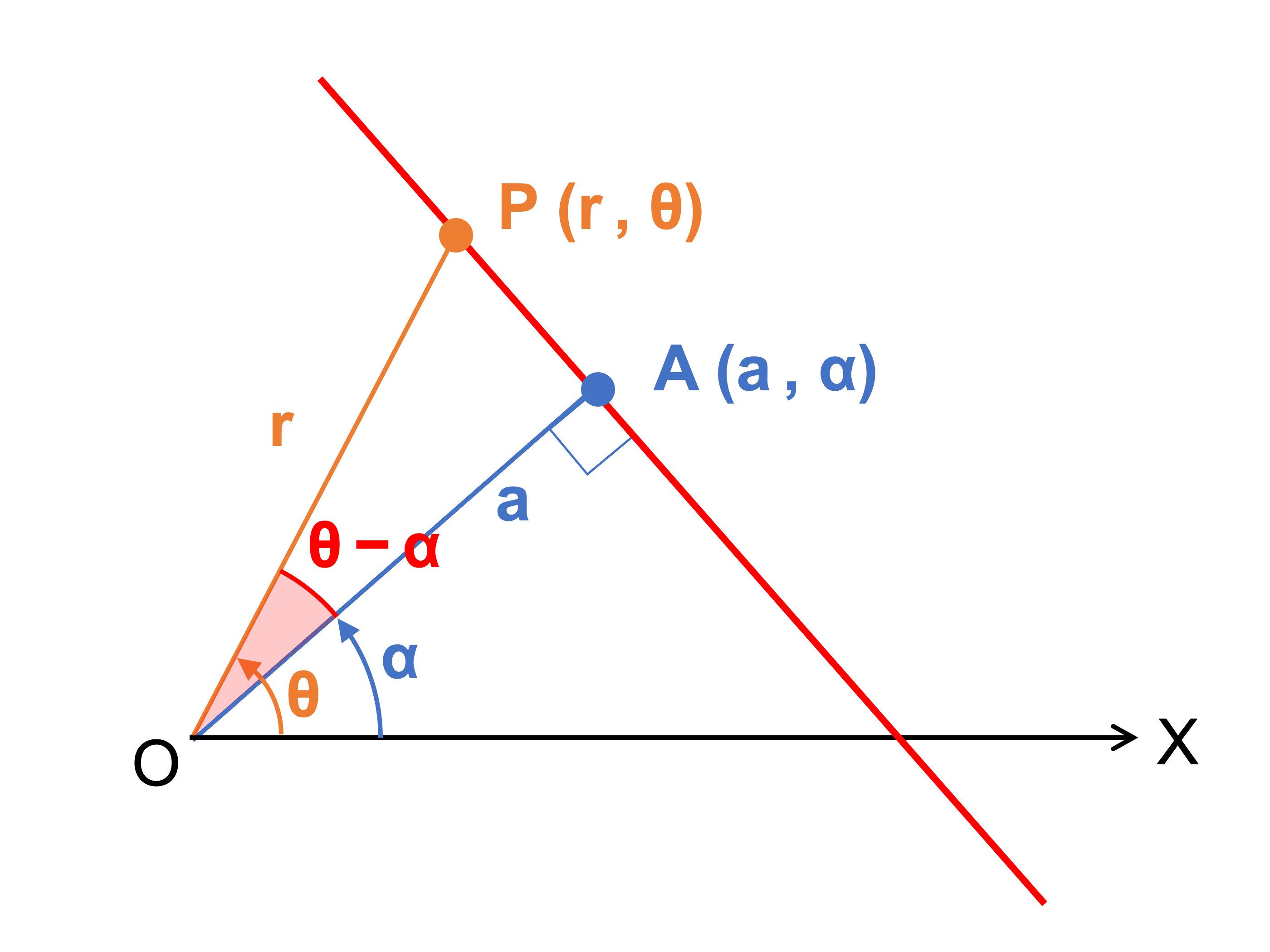
\(\triangle \mathrm{OAP}\) において、\(\angle A = 90^\circ\) より
\(\mathrm{OP} \cos \angle \mathrm{POA} = \mathrm{OA}\)
すなわち
\(\color{red}{r \cos (\theta −\alpha) = a}\)
（証明終わり）
円の極方程式
円には、次の \(3\) 通りの極方程式があります。
- 中心が極 \(\mathrm{O}\)、半径が \(a\) の円
\begin{align}\color{red}{r = a}\end{align} - 中心が \((a, 0)\)、半径が \(a\) の円
\begin{align}\color{red}{r = 2a\cos\theta}\end{align} - 中心が \((r_0, \theta_0)\)、半径が \(a\) の円
\begin{align}\color{red}{r^2 − 2rr_0 \cos(\theta − \theta_0) + r_0^2 = a^2}\end{align}
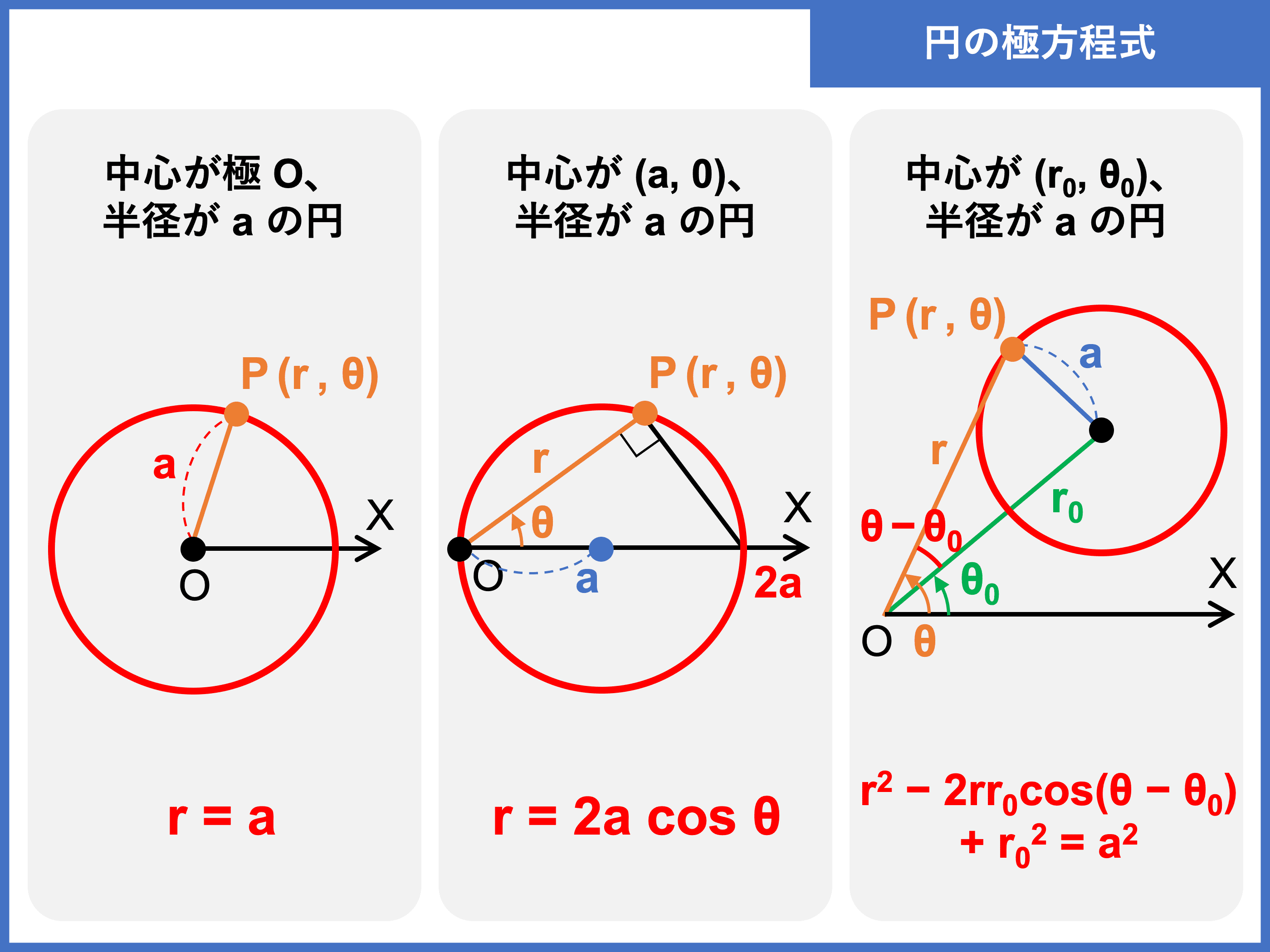
円周上の任意の点 \(\mathrm{P}(r, \theta)\) において成り立つ関係性に着目します。
中心が極 \(\mathrm{O}\)、半径が \(a\) の円
→ 偏角 \(\theta\) によらず、原点からの距離 \(r = a\) で一定である。
よって極方程式は
\(\color{red}{r = a}\)
（証明終わり）
中心が \(\mathrm{A}(a, 0)\)、半径が \(a\) の円
→ 直角三角形 \(\mathrm{OBP}\) において、三角比を考える。
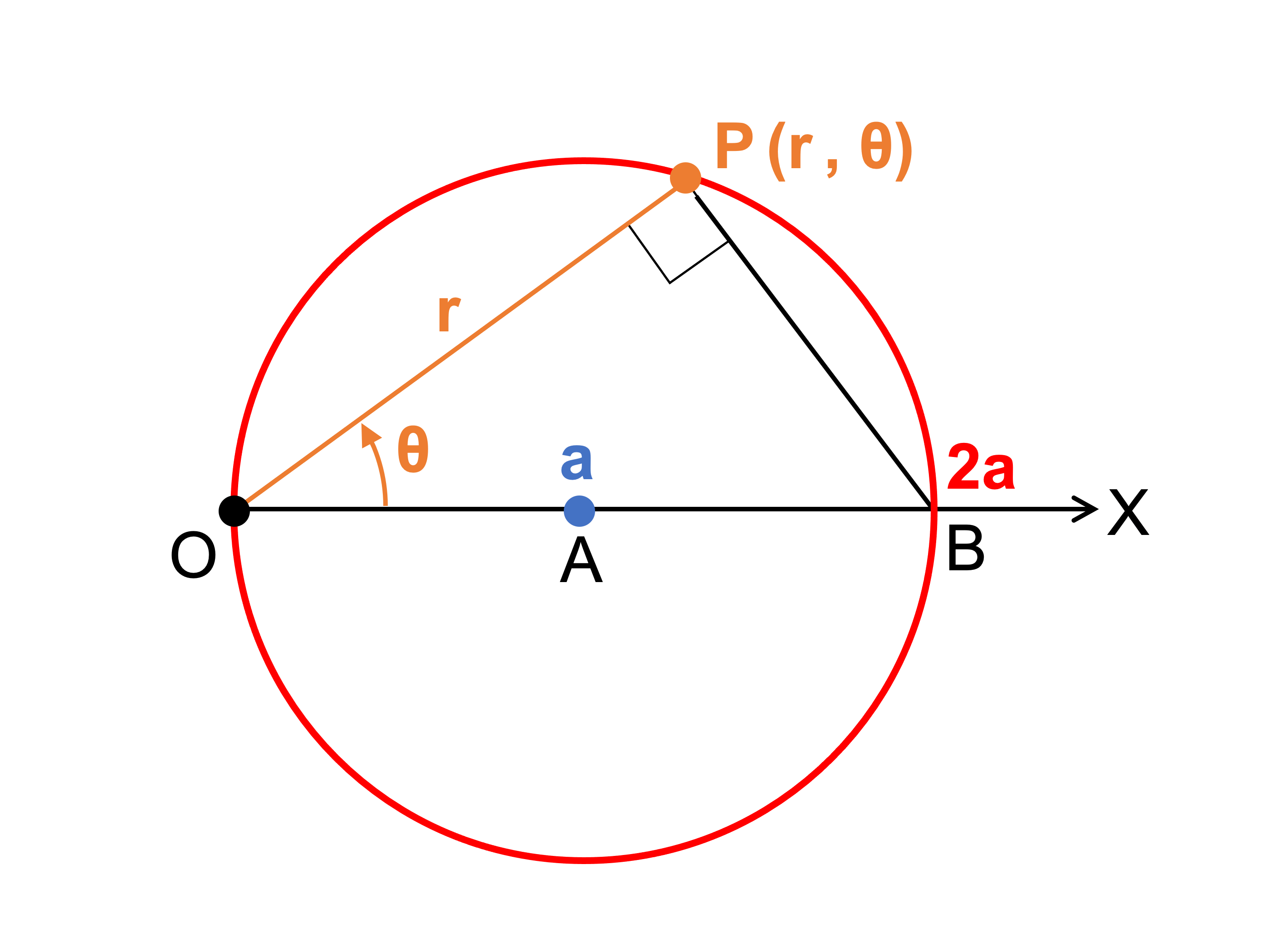
\(\triangle \mathrm{OBP}\) において、\(\angle P = 90^\circ\) より
\(\mathrm{OP} = \mathrm{OB} \cos \angle \mathrm{POB}\)
すなわち
\(\color{red}{r = 2a \cos \theta}\)
（証明終わり）
中心が \(\mathrm{C}(r_0, \theta_0)\)、半径が \(a\) の円
→ 三角形 \(\mathrm{OCP}\) において、余弦定理を適用する。
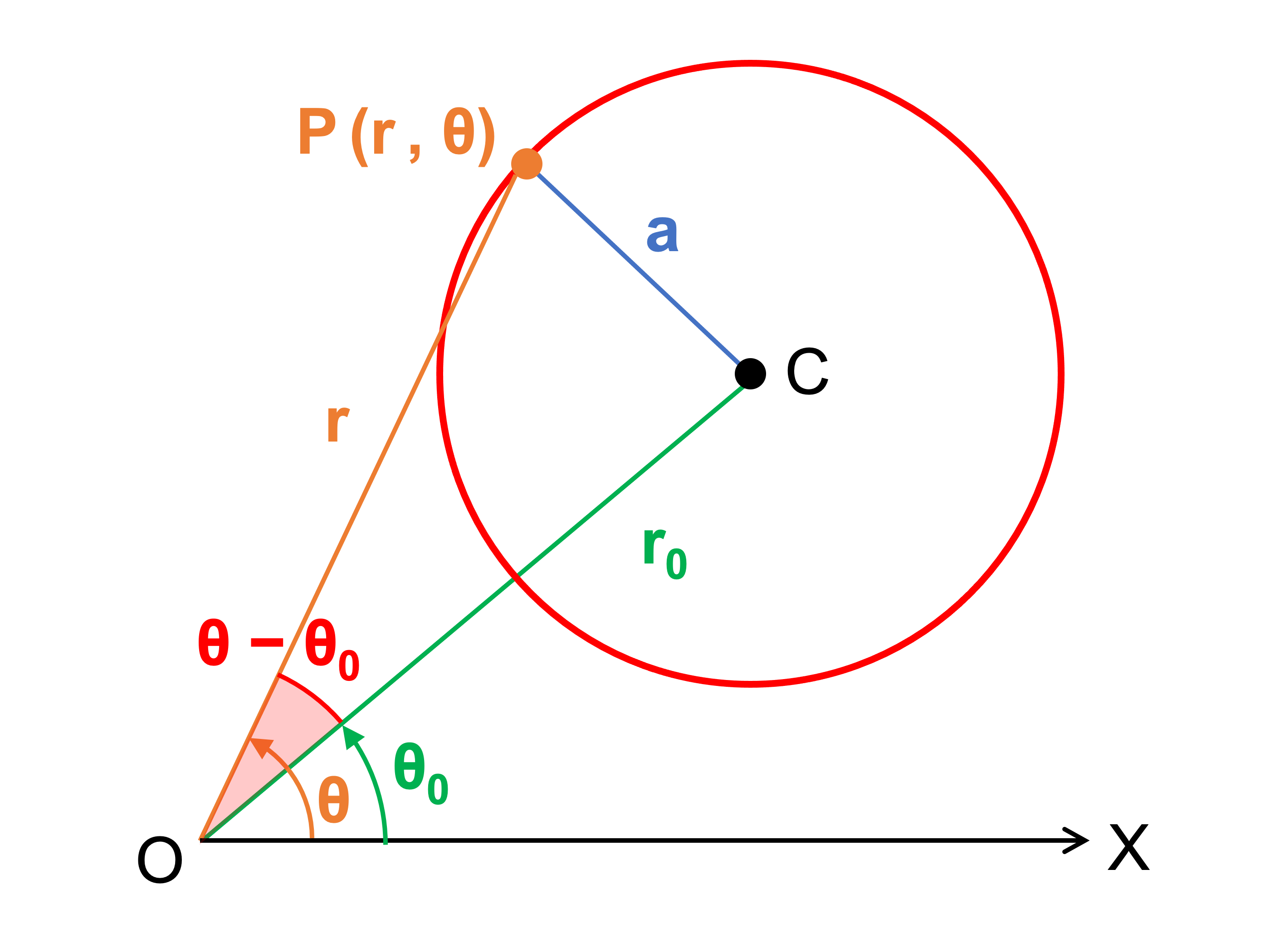
\(\triangle \mathrm{OCP}\) において、余弦定理より
\(\mathrm{CP}^2 = \mathrm{OP}^2 + \mathrm{OC}^2 − 2 \mathrm{OP} \cdot \mathrm{OC} \cos \angle \mathrm{COP}\)
\(a^2 = r^2 + r_0^2 − 2rr_0 \cos (\theta − \theta_0)\)
整理して
\(\color{red}{r^2 − 2rr_0 \cos(\theta − \theta_0) + r_0^2 = a^2}\)
（証明終わり）
二次曲線の極方程式
二次曲線（楕円・放物線・双曲線）は、共通の極方程式で表すことができます。
これは、二次曲線が「\(1\) 点（焦点 \(\mathrm{F}\)）と \(1\) 本の直線（準線 \(\ell\)）からの距離の比（離心率 \(e\)） が一定である点の軌跡」と考えられるためです。
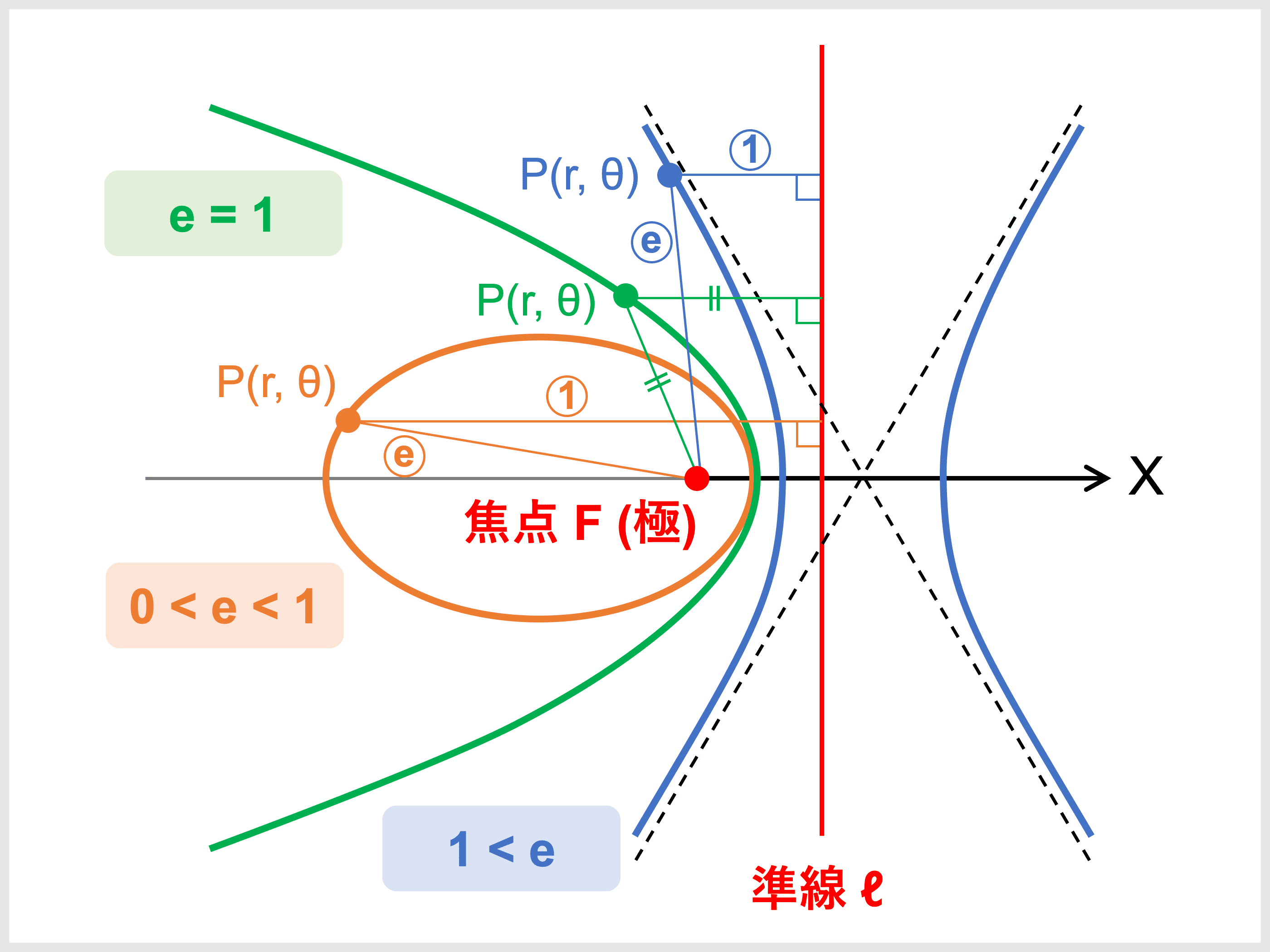
焦点 \(\mathrm{F}\) を極とする二次曲線上の点を \((r, \theta)\)、半直弦 \(l \,(> 0)\)、離心率を \(e\) とすると、その極方程式は
- \(\color{red}{\displaystyle r = \frac{l}{1 + e\cos\theta}}\) （準線 \(\ell\) が焦点 \(\mathrm{F}\) より右の場合）
- \(\color{red}{\displaystyle r = \frac{l}{1 − e\cos\theta}}\) （準線 \(\ell\) が焦点 \(\mathrm{F}\) より左の場合）
なお、
- \(0 < e < 1\) のときは 楕円
- \(e = 1\) のときは 放物線
- \(1 < e\) のときは 双曲線
を表す。
代表的な二次曲線については個別の記事で説明しています。
楕円とは？方程式やグラフ、焦点・接線・面積の求め方
双曲線とは？方程式やグラフ、焦点・漸近線・接線の求め方
また、極方程式（または媒介変数表示）で簡潔に表せる曲線はまだまだあります。
特に有名な曲線については、以下の記事で説明しています。
有名曲線のグラフ・式一覧（カージオイド・サイクロイドなど）
極方程式 ⇆ 直交座標の方程式の変換
極座標 \((r, \theta)\) と直交座標 \((x, y)\) の関係を理解できていれば、極方程式と直交座標の方程式の変換も楽勝です。
- 直交座標の方程式 → 極方程式
\(F(x, y) = 0\) に関係式 \(\color{red}{x = r\cos\theta, }\) \(\color{red}{y = r\sin\theta}\) を代入する。 - 極方程式 → 直交座標の方程式
\(F(r, \theta) = 0\) を \(r^2\), \(r\cos\theta\), \(r\sin\theta\) を含む形に変形し、
関係式 \(\color{red}{r^2 = x^2 + y^2, }\) \(\color{red}{r \cos\theta = x, }\) \(\color{red}{r \sin\theta = y}\) を代入する。
どちらも練習しておきましょう。
例題①（直交座標の方程式 → 極方程式）
次の図形の方程式を極方程式で表せ。
(1) 楕円 \(2x^2 + 3y^2 = 1\)
(2) 直線 \(x + \sqrt{3}y − 4 = 0\)
\(x = r\cos\theta\), \(y = r\sin\theta\) を代入し、方程式の \(x\), \(y\) を消去します。
極方程式がなるべく簡潔になるように、三角関数の性質を利用して式を整理しましょう。
(1)
\(2x^2 + 3y^2 = 1\) に \(x = r\cos\theta\), \(y = r\sin\theta\) を代入して
\(2r^2\cos^2\theta + 3r^2\sin^2\theta = 1\)
\(2r^2(\cos^2\theta + \sin^2\theta) + r^2\sin^2\theta = 1\)
\(2r^2 + r^2\sin^2\theta = 1\)
よって \(r^2(2 + \sin^2\theta) = 1\)
（\(r^2(3 − \cos^2\theta) = 1\) なども可）
答え： \(\color{red}{r^2(2 + \sin^2\theta) = 1}\)
(2)
\(x + \sqrt{3}y − 4 = 0\) に \(x = r\cos\theta\), \(y = r\sin\theta\) を代入して
\(r\cos\theta + \sqrt{3}r\sin\theta − 4 = 0\)
\(\displaystyle 2r \left( \frac{1}{2} \cos\theta + \frac{\sqrt{3}}{2} \sin\theta \right) − 4 = 0\)
\(\displaystyle 2r \left( \cos\theta \cos\frac{\pi}{3} + \sin\theta \sin\frac{\pi}{3} \right) − 4 = 0\)
\(\displaystyle 2r\cos\left( \theta − \frac{\pi}{3} \right) − 4 = 0\)
\(\displaystyle 2r\cos\left( \theta − \frac{\pi}{3} \right) = 4\)
よって \(\displaystyle r\cos\left( \theta − \frac{\pi}{3} \right) = 2\)
（\(\displaystyle r\sin\left( \theta + \frac{\pi}{6} \right) = 2\) なども可）
答え： \(\color{red}{\displaystyle r\cos\left( \theta − \frac{\pi}{3} \right) = 2}\)
使用する三角関数の性質や加法定理の種類によって、複数の解答があり得ます。
例題②（極方程式 → 直交座標の方程式）
次の極方程式はどのような曲線を示すか。直交座標の方程式で答えよ。
(1) \(r = \sqrt{3}\cos\theta + \sin\theta\)
(2) \(r^2(1 + 3\cos^2\theta) = 4\)
式が \(r^2\), \(r\cos\theta\), \(r\sin\theta\) を含む状態にしてあげると、\(x\), \(y\) にスムーズに変換できます。
必要に応じて両辺に何かをかけたり、式を部分的に展開したりと工夫しましょう。
(1)
両辺に \(r\) をかけて
\(r^2 = \sqrt{3}r\cos\theta + r\sin\theta\)
\(r^2 = x^2 + y^2\), \(r\cos\theta = x\), \(r\sin\theta = y\) より
\(x^2 + y^2 = \sqrt{3}x + y\)
\(x^2 − \sqrt{3}x + y^2 − y = 0\)
\(\displaystyle \left( x − \frac{\sqrt{3}}{2} \right)^2 − \frac{3}{4} + \left( y − \frac{1}{2} \right)^2 − \frac{1}{4} = 0\)
よって 円 \(\displaystyle \left( x − \frac{\sqrt{3}}{2} \right)^2 + \left( y − \frac{1}{2} \right)^2 = 1\)
答え： 円 \(\color{red}{\displaystyle \left( x − \frac{\sqrt{3}}{2} \right)^2 + \left( y − \frac{1}{2} \right)^2 = 1}\)
(2)
\(r^2(1 + 3\cos^2\theta) = 4\) より
\(r^2 + 3(r\cos\theta)^2 = 4\)
\(r^2 = x^2 + y^2\), \(r\cos\theta = x\) を代入して
\(x^2 + y^2 + 3x^2 = 4\)
\(4x^2 + y^2 = 4\)
よって 楕円 \(\displaystyle \frac{x^2}{4} + y^2 = 1\)
答え： 楕円 \(\color{red}{\displaystyle \frac{x^2}{4} + y^2 = 1}\)
極方程式のグラフの書き方
ここでは、極方程式のグラフの概形を書く方法を説明します。
基本的には、具体的な角 \(\theta\) の値を代入して曲線上の極座標 \((r, \theta)\) の組をいくつか求めていけば、グラフの概形が得られます。
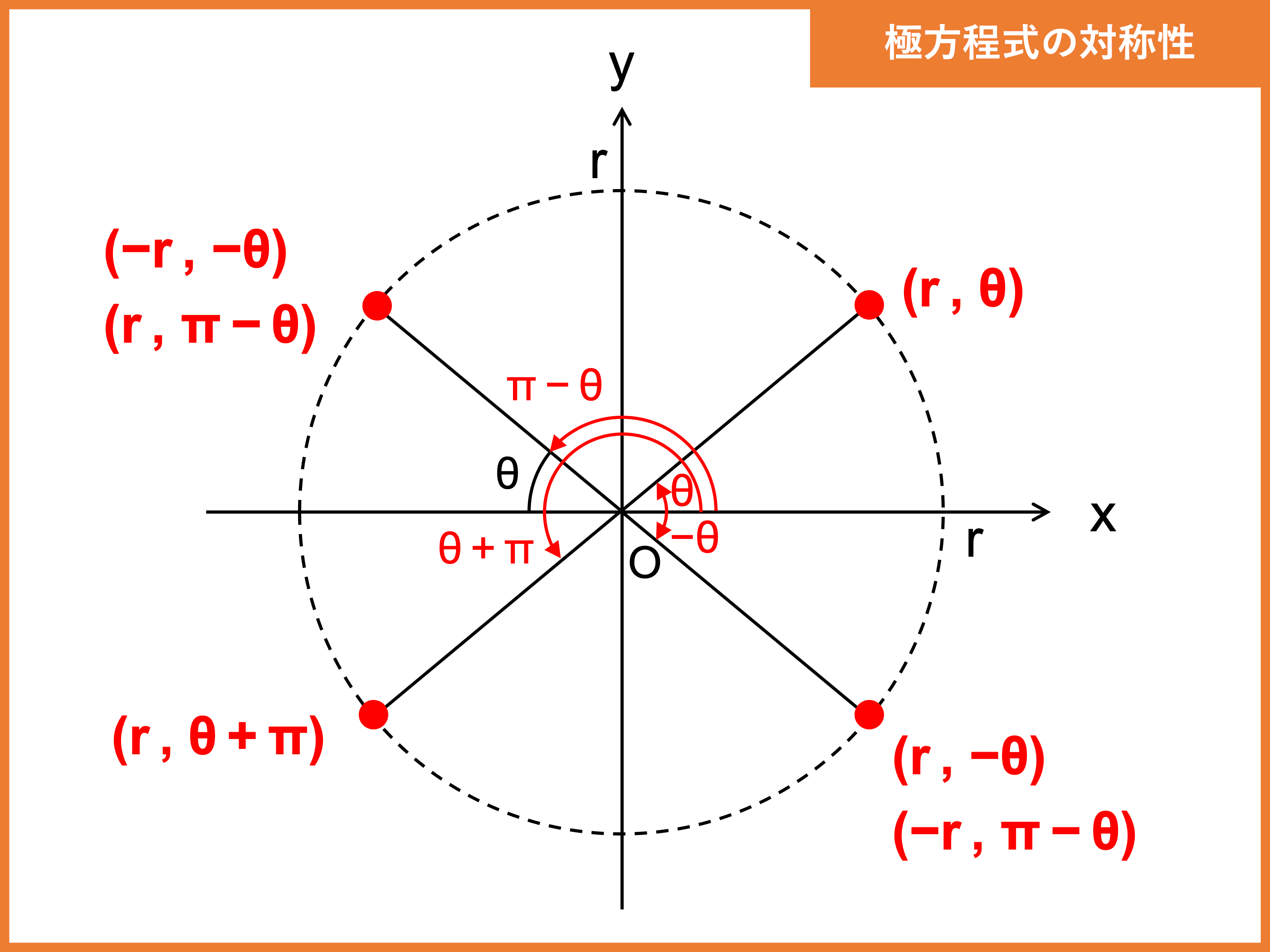
その際、極方程式の対称性に注目すると、極座標を求める労力、グラフを図示する労力を最小限に抑えられます。
- 始線に関して対称（\(x\) 軸対称）
\(f(−\theta) = f(\theta)\) または \(f(\pi − \theta) = − f(\theta)\) - 極を通り、視線に垂直な直線に関して対称（\(y\) 軸対称）
\(f(−\theta) = −f(\theta)\) または \(f(\pi − \theta) = f(\theta)\) - 極に関して対称（原点対称）
\(f(\theta + \pi) = f(\theta)\)
それでは、例題を通してグラフの書き方を確認しましょう。
次の極方程式で表される曲線の概形を書け。
\(r^2 = \cos2\theta\)
\(f(\theta) = \cos2\theta\) とおくと、
\(\begin{align} f(\theta + \pi) &= \cos(2\theta + 2\pi) \\ &= \cos2\theta \\ &= f(\theta) \end{align}\)
よって \(r^2 = f(\theta)\) は極に関して対称
\(\begin{align} f(−\theta) &= \cos2(−\theta) \\ &= \cos2\theta \\ &= f(\theta) \end{align}\)
よって \(r^2 = f(\theta)\) は始線に関して対称
\(\begin{align} f(\pi − \theta) &= \cos(2\pi − 2\theta) \\ &= \cos(− 2\theta) \\ &= \cos2\theta \\ &= f(\theta) \end{align}\)
よって \(r^2 = f(\theta)\) は極を通り始線に垂直な直線に関して対称
以上より、\(\displaystyle 0 \leq \theta \leq \frac{\pi}{2}\) で考える。
また、\(r^2 \geq 0\) より、\(\cos2\theta \geq 0\)
このとき、\(\displaystyle 0 \leq 2\theta \leq \frac{\pi}{2}\) であるから
さらに \(\displaystyle 0 \leq \theta \leq \frac{\pi}{4}\) で考える。
上記の範囲で \(r^2 = \cos2\theta\) 上のいくつかの点を調べると、
| \(\theta\) | \(0\) | \(\displaystyle \frac{\pi}{12}\) | \(\displaystyle \frac{\pi}{8}\) | \(\displaystyle \frac{\pi}{6}\) | \(\displaystyle \frac{\pi}{4}\) |
|---|---|---|---|---|---|
| \(r^2\) | \(1\) | \(\displaystyle \frac{\sqrt{3}}{2}\) | \(\displaystyle \frac{1}{\sqrt{2}}\) | \(\displaystyle \frac{1}{2}\) | \(0\) |
| \(r\) | \(1\) | \(\displaystyle \frac{\sqrt[4]{3}}{\sqrt{2}}\) | \(\displaystyle \frac{1}{\sqrt[4]{2}}\) | \(\displaystyle \frac{1}{\sqrt{2}}\) | \(0\) |
対称性を考慮すると、曲線 \(r^2 = \cos2\theta\) の概形は以下の通り。
答え：
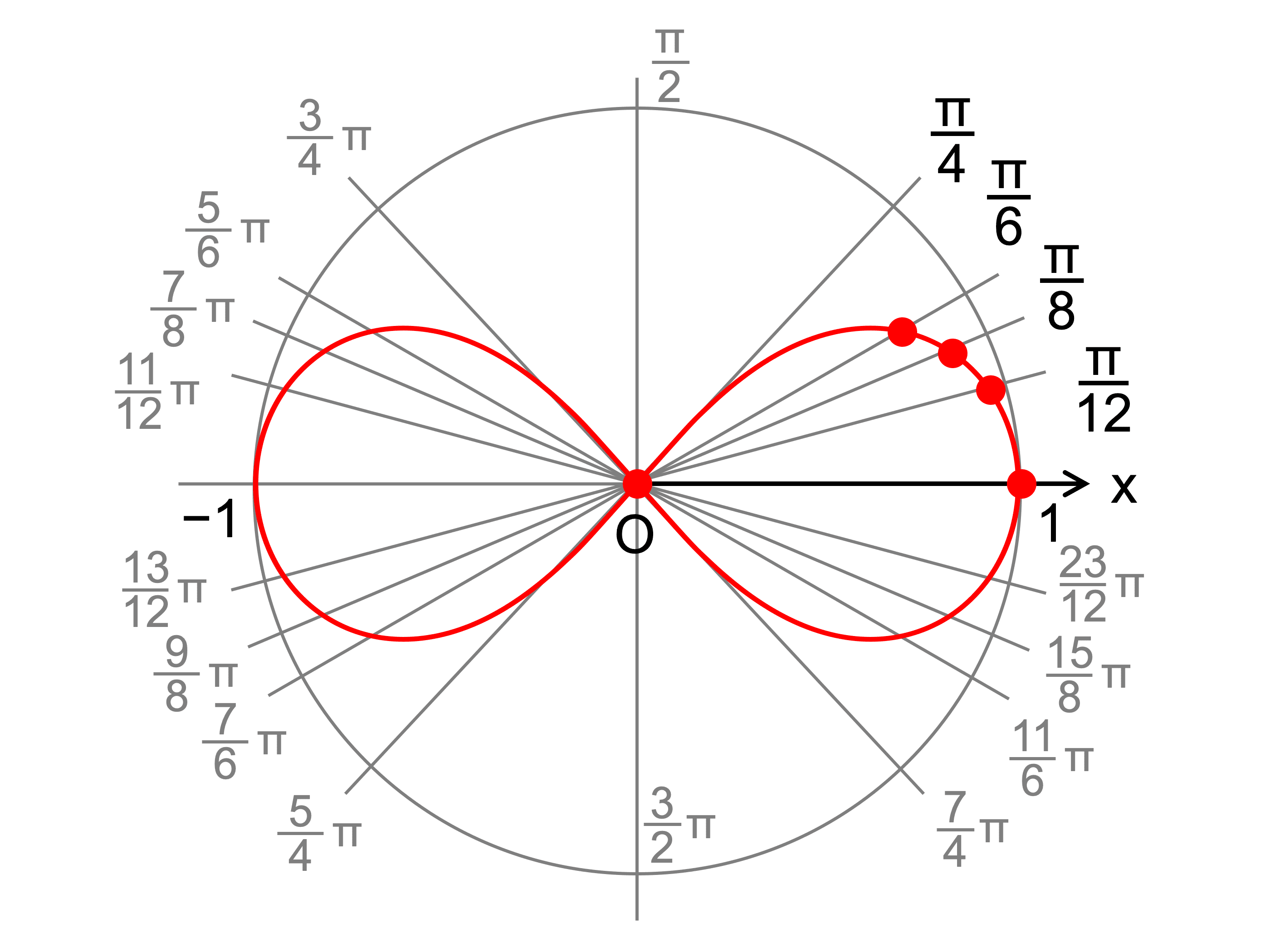
ちなみに、上記のように極方程式 \(r^2 = a^2\cos2\theta\) で表される曲線をレムニスケート（連珠形）といいます。
レムニスケートを含む有名な曲線については、以下の記事で説明しています。
有名曲線のグラフ・式一覧（カージオイド・サイクロイドなど）
極方程式の発展公式
ここでは、極方程式の発展的な公式を紹介します。
高校では扱わないことが多いので、参考までに眺めてくださいね。
極方程式の面積
極方程式 \(r = f(\theta) \) \((\alpha \leq \theta \leq \beta)\) で表される曲線上の点と極 \(\mathrm{O}\) を結んだ線分が通過する領域の面積 \(S\) は
\begin{align}\color{red}{\displaystyle S = \frac{1}{2} \int_\alpha^\beta r^2 d\theta}\end{align}
上記の公式は、偏角 \(\theta\) の微小な変化 \(\Delta\theta\) に対する微小な面積変化 \(\Delta S\) を扇型の面積とみなして積分すると導くことができます。
極方程式の曲線の長さ
極方程式 \(r = f(\theta) \) \((\alpha \leq \theta \leq \beta)\) で表される曲線の長さ \(L\) は
\begin{align}\color{red}{\displaystyle L = \int_\alpha^\beta \sqrt{\left( \frac{dr}{d\theta} \right)^2 + r^2} \, d\theta}\end{align}
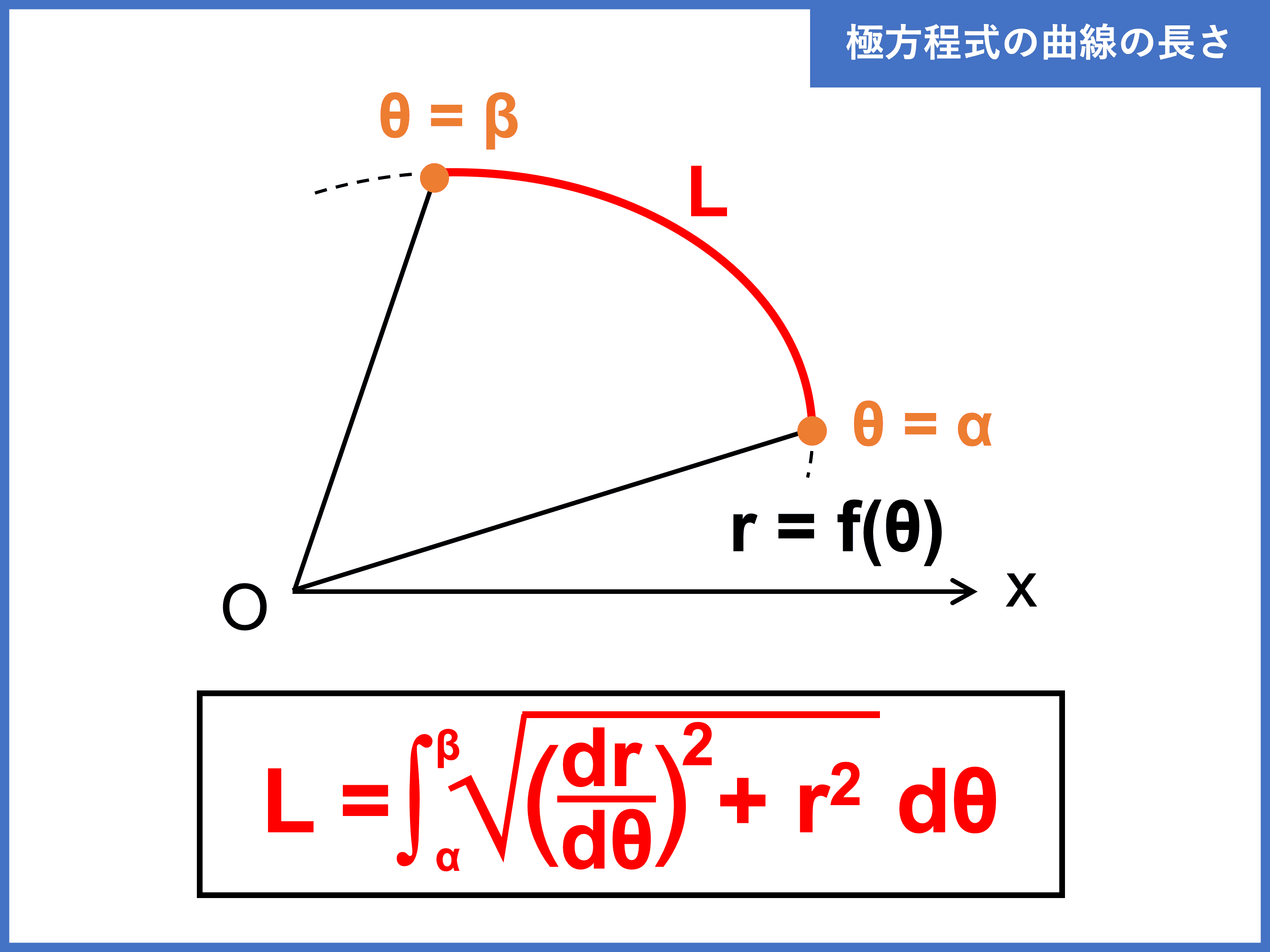
上記の公式は、陽関数表示または媒介変数表示の曲線の長さの公式を地道に式変形していけば得られます。
「曲線の長さ」については以下の記事を確認してください。
曲線の長さの求め方を解説！積分公式と証明、問題の解き方
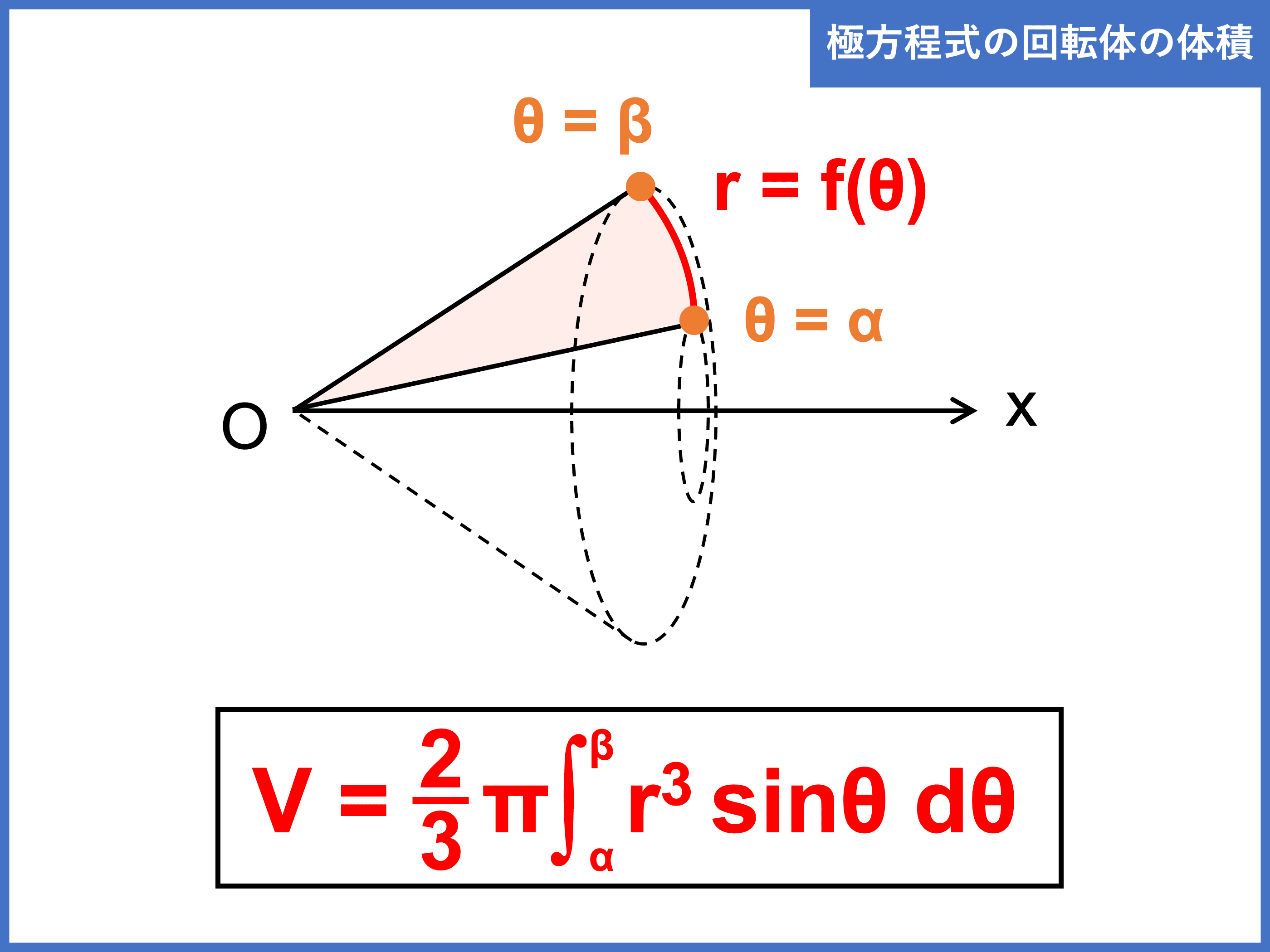
極方程式の回転体の体積
\(r = f(\theta)\), \(\theta = \alpha\), \(\theta = \beta\) に囲まれた図形を始線（\(x\) 軸）のまわりに一回転させてできる立体の体積 \(V\) は
\begin{align}\color{red}{\displaystyle V = \frac{2}{3}\pi \int_\alpha^\beta r^3 \sin\theta \, d\theta}\end{align}
以上で解説は終わりです。
極方程式があることで、表現できる曲線の種類がグッと広がります。
極方程式への理解を深めて、ぜひマスターしてくださいね！
質問です
極方程式の曲線の概形の例題では
f(-θ)=f(θ)で始線について対称と書いていますが、
対称性の公式では
f(-θ)=f(θ)
のとき始線の垂直な線について対称と書いています。
どちらが正しいのですか？
すみません
勘違いしてました